A particle is projected upwards with a velocity of 100m/s at an angle of 60∘ with the vertical. Find the time when the particle will move perpendicular to its initial direction, taking g=10m/s2.
The correct Answer is:20 s
To solve the problem step by step, we will analyze the motion of the particle projected at an angle with respect to the vertical.
Step 1: Understand the Components of Velocity
The initial velocity u of the particle is given as 100m/s at an angle of 60∘ with the vertical. We need to find the horizontal and vertical components of this velocity.
- The horizontal component ux (along the x-axis) is given by:
ux=usin(60∘)=100sin(60∘)=100⋅√32=50√3m/s
- The vertical component uy (along the y-axis) is given by:
uy=ucos(60∘)=100cos(60∘)=100⋅12=50m/s
Step 2: Determine the Condition for Perpendicular Motion
The problem states that we need to find the time when the particle's motion becomes perpendicular to its initial direction. For two vectors to be perpendicular, their dot product must equal zero.
Let vx and vy be the horizontal and vertical components of the velocity at time t.
Step 3: Express the Components of Velocity
Since there is no acceleration in the x-direction, the horizontal velocity remains constant:
vx=ux=50√3m/s
For the vertical component, the velocity changes due to gravity:
vy=uy−gt=50−10t
Step 4: Set Up the Dot Product Condition
The dot product of the initial velocity vector u=(ux,uy) and the final velocity vector v=(vx,vy) must be zero:
uxvx+uyvy=0
Substituting the values:
(50√3)(50√3)+(50)(50−10t)=0
Step 5: Simplify the Equation
Calculating the first term:
(50√3)2=2500⋅3=7500
So, the equation becomes:
7500+2500−500t=0
10000−500t=0
Step 6: Solve for Time t
Rearranging the equation gives:
500t=10000
t=10000500=20s
Final Answer
The time when the particle will move perpendicular to its initial direction is t=20s.
---
The initial velocity u of the particle is given as 100m/s at an angle of 60∘ with the vertical. We need to find the horizontal and vertical components of this velocity.
ux=usin(60∘)=100sin(60∘)=100⋅√32=50√3m/s
uy=ucos(60∘)=100cos(60∘)=100⋅12=50m/s
The problem states that we need to find the time when the particle's motion becomes perpendicular to its initial direction. For two vectors to be perpendicular, their dot product must equal zero.
Since there is no acceleration in the x-direction, the horizontal velocity remains constant:
vx=ux=50√3m/s
vy=uy−gt=50−10t
The dot product of the initial velocity vector u=(ux,uy) and the final velocity vector v=(vx,vy) must be zero:
uxvx+uyvy=0
(50√3)(50√3)+(50)(50−10t)=0
Calculating the first term:
(50√3)2=2500⋅3=7500
So, the equation becomes:
7500+2500−500t=0
10000−500t=0
Rearranging the equation gives:
500t=10000
t=10000500=20s
The time when the particle will move perpendicular to its initial direction is t=20s.
Topper's Solved these Questions
- KINEMATICS-2DBook:ALLENChapter:KINEMATICS-2DExercise:Exercise (S-2)
Explore 15 Videos
- KINEMATICS-2DBook:ALLENChapter:KINEMATICS-2DExercise:Exercise (O-1)
Explore 40 Videos
- KINEMATICS-2DBook:ALLENChapter:KINEMATICS-2DExercise:Exercise (O-2)
Explore 46 Videos
- GRAVITATIONBook:ALLENChapter:GRAVITATIONExercise:EXERCISE 4
Explore 9 Videos
- MOTION IN A PALNEBook:ALLENChapter:MOTION IN A PALNEExercise:SOLVED EXAMPLE
Explore 28 Videos
Similar Questions
A particle is projected with a velocity 10 m//s at an angle 37∘ to the horizontal. Find the location at which the particle is at a height 1 m from point of projection.
View SolutionA particle is projected with velocity of 10ms at an angle of 15° with horizontal.The horizontal range will be(g=10ms2)
View SolutionA particle is projected with speed 10m/s at angle 60∘ with the horizontal. Then the time after which its speed becomes half of initial.
View SolutionA particle A is projected with an initial velocity of 60m/s at an angle 30∘ to the horizontal. At the same time a second particle B is projected in opposite direction with initial speed of 50m/s from a point at a distance of 100 m from A. If the particles collide in air, find (a)the angle of projection α of particle B, (b) time when the collision takes place and (c) the distance of P from A, where collision occurs. (g=10m/s2)
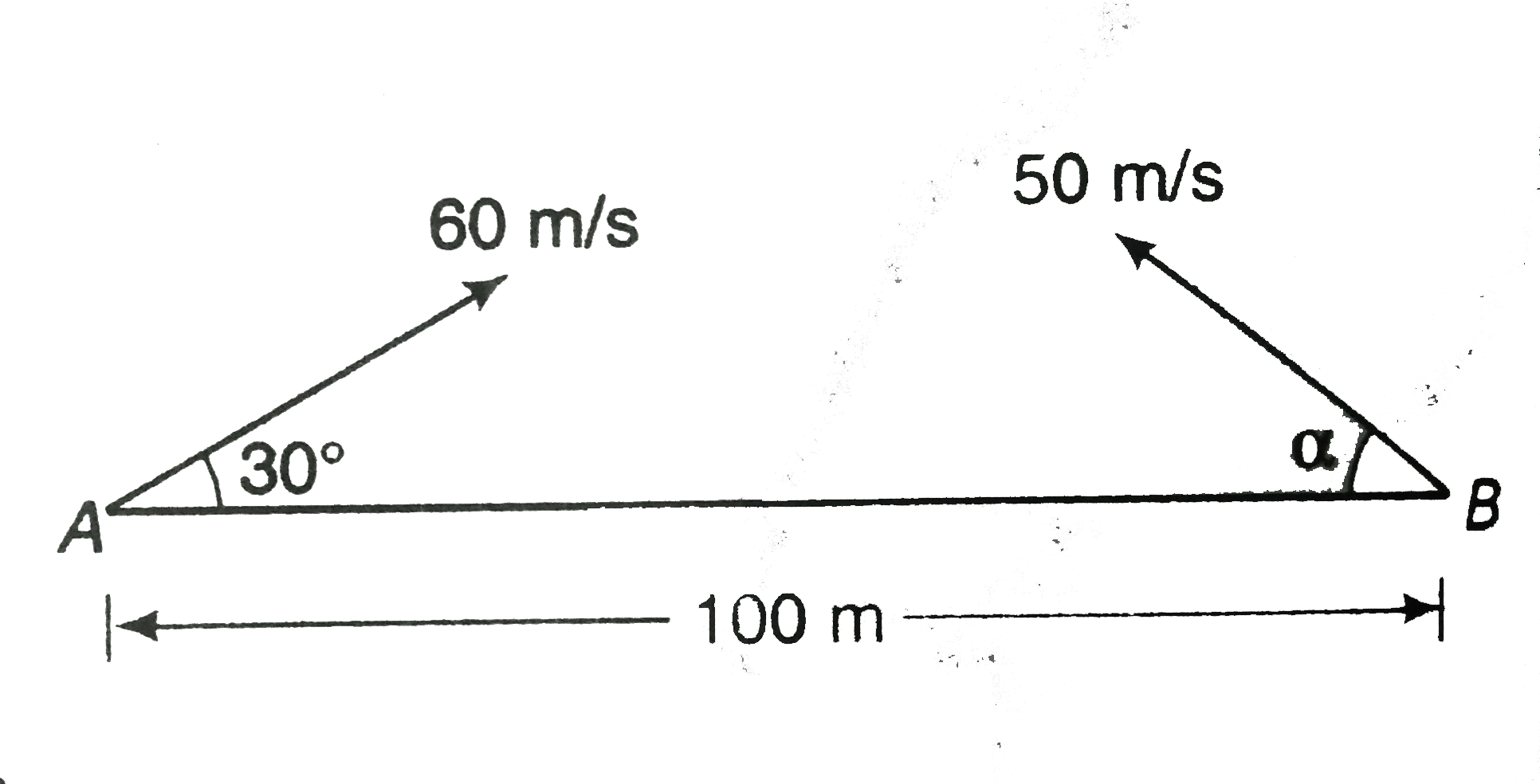
View SolutionA particle A is projected from the ground with an initial velocity of 10m/s at an angle of 60∘ with horizontal. From what height should an another particle B be projected horizontally with velocity 5m/s so that both the particles collide in ground at point C if both are projected simultaneously g=10m/s2.

View SolutionA particle is projected from the bottom of an inclined plane of inclination 30∘ with velocity of 40m/s at an angle of 60∘ with horizontal. Find the speed of the particle when its velocity vector is parallel to the plane. Take g=10m/s2.
View SolutionA particle is projected with velocity u at angle θ with horizontal. Find the time when velocity vector is perpendicular to initial velocity vector.
View SolutionA particle is projected with velocity u at angle θ with horizontal. Find the time when velocity vector is perpendicular to initial velocity vector.
View SolutionA particle is projected with velocity 50 m/s at an angle 60∘ with the horizontal from the ground. The time after which its velocity will make an angle 45∘ with the horizontal is
View SolutionA body is projected at 60∘ with the horizontal with the velocity of 10√3ms−1. Find the velocity of the projectile when it moves perpendicular to its initial direction.
View Solution