Given that P=Q=R If →P+→Q=→R then the angle between →P & →R is θ1. If →P+→Q+→R=→0 then the angle between →P & →R is θ2. What is the relation between θ1 and θ2 ?
A
θ1=θ2
B
θ1=θ22
C
θ1=2θ2
D
None of the above
Video Solution
Text Solution
The correct Answer is:2
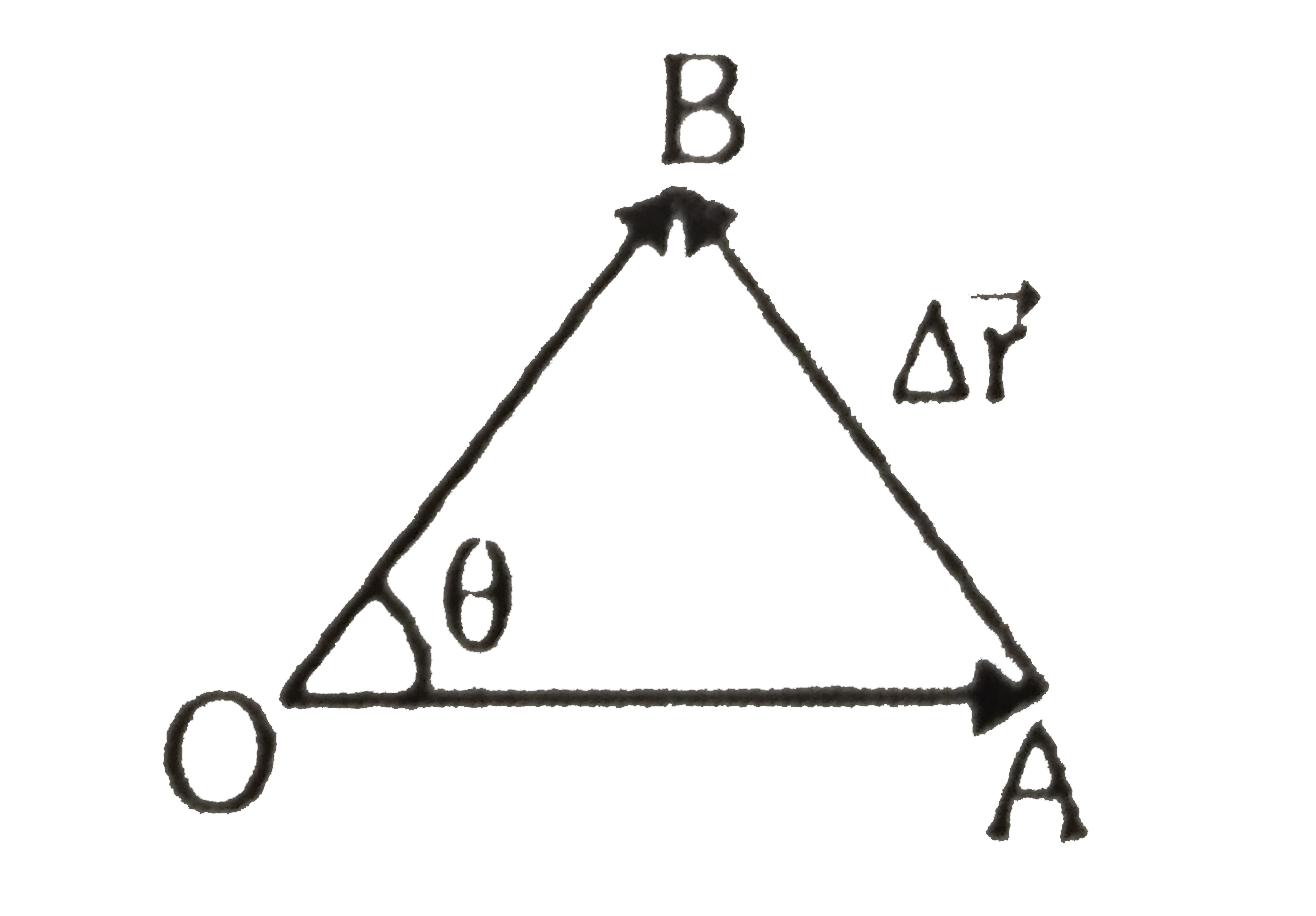
−→Δr=−−→OB−−−→OA
∣∣∣−−→OA∣∣∣=∣∣∣−−→OB∣∣∣=l
∣∣∣−→Δr∣∣∣=√l2+l2−2l2cosθ
=√2l2(1−cosθ)=√2l2×2sin2θ/2 ltBrgt =2lsinθ/2
|
Topper's Solved these Questions
- BASIC MATHEMATICS USED IN PHYSICS &VECTORS BOOK - ALLENCHAPTER - BASIC MATHEMATICS USED IN PHYSICS &VECTORS EXERCISE - EXERCISE-IV ASSERTION & REASON
11 Videos
- BASIC MATHEMATICS USED IN PHYSICS &VECTORS BOOK - ALLENCHAPTER - BASIC MATHEMATICS USED IN PHYSICS &VECTORS EXERCISE - EXERCISE-II AIPMT/NEET & AIIMS (2006- 2018)
6 Videos
- CENTRE OF MASSBOOK - ALLENCHAPTER - CENTRE OF MASSEXERCISE - EXERCISE-V B
19 Videos
Similar Questions
Knowledge Check
If θ is the angle between two vectors →a and →b, then →a.→b≥0 only when
A0<θ<π2B0≤θ≤π2C0<θ<πD0≤θ≤πIf θ is the angle between two vectors →aand→b, then →a.→b≥0 only when
A0<θ<π2B0≤θ≤π2C0<θ<πD0≤θ≤π
If →P.→Q=PQ, then angle between →P and →Q is
View SolutionWhat is the angle between →P and the resultant of (→P+→Q) and (→P−→Q) ?
View SolutionWhat can be the angle between (→P+→Q) and (→P−→Q) ?
View SolutionThe resultant →Pand→Q is perpendicular to →P. What is the angle between →Pand→Q?
View SolutionWhat is the angle between (→P+→Q) and (→P×→Q)?
View SolutionIf →P×→Q=→R, then which of the following statements is not true?
View Solution
