Enthalpy of 1 mole monoatomic ideal gas is equals to :-
32RT
52RT
RT
2 RT
The correct Answer is:B
To determine the enthalpy of 1 mole of a monoatomic ideal gas, we can follow these steps:
1. Understand the Formula for Enthalpy:
The enthalpy change (ΔH) for a gas can be expressed as:
ΔH=nCpΔT
where:
- n = number of moles of the gas,
- Cp = molar heat capacity at constant pressure,
- ΔT = change in temperature.
2. Identify the Molar Heat Capacity for Monoatomic Gas:
For a monoatomic ideal gas, the molar heat capacity at constant pressure (Cp) is given by:
Cp=52R
where R is the universal gas constant.
3. Substitute the Values:
Since we are considering 1 mole of the gas (n=1), we can substitute the values into the enthalpy change formula:
ΔH=1⋅52RΔT
This simplifies to:
ΔH=52RΔT
4. Integrate to Find Total Enthalpy:
To find the total enthalpy (H), we can integrate with respect to temperature:
H=52RT
Here, T is the absolute temperature of the gas.
5. Conclusion:
Therefore, the enthalpy of 1 mole of a monoatomic ideal gas is:
H=52RT
Final Answer:
The enthalpy of 1 mole of a monoatomic ideal gas is 52RT.
The enthalpy change (ΔH) for a gas can be expressed as:
ΔH=nCpΔT
where:
- n = number of moles of the gas,
- Cp = molar heat capacity at constant pressure,
- ΔT = change in temperature.
For a monoatomic ideal gas, the molar heat capacity at constant pressure (Cp) is given by:
Cp=52R
where R is the universal gas constant.
Since we are considering 1 mole of the gas (n=1), we can substitute the values into the enthalpy change formula:
ΔH=1⋅52RΔT
This simplifies to:
ΔH=52RΔT
To find the total enthalpy (H), we can integrate with respect to temperature:
H=52RT
Here, T is the absolute temperature of the gas.
Therefore, the enthalpy of 1 mole of a monoatomic ideal gas is:
H=52RT
The enthalpy of 1 mole of a monoatomic ideal gas is 52RT.
Topper's Solved these Questions
- THERMODYNAMICS BOOK - ALLENCHAPTER - THERMODYNAMICS EXERCISE - EXERCISE -3
108 Videos
- THERMODYNAMICS BOOK - ALLENCHAPTER - THERMODYNAMICS EXERCISE - EXERCISE -4
18 Videos
- THERMODYNAMICS BOOK - ALLENCHAPTER - THERMODYNAMICS EXERCISE - EXERCISE -1B
22 Videos
- TEST PAPERSBOOK - ALLENCHAPTER - TEST PAPERSEXERCISE - MATHEMATICS
2 Videos
- Thermodynamics And Thermo ChemistryBOOK - ALLENCHAPTER - Thermodynamics And Thermo ChemistryEXERCISE - All Questions
40 Videos
Similar Questions
Knowledge Check
A thermodynamic process of one mole ideal monoatomic gas is shown in figure. The efficiency of cyclic process ABCA will be
A0.25B0.125C0.5D0.077
The ratio of P to V at any instant is constant and is equal to 1. for a monoatomic ideal gas under going a process. What is the molar heat capacity of the gas
View SolutionThe ratio of P to V at any instant is constant and is equal to 1, for a monoatomic ideal gas under going a process. What is the molar heat capacity of the gas
View SolutionThe internal energy of one mole of ideal gas is
View SolutionTwo moles of a monoatomic ideal gas undergoes a process AB as shown in the figure. Then, find the work done during the process in Joule.
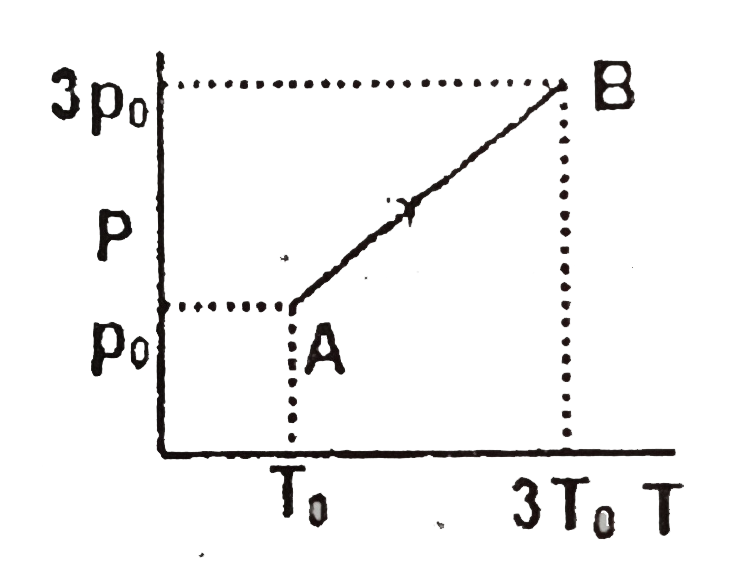
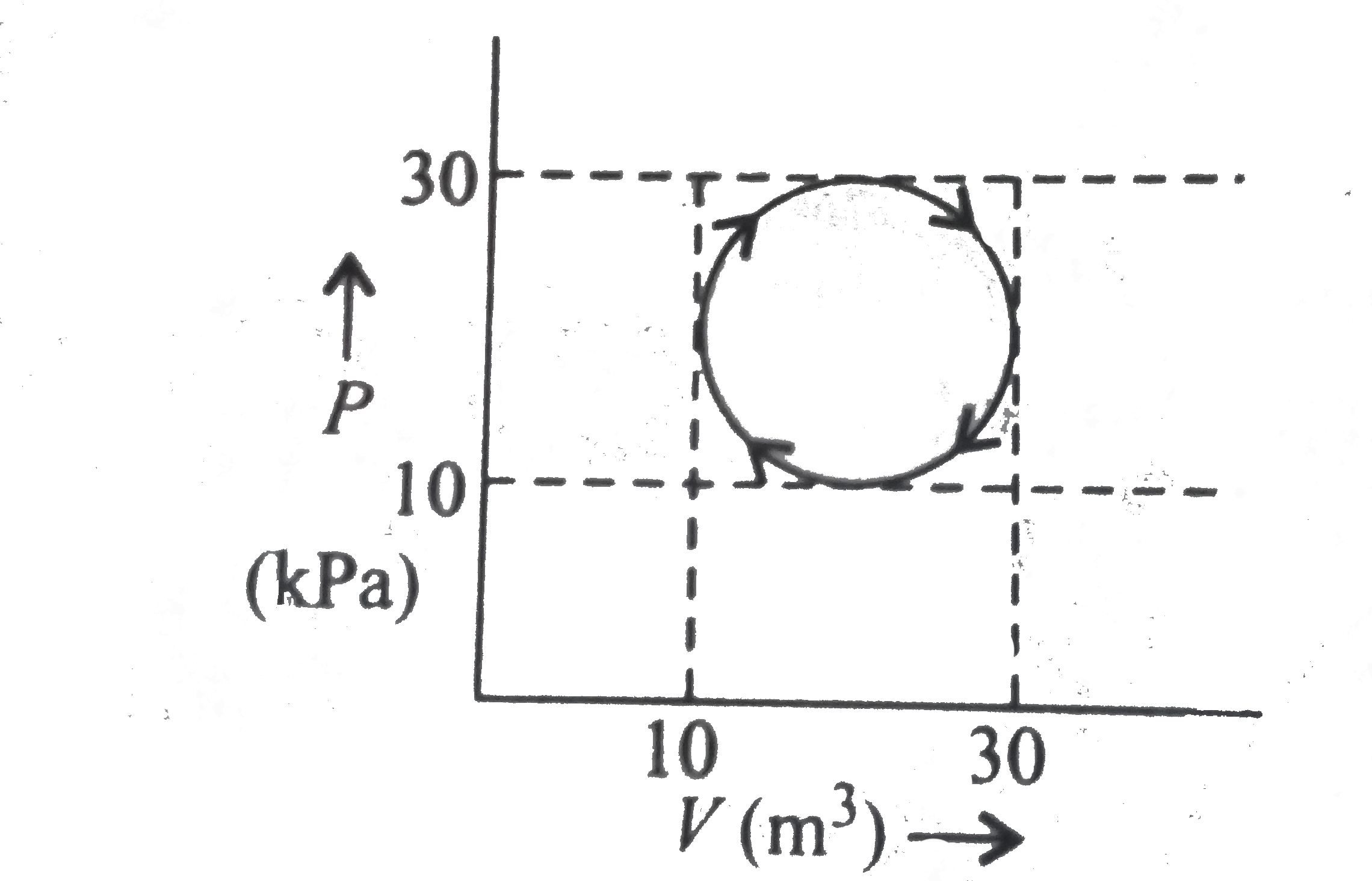
View SolutionWhich one is not correct for a cyclic process as shown in the figure ?
View SolutionA thermodynamic process of one mole ideal monoatomic gas is shown in figure. The efficiency of cyclic process ABCA will be

View SolutionOne mole of an ideal monoatomic gas is caused to go through the cycle shown in figure. Then the change in the internal energy of gas from a to b and b to c is respectively
View Solution
