Three identical particles force exerted on one body due to the other two.
The correct Answer is:√3Gm2a2
To solve the problem of finding the net force exerted on one body due to the other two identical particles placed at the corners of an equilateral triangle, we can follow these steps:
1. Identify the Setup:
We have three identical particles, each of mass m, placed at the corners of an equilateral triangle with side length a.
2. Determine the Gravitational Force Between Two Particles:
The gravitational force F between any two particles is given by Newton's law of gravitation:
F=Gm2a2
where G is the gravitational constant.
3. Analyze Forces Acting on One Particle:
Let's focus on one particle (let's call it Particle 1) and determine the forces acting on it due to the other two particles (Particle 2 and Particle 3).
4. Resolve Forces into Components:
The forces F12 (from Particle 2) and F13 (from Particle 3) can be resolved into horizontal and vertical components. Since the triangle is equilateral, the angle between the line connecting any two particles and the horizontal is 60∘.
- The horizontal components of the forces are:
F12x=F12cos(60∘)=Gm2a2⋅12=Gm22a2
F13x=F13cos(60∘)=Gm2a2⋅12=Gm22a2
- The vertical components of the forces are:
F12y=F12sin(60∘)=Gm2a2⋅√32=√3Gm22a2
F13y=F13sin(60∘)=Gm2a2⋅√32=√3Gm22a2
5. Calculate Net Forces:
- The net horizontal force Fnet,x is:
Fnet,x=F12x+F13x=Gm22a2+Gm22a2=Gm2a2
- The net vertical force Fnet,y is:
Fnet,y=F12y+F13y=√3Gm22a2+√3Gm22a2=√3Gm2a2
6. Calculate the Resultant Force:
The resultant force Fnet can be calculated using the Pythagorean theorem:
Fnet=√F2net,x+F2net,y=√(Gm2a2)2+(√3Gm2a2)2
=√G2m4a4+3G2m4a4=√4G2m4a4=2Gm2a2
7. Final Result:
The net force exerted on one particle due to the other two is:
Fnet=2Gm2a2
We have three identical particles, each of mass m, placed at the corners of an equilateral triangle with side length a.
The gravitational force F between any two particles is given by Newton's law of gravitation:
F=Gm2a2
where G is the gravitational constant.
Let's focus on one particle (let's call it Particle 1) and determine the forces acting on it due to the other two particles (Particle 2 and Particle 3).
The forces F12 (from Particle 2) and F13 (from Particle 3) can be resolved into horizontal and vertical components. Since the triangle is equilateral, the angle between the line connecting any two particles and the horizontal is 60∘.
F12x=F12cos(60∘)=Gm2a2⋅12=Gm22a2
F13x=F13cos(60∘)=Gm2a2⋅12=Gm22a2
F12y=F12sin(60∘)=Gm2a2⋅√32=√3Gm22a2
F13y=F13sin(60∘)=Gm2a2⋅√32=√3Gm22a2
- The net horizontal force Fnet,x is:
Fnet,x=F12x+F13x=Gm22a2+Gm22a2=Gm2a2
- The net vertical force Fnet,y is:
Fnet,y=F12y+F13y=√3Gm22a2+√3Gm22a2=√3Gm2a2
The resultant force Fnet can be calculated using the Pythagorean theorem:
Fnet=√F2net,x+F2net,y=√(Gm2a2)2+(√3Gm2a2)2
=√G2m4a4+3G2m4a4=√4G2m4a4=2Gm2a2
The net force exerted on one particle due to the other two is:
Fnet=2Gm2a2
Topper's Solved these Questions
- GRAVITATIONBOOK - ALLENCHAPTER - GRAVITATIONEXERCISE - BOX-2
13 Videos
- GRAVITATIONBOOK - ALLENCHAPTER - GRAVITATIONEXERCISE - BOX-3
8 Videos
- GRAVITATIONBOOK - ALLENCHAPTER - GRAVITATIONEXERCISE - Illustration
34 Videos
- GEOMETRICAL OPTICSBOOK - ALLENCHAPTER - GEOMETRICAL OPTICSEXERCISE - subjective
14 Videos
- KINEMATICS-2DBOOK - ALLENCHAPTER - KINEMATICS-2DEXERCISE - Exercise (O-2)
46 Videos
Similar Questions
Figure shows, three long straight wires parallel and equally speed with identical currents. Then, the force acting on each wire due to the other is
View SolutionTwo men support a uniform horizontal beam at its two ends, if one of them suddenly lets go, the force exerted by the beam on the other man will
View SolutionThree identical particles each of mass m are placed at the vertices of an equilateral triangle of side a. Fing the force exerted by this system on a particle P of mass m placed at the

(a) the mid point of a side
(b) centre of the triangle
View SolutionThree point charges q are placed at three vertices of an equilateral triangle of side a. Find magnitude of electric force on any charge due to the other two.
View SolutionThree uniform spheres each having a mass M and radius a are kept in such a way that each touches the other two. Find the magnitude of the gravitational force on any of the spheres due to the other two.
View SolutionThree uniform spheres each having a mass M and radius a are kept in such a way that each touches the other two. Find the magnitude of the gravitational force on any of the spheres due to the other two.
View SolutionThree uniform spheres each having a mass M and radius a are kept in such a way that each touches the other two. Find the magnitude of the gravitational force on any of the spheres due to the other two.
View SolutionA particle is attached to an end of a rigid rod. The other end of the rod is hunged and the rod rotates always remaining horizontal. Its angular speed is increasing at contant rate. The mass of the particle is m . The force exerted by the rod on the particle is →F , then:
View SolutionA figure below shows the forces that three charged particles exert on each other. Which of the four situations shown can be correct .

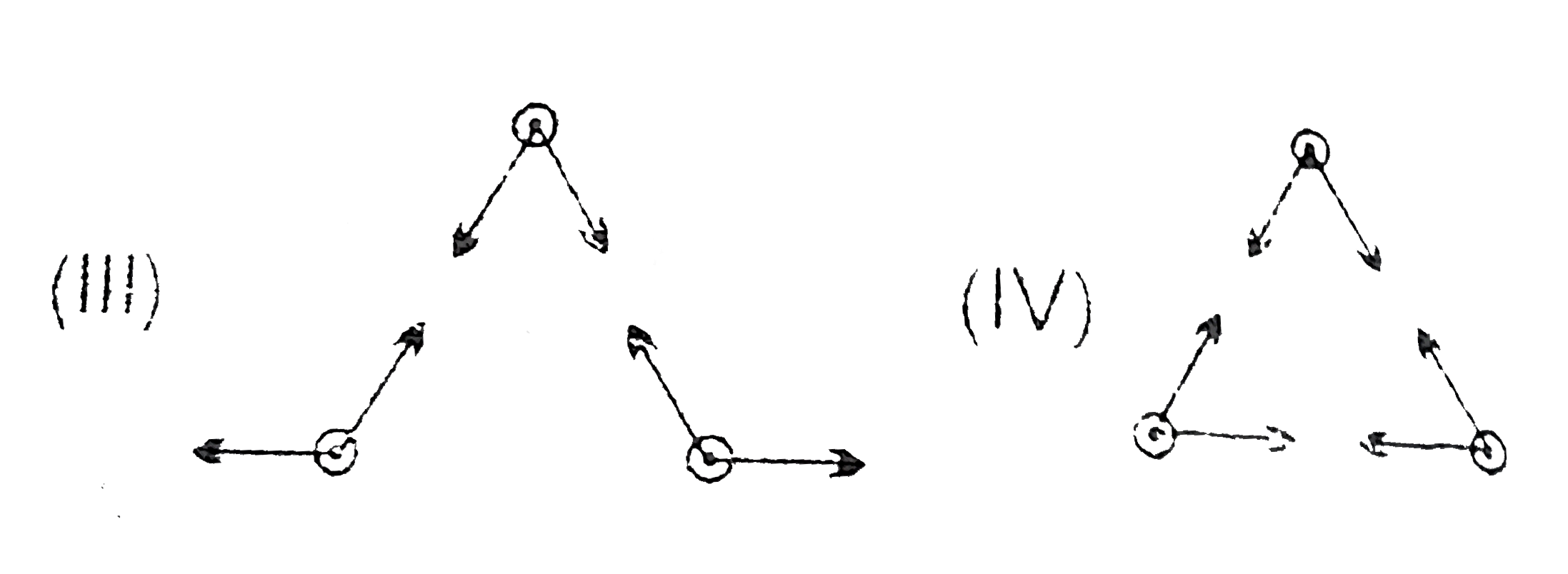
View SolutionConsider a system of two identical particles. One of the particles is at rest and the other has an acceleration. The centre of mass has an acceleration.
View Solution
