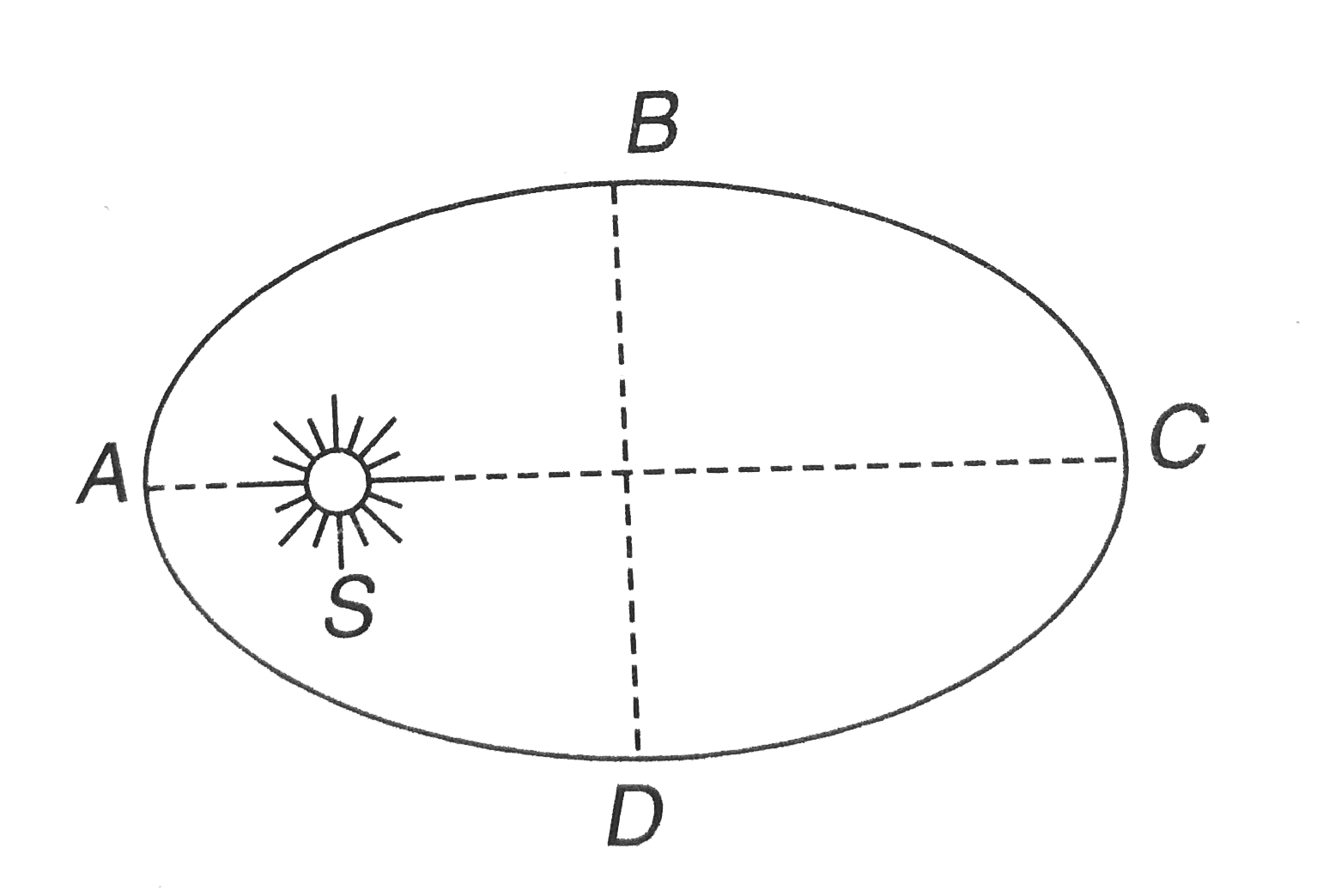
A planet is revolving around the sun. its distance from the sun at apogee is $r_{A}$ and that at perigee is $r_{p}$ . The masses of planet and sun are 'm' and $M$ respectively, $V_{A}$ is the velocity of planet at apogee and $V_{P}$ is at perigee respectively and $T$ is the time period of revolution of planet around the sun, then identify the wrong answer.

A

(a) $T^{2} = \frac{π^{2}}{2 G m} {(r_{A} + r_{p})}_{A}^{3}$

B

(b) $T^{2} = \frac{π^{2}}{2 G m} {(r_{A} + r_{p})}_{A}^{2}$

C

(c) $v_{A} r_{A} = v_{p} r_{p}$

D

(d) $v_{A} < v_{p}, r_{A} > r_{p}$

Video Solution

Text Solution

Generated By DoubtnutGPT

The correct Answer is:C

To solve the problem, we need to analyze the motion of a planet revolving around the sun, focusing on its characteristics at apogee and perigee. We will derive relationships between the velocities, distances, and the time period of revolution, and then identify the incorrect statement among the options provided.
1. Understanding the Problem:
- The planet has two key positions in its orbit: apogee (farthest point from the sun) and perigee (closest point to the sun).
- At apogee, the distance from the sun is $r_{A}$ and the velocity is $V_{A}$ .
- At perigee, the distance from the sun is $r_{P}$ and the velocity is $V_{P}$ .
- The mass of the planet is $m$ and the mass of the sun is $M$ .
- The time period of revolution is $T$ .
2. Applying Conservation of Angular Momentum:
- The angular momentum $L$ of the planet at any point in its orbit is given by:
$L = m \cdot V \cdot r$
- At perigee:
$L_{P} = m \cdot V_{P} \cdot r_{P}$
- At apogee:
$L_{A} = m \cdot V_{A} \cdot r_{A}$
- By conservation of angular momentum:
$L_{P} = L_{A} ⟹ m \cdot V_{P} \cdot r_{P} = m \cdot V_{A} \cdot r_{A}$
- Simplifying gives:
$V_{P} \cdot r_{P} = V_{A} \cdot r_{A}$
- This implies that the product of velocity and distance is constant.
3. Analyzing Velocities:
- Since $r_{A} > r_{P}$ (the distance at apogee is greater than at perigee), it follows that:
$V_{A} < V_{P}$
- Therefore, the velocity at apogee is less than the velocity at perigee.
4. Finding the Time Period:
- The gravitational force provides the centripetal force required for circular motion:
$F_{g} = \frac{G M m}{r^{2}} = \frac{m V^{2}}{r}$
- For an elliptical orbit, we can use the average distance $a$ (semi-major axis):
$a = \frac{r_{A} + r_{P}}{2}$
- The orbital velocity can be expressed as:
$V = \sqrt{\frac{G M}{a}}$
- The time period $T$ can be derived using Kepler's third law:
$T^{2} = \frac{4 π^{2} a^{3}}{G M}$
- Substituting $a$ :
$T^{2} = \frac{4 π^{2}}{G M} {(\frac{r_{A} + r_{P}}{2})}^{3}$
5. Identifying the Incorrect Statement:
- Based on the analysis:
- Option A: Correct (relates to time period).
- Option C: Correct (relates to angular momentum).
- Option D: Correct (relates to velocity comparison).
- Option B: Incorrect (likely misstates a relationship).
Conclusion:
The incorrect statement is Option B.

Updated on:7/8/2024

|

Class 12 PHYSICS GRAVITATION

Topper's Solved these Questions

Similar Questions

Knowledge Check

A planet is revolving in an elliptical orbit around the sun. Its closest distance from the sun is r and the farthest distance is R. If the velocity of the planet nearest to the sun be v and that farthest away from the sun be V. then v/V is
A $R^{2} / r^{2}$
B $r^{2} / R^{2}$
C $R / r$
D $r / R$
A planet revolves around the sun in an elliptical orbit. If $v_{p}$ and $v_{a}$ are the velocities of the planet at the perigee and apogee respectively, then the eccentricity of the elliptical orbit is given by :
A $\frac{v_{p}}{v_{a}}$
B $\frac{v_{a} - v_{p}}{v_{a} + v_{p}}$
C $\frac{v_{p} + v_{a}}{v_{p} - v_{a}}$
D $\frac{v_{p} - v_{a}}{v_{p} + v_{a}}$

ALLEN-GRAVITATION-EXERCISE 1

Home
Ask Doubt
Profile