For a straight line y=43x−4. Choose correct alternate(s)
dydx=tan53∘
dxdy=tan37∘
x−Intercept is 3
length of line between x-axis and y-axis is 5 units
The correct Answer is:A, B, C, D
To solve the problem regarding the straight line given by the equation y=43x−4, we will analyze the equation step-by-step and determine the correctness of the provided alternatives.
Step 1: Identify the slope of the line
The equation of the line is in the slope-intercept form y=mx+b, where m is the slope and b is the y-intercept.
- Here, the slope m=43.
Step 2: Find the angle of inclination
The slope of the line can be related to the angle of inclination θ using the tangent function:
tan(θ)=slope=43
To find the angle θ:
θ=tan−1(43)
Step 3: Calculate the x-intercept
To find the x-intercept, set y=0 in the equation:
0=43x−4
Solving for x:
43x=4⟹x=4⋅34=3
Thus, the x-intercept is 3.
Step 4: Find the length of the line segment between the x-axis and y-axis
The y-intercept can be found by substituting x=0:
y=43(0)−4=−4
The points of intersection with the axes are (3,0) and (0,−4).
To find the length of the line segment between these two points, we can use the distance formula:
Distance=√(x2−x1)2+(y2−y1)2
Substituting the points (3,0) and (0,−4):
Distance=√(3−0)2+(0−(−4))2=√32+42=√9+16=√25=5
Conclusion
Based on the calculations:
1. The slope of the line is 43.
2. The angle of inclination θ is tan−1(43).
3. The x-intercept is 3.
4. The length of the line segment between the x-axis and y-axis is 5 units.
Thus, all the alternatives provided in the question are correct.
The equation of the line is in the slope-intercept form y=mx+b, where m is the slope and b is the y-intercept.
The slope of the line can be related to the angle of inclination θ using the tangent function:
To find the x-intercept, set y=0 in the equation:
The y-intercept can be found by substituting x=0:
Based on the calculations:
1. The slope of the line is 43.
2. The angle of inclination θ is tan−1(43).
3. The x-intercept is 3.
4. The length of the line segment between the x-axis and y-axis is 5 units.
Topper's Solved these Questions
- RACEBOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (Integration)
12 Videos
- RACEBOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (Graphs))
29 Videos
- RACEBOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (Trigonometry)
22 Videos
- NEWTONS LAWS OF MOTIONBOOK - ALLENCHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - EXERCISE-III
28 Videos
- SIMPLE HARMONIC MOTIONBOOK - ALLENCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Example
1 Videos
Similar Questions
A particle moves in a straight line with constant acceleration under a constant force F. Select the correct alternative(s).
View SolutionThe slope of straight line √3y=3x+4 is
View SolutionDisplacement time graph of a particle moving in a straight line is as shown in figure. Select the correct alternative (s) :
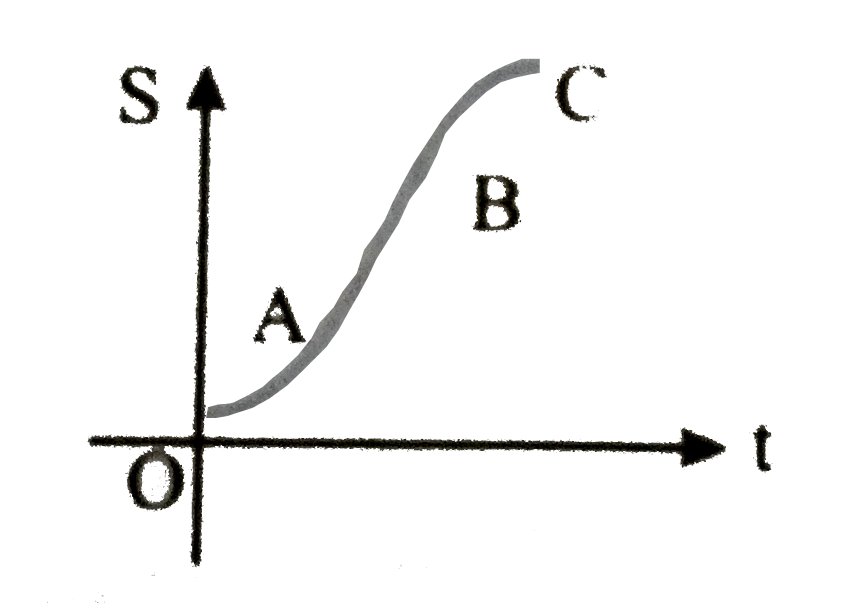 .
.
View SolutionThe point P(a,b) lies on the straight line 3x+2y=13 and the point Q(b,a) lies on the straight line 4x−y=5 , then the equation of the line PQ is
View SolutionThe point P(a,b) lies on the straight line 3x+2y=13 and the point Q(b,a) lies on the straight line 4x−y=5 , then the equation of the line PQ is
View SolutionThe values that m can take so that the straight line y=4x+m touches the curve x2+4y2=4 is
View SolutionFind the equation of a straight line through the point of intersection of the lines 4x−3y=0 and 2x−5y+3=0 and parallel to 4x+5y+6=0.
View SolutionEquation of the plane containing the straight line x2=y3=z4 and perpendicular to the plane containing the straight lines x2=y4=z2 and x4=y2=z3 is
View SolutionFind the equation of the straight line whose intercepts on the axes are twice the intercepts of the straight line 3x+4y=6.
View SolutionEquation of the straight line which belongs to the system of straight lines a(2x+y−3)+b(3x+2y−5)=0 and is farthest from the pint (4,−3) is
View Solution
