A block of mass m is released on the top of a smooth inclined plane of length x and inclination θ as shown in figure. Horizontal surface is rough. If block comes to rest after moving a distabce d on the horizontal surface, then coefficient of friction between block and surface is :-
xsinθ2d
xcosθ2d
xsinθd
xcosθd
The correct Answer is:C
To solve the problem step by step, we will analyze the motion of the block on the inclined plane and the horizontal surface.
Step 1: Understand the Energy Conservation
When the block is released from the top of the inclined plane, it will convert its potential energy into kinetic energy as it moves down the incline. The potential energy at the top is given by:
PE=mgh
where h is the height of the incline.
Step 2: Determine the Height
The height h can be expressed in terms of the length of the incline x and the angle θ:
h=xsinθ
Step 3: Write the Kinetic Energy Equation
As the block reaches the bottom of the incline, all the potential energy will convert into kinetic energy:
KE=12mv2
Setting the potential energy equal to the kinetic energy gives:
mgh=12mv2
Step 4: Substitute for Height
Substituting h into the equation:
mg(xsinθ)=12mv2
We can cancel m from both sides (assuming m≠0):
g(xsinθ)=12v2
Step 5: Solve for Velocity
Rearranging gives us the velocity v at the bottom of the incline:
v2=2g(xsinθ)
v=√2g(xsinθ)
Step 6: Analyze Motion on the Horizontal Surface
When the block reaches the horizontal surface, it will experience friction, which will decelerate it until it comes to rest after traveling a distance d. The frictional force f acting on the block is given by:
f=μmg
where μ is the coefficient of friction.
Step 7: Apply Newton's Second Law
Using Newton's second law, the deceleration a due to friction can be expressed as:
ma=μmg
Thus, the deceleration a is:
a=μg
Step 8: Use Kinematic Equation
Using the kinematic equation for motion:
v2=u2+2ad
where u is the initial velocity (which we found earlier), v=0 (final velocity), and d is the distance traveled on the horizontal surface:
0=v2−2(μg)d
Substituting for v2:
0=2g(xsinθ)−2(μg)d
Step 9: Rearrange to Find Coefficient of Friction
Rearranging gives:
2(μg)d=2g(xsinθ)
Dividing both sides by 2g:
μd=xsinθ
Thus, the coefficient of friction μ is:
μ=xsinθd
Final Answer
The coefficient of friction between the block and the surface is:
μ=xsinθd
When the block is released from the top of the inclined plane, it will convert its potential energy into kinetic energy as it moves down the incline. The potential energy at the top is given by:
PE=mgh
where h is the height of the incline.
The height h can be expressed in terms of the length of the incline x and the angle θ:
h=xsinθ
As the block reaches the bottom of the incline, all the potential energy will convert into kinetic energy:
KE=12mv2
Setting the potential energy equal to the kinetic energy gives:
mgh=12mv2
Substituting h into the equation:
mg(xsinθ)=12mv2
We can cancel m from both sides (assuming m≠0):
g(xsinθ)=12v2
Rearranging gives us the velocity v at the bottom of the incline:
v2=2g(xsinθ)
v=√2g(xsinθ)
When the block reaches the horizontal surface, it will experience friction, which will decelerate it until it comes to rest after traveling a distance d. The frictional force f acting on the block is given by:
f=μmg
where μ is the coefficient of friction.
Using Newton's second law, the deceleration a due to friction can be expressed as:
ma=μmg
Thus, the deceleration a is:
a=μg
Using the kinematic equation for motion:
v2=u2+2ad
where u is the initial velocity (which we found earlier), v=0 (final velocity), and d is the distance traveled on the horizontal surface:
0=v2−2(μg)d
Substituting for v2:
0=2g(xsinθ)−2(μg)d
Rearranging gives:
2(μg)d=2g(xsinθ)
Dividing both sides by 2g:
μd=xsinθ
Thus, the coefficient of friction μ is:
μ=xsinθd
The coefficient of friction between the block and the surface is:
μ=xsinθd
Topper's Solved these Questions
- RACEBOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (CIRCULAR MOTION)
17 Videos
- RACEBOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (COLLISION AND CENTRE OF MASS )
12 Videos
- RACEBOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (FRICTION)
14 Videos
- NEWTONS LAWS OF MOTIONBOOK - ALLENCHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - EXERCISE-III
28 Videos
- SIMPLE HARMONIC MOTIONBOOK - ALLENCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Example
1 Videos
Similar Questions
A block of mass m slides from the top of an inclined plane of angle of inclination θ & length l. The coefficient of friction between the plane and the block is μ. Then it is observed over a distance d along the horizontal surface having the same coefficient of friction μ, before it comes to a stop. Find the value of d.
View SolutionA block of mass m is placed on a smooth inclined plane of inclination θ with the horizontal. The force exerted by the plane on the block has a magnitude
View Solution
Knowledge Check
A block slides with a velocity of 10 m/sec. on a rough horizontal surface. It comes to rest after covering of 50 m. If g is 10 m/sec2, then the coefficient of dynamic friction between the block and surface is
A1B10C2D0.1
A block of mass m is placed on a smooth inclined plane of inclination θ with the horizontal. The force exerted by the plane on the block has a magnitude
View SolutionA block of mass 4 kg is released from the top of an inclined smooth surface as shown in figure If spring constant of spring is 100N/m and block comes to rest after compressing spring by 1 m, find the distance travelled by the block before it comes t o rest.

View SolutionA block of mass 8 kg is released from the top of an inclined smooth surface as shown in figure. If spring constant of spring is 200 Nm^(-1) and block comes to rest after compressing spring by 1 m then find the distance travelled by block before it comes to rest
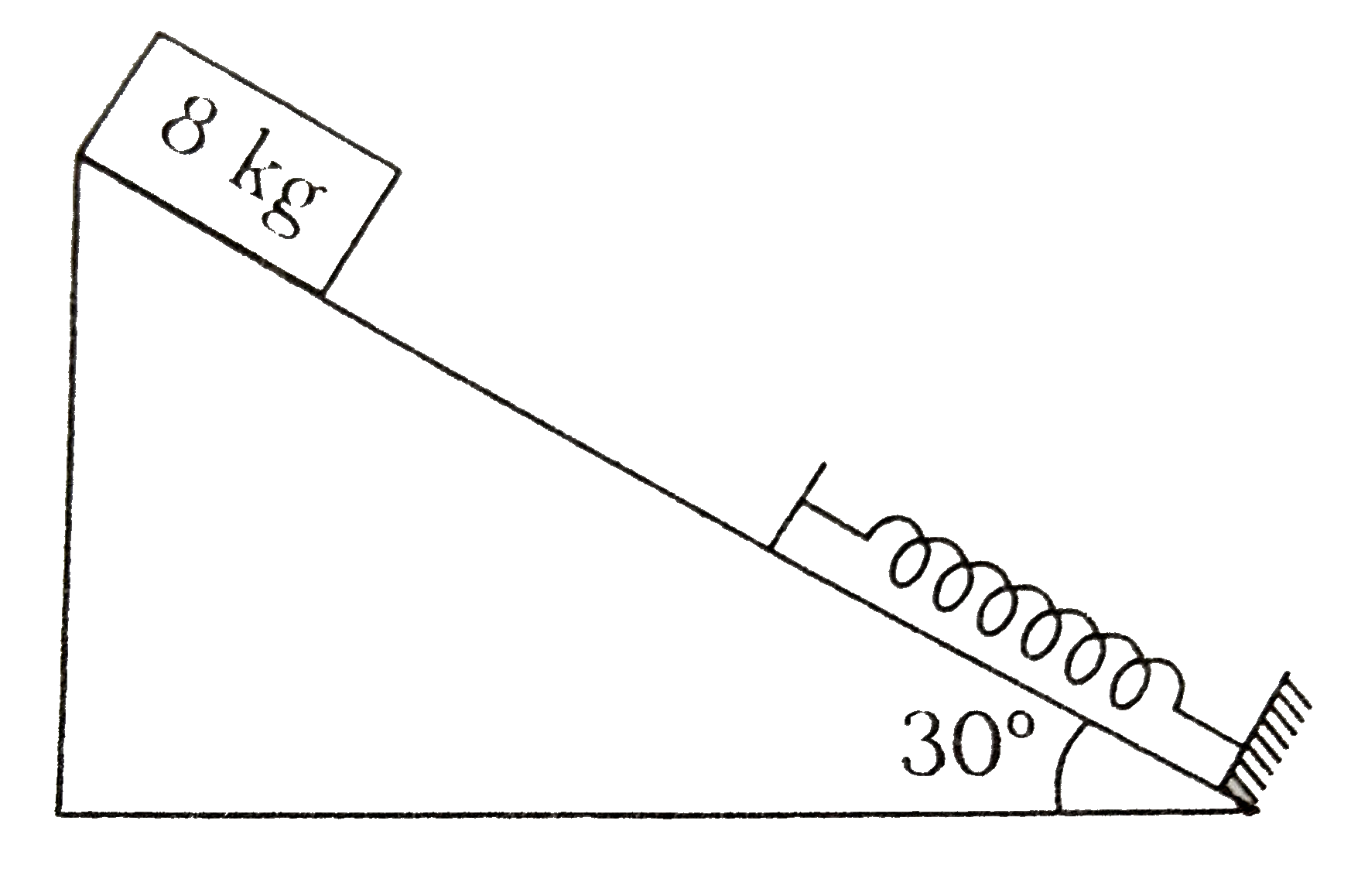
View SolutionA block of mass 8 kg is released from the top of an inclined smooth surface as shown in figure. If spring constant of spring is 200 Nm^(-1) and block comes to rest after compressing spring by 1 m then find the distance travelled by block before it comes to rest

View SolutionA block of mass m moves with constant speed down the inclined plane of inclination θ. Find the coefficient of kinetic friction.

View SolutionA block of mass m takes time t to slide down on a smooth inclined plane of angle of inclination θ and height h. If same block slids down on a rough inclined plane of same angle of inclination and same height and takes time n times of initial value, then coefficient friction between block and inclined plane is
View SolutionA block is sliding on a rough horizontal surface. If the contact force on the block is √2 times the frictional force, the coefficient of friction is
View Solution
