Determine the light pressure exerted on the walls of an electric bulb of power . The bulb is made in the form of a sphere of radius , its walls reflect of the incident light and the rest of the light is transmitted. Assume that the entire power consumed by the bulb is converted into radiation and transmitted light does not exert any pressure.
To determine the light pressure exerted on the walls of an electric bulb of power 100 W, we can follow these steps:
Step 1: Understand the Problem
We need to calculate the light pressure exerted on the walls of a spherical electric bulb. The bulb has a power output of 100 W, a radius of 5 cm, and reflects 10% of the incident light.
Step 2: Calculate the Surface Area of the Bulb
The surface area A of a sphere is given by the formula:
A=4πr2
where r is the radius of the sphere.
Given:
- r=5cm=0.05m
Calculating the surface area:
A=4π(0.05)2=4π(0.0025)=0.01πm2
Step 3: Calculate the Total Power Reflected
The total power P of the bulb is 100 W. Since the walls reflect 10% of the incident light, the power reflected Pr is:
Pr=0.10×P=0.10×100=10W
Step 4: Calculate the Total Energy Incident on the Surface
The energy incident on the walls of the bulb per second (which is equal to the power) is given by:
U=P×A=100W×0.01πm2=1πJ/s
Step 5: Calculate the Radiation Pressure
The radiation pressure Rp is given by the formula:
Rp=Uc
where c is the speed of light, approximately 3×108m/s.
Since the reflected light doubles the pressure, we need to consider this in our calculation:
Rp=2×Uc
Substituting the values:
Rp=2×(1π)3×108=2π3×108N/m2
Step 6: Final Calculation
Calculating the numerical value:
Rp≈2×3.143×108≈6.283×108≈2.093×10−9N/m2
Conclusion
The light pressure exerted on the walls of the electric bulb is approximately:
Rp≈2.093×10−9N/m2
We need to calculate the light pressure exerted on the walls of a spherical electric bulb. The bulb has a power output of 100 W, a radius of 5 cm, and reflects 10% of the incident light.
The surface area A of a sphere is given by the formula:
A=4πr2
where r is the radius of the sphere.
- r=5cm=0.05m
A=4π(0.05)2=4π(0.0025)=0.01πm2
The total power P of the bulb is 100 W. Since the walls reflect 10% of the incident light, the power reflected Pr is:
Pr=0.10×P=0.10×100=10W
The energy incident on the walls of the bulb per second (which is equal to the power) is given by:
U=P×A=100W×0.01πm2=1πJ/s
The radiation pressure Rp is given by the formula:
Rp=Uc
where c is the speed of light, approximately 3×108m/s.
Rp=2×Uc
Substituting the values:
Rp=2×(1π)3×108=2π3×108N/m2
Calculating the numerical value:
Rp≈2×3.143×108≈6.283×108≈2.093×10−9N/m2
The light pressure exerted on the walls of the electric bulb is approximately:
Rp≈2.093×10−9N/m2
Similar Questions
A 100 W light bulb is placed at the centre of a spherical chamber of radius 20cm. Assume that 60% of the energy supplied to the bulb is converted into light and that the surface of the chamber is perfectly absorbing. Find the pressure exerted by the light on the surface of the chamber.
View SolutionA sphere of radius 1.00 cm is placed in the path of a parallel beam of light of large aperture. The intensity of the light is 0.50 W cm−2. If the sphere completely absorbs the radiation falling on it, find the force exerted by the light beam on the sphere.
View SolutionA beam of white light is incident normaly on a plane surface absorbing 70% of the light and reflecting the rest. If the incident beam carries 10 w of power, find the force exerated by it on the surface.
View SolutionA beam of white light is incident normally on a plane surface absorbing (70%) of the light and reflecting the rest. If the incident beam carries 10 W of power , find the force exerted by it on the surface .
View SolutionA parallel beam of light is incident normally on a plane surface absorbing 40% of the light and reflecting the rest. If the incident beam carries 60 W of power, the force exerted by it on the surface is
View SolutionA laser beam of power 20W incident normally on a surface. if 25% light is absorbed and rest reflected find magnitude of force experienced by the surface.
View SolutionHow many photons per second does a one watt bulb emit if its efficiency is 10% and the wavelength of light emitted is 500 nm
View SolutionIn a modified Young's double-slit experiment, a monochromatic uniform and parallel beam of light of wavelength 6000Å and intensity (10/π) W m−2 is incident normally on two circular apertures A and B of radii 0.001 m and 0.002 m, respectively. A perfectly transparent film of thickness 2000Å and refractive index 1.5 for the wavelength of 6000Å is placed in front of aperture A (see the figure). Calculate the power (in mW) received at the focal spot F of the lens. Then lens is symmetrically placed with respect to the aperture. Assume that 10% of the power received by each aperture goes in the original direction and is brought to the focal spot.
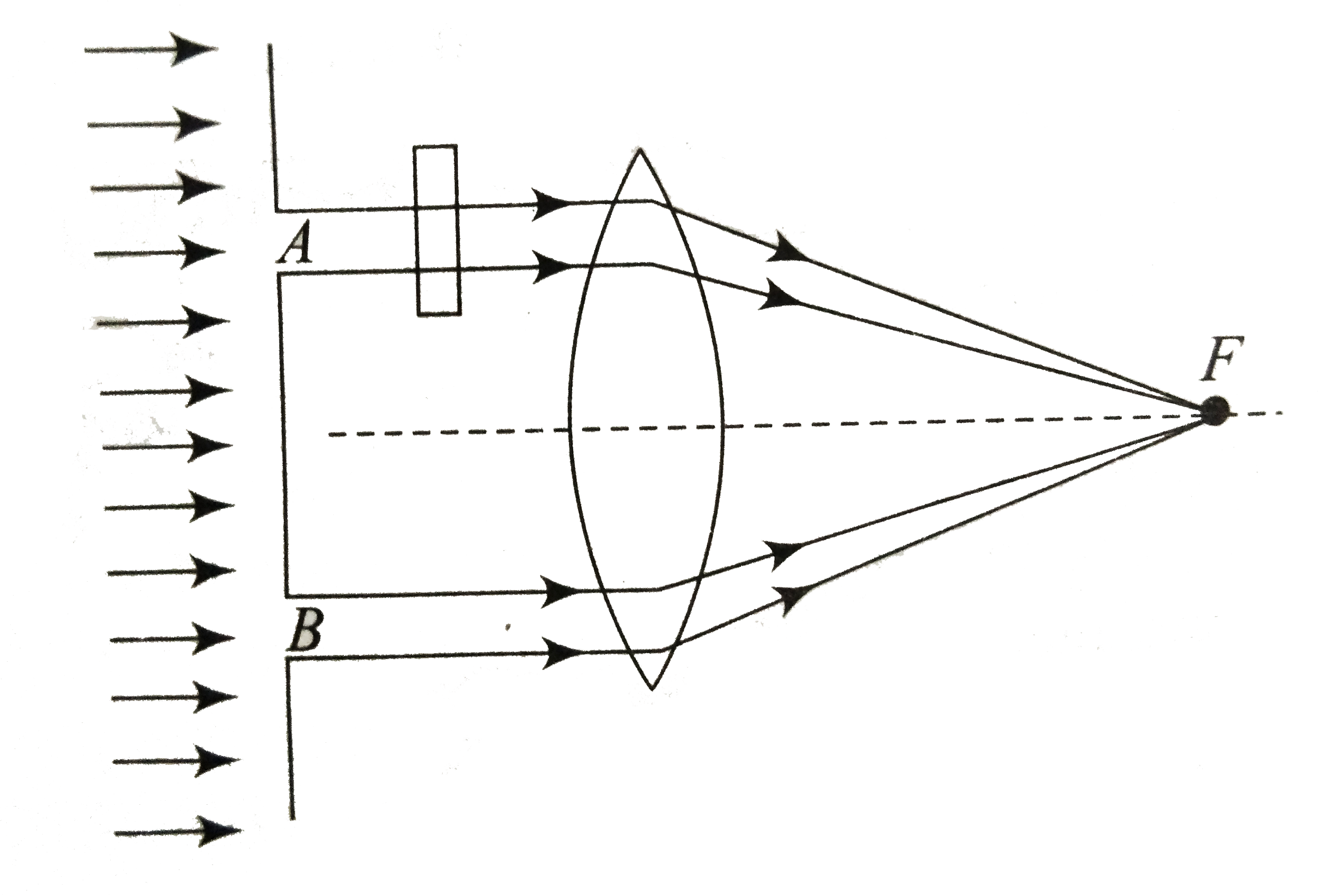
View SolutionThe number of photons emitted by a 60 W bulb per second, if 10% of the electrical energy supplied to an incandescent light bulb is radiated as visible light, is
View SolutionAbout 5% of the power of a 100W light bulb is converted to visible radiation. What is the average intensity of visible radiation
(a) at a distance of 1m from the bulb?
(b) at a distance of 10m ?
Assume that the radiation is esmitted isotropically and neglect reflection.
View Solution
