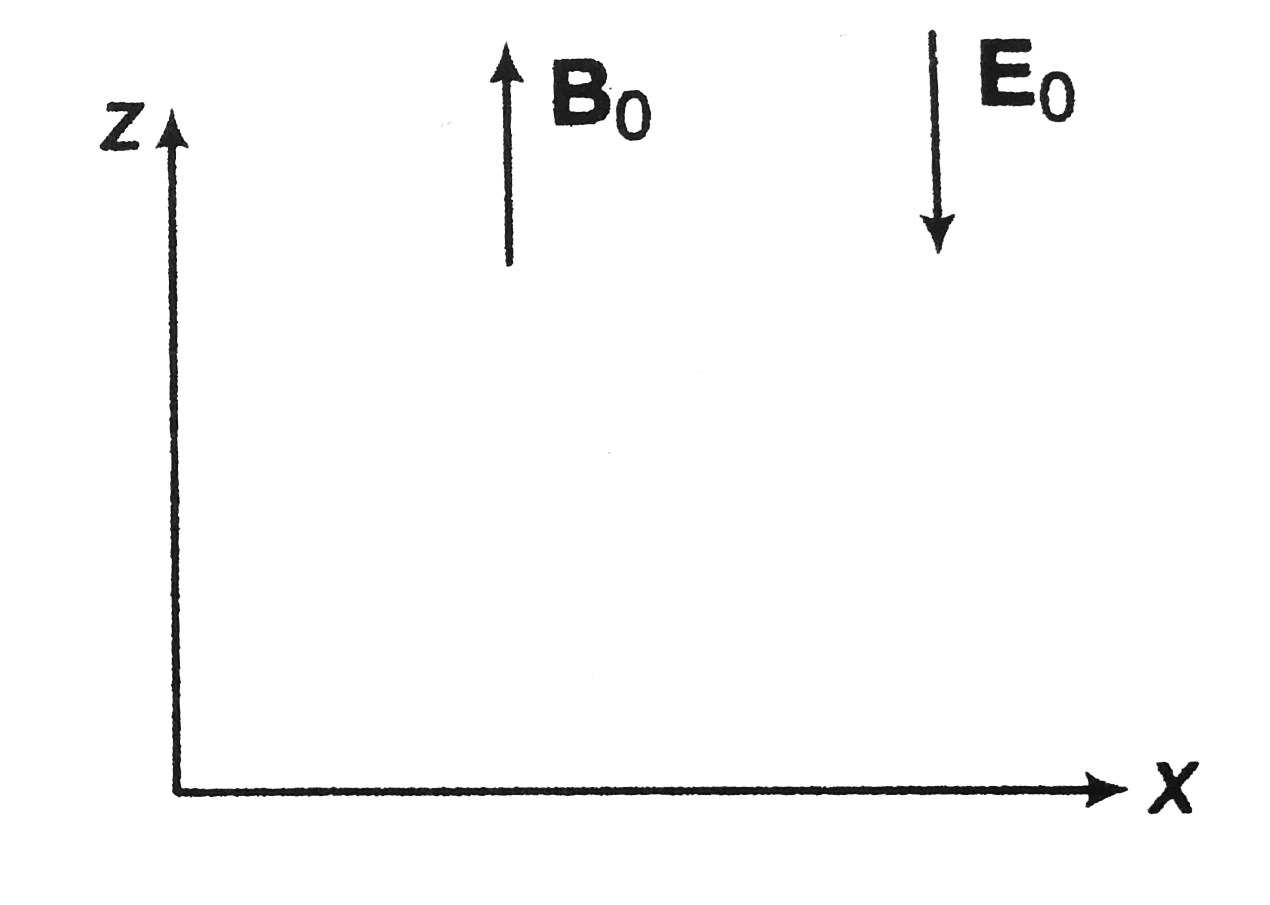
The space has electromagnetic field which is given as →B=−B0ˆk and →E=E0ˆk. A charged particle having mass m and positive charge q is given velocity v0ˆi at origin at t=0sec. The z coordinate of the particle when it passes through the z−axis is (neglect gravity through motion)
2π2mE0qB20
4π2mE0qB20
mE0qB20
−4π2mE0qB20
To solve the problem step by step, we need to analyze the motion of a charged particle in the presence of electric and magnetic fields.
Step 1: Understand the Forces Acting on the Particle
The charged particle experiences two forces due to the electric field →E and the magnetic field →B:
- The electric force →FE=q→E=qE0^k
- The magnetic force →FB=q→v×→B
Given that →B=−B0^k and the initial velocity →v=v0^i, we can calculate the magnetic force.
Step 2: Calculate the Magnetic Force
Using the right-hand rule for the cross product:
→FB=q→v×→B=q(v0^i)×(−B0^k)=−qv0B0(^i×^k)=−qv0B0^j
Thus, the magnetic force acts in the ^j direction.
Step 3: Write the Equation of Motion
The net force acting on the particle is:
→Fnet=→FE+→FB=qE0^k−qv0B0^j
Using Newton's second law, →Fnet=m→a, we can express the acceleration:
m→a=qE0^k−qv0B0^j
Thus, the acceleration →a is:
→a=qE0m^k−qv0B0m^j
Step 4: Determine the Circular Motion
The particle will undergo circular motion in the xy-plane due to the magnetic force, while it will also move in the z-direction due to the electric force. The centripetal force required for circular motion is provided by the magnetic force:
mv2R=qv0B0
where R is the radius of the circular path.
Step 5: Find the Angular Frequency
The angular frequency ω can be expressed as:
ω=qB0m
Step 6: Calculate the Time Period
The time period T of the circular motion is given by:
T=2πω=2πmqB0
Step 7: Calculate the Displacement in the z-direction
The displacement in the z-direction while the particle is in circular motion can be calculated using the formula for displacement under constant acceleration:
z=12azt2
where az=qE0m and t=T2 (since we want the displacement during half a period):
z=12(qE0m)(T2)2
Substituting T:
z=12(qE0m)(12⋅2πmqB0)2
Simplifying this gives:
z=12⋅qE0m⋅π2m2q2B20
z=π2mE02qB20
Final Answer
The z-coordinate of the particle when it passes through the z-axis is:
z=π2mE02qB20
The charged particle experiences two forces due to the electric field →E and the magnetic field →B:
- The electric force →FE=q→E=qE0^k
- The magnetic force →FB=q→v×→B
Using the right-hand rule for the cross product:
→FB=q→v×→B=q(v0^i)×(−B0^k)=−qv0B0(^i×^k)=−qv0B0^j
Thus, the magnetic force acts in the ^j direction.
The net force acting on the particle is:
→Fnet=→FE+→FB=qE0^k−qv0B0^j
Using Newton's second law, →Fnet=m→a, we can express the acceleration:
m→a=qE0^k−qv0B0^j
Thus, the acceleration →a is:
→a=qE0m^k−qv0B0m^j
The particle will undergo circular motion in the xy-plane due to the magnetic force, while it will also move in the z-direction due to the electric force. The centripetal force required for circular motion is provided by the magnetic force:
mv2R=qv0B0
where R is the radius of the circular path.
The angular frequency ω can be expressed as:
ω=qB0m
The time period T of the circular motion is given by:
T=2πω=2πmqB0
The displacement in the z-direction while the particle is in circular motion can be calculated using the formula for displacement under constant acceleration:
z=12azt2
where az=qE0m and t=T2 (since we want the displacement during half a period):
z=12(qE0m)(T2)2
Substituting T:
z=12(qE0m)(12⋅2πmqB0)2
Simplifying this gives:
z=12⋅qE0m⋅π2m2q2B20
z=π2mE02qB20
The z-coordinate of the particle when it passes through the z-axis is:
z=π2mE02qB20
Similar Questions
A charge q of mass m enters a region of electric field E=−E0ˆj with a velocity v0ˆi+v0ˆj at origin. Find the x coordinate where the charge hits the x axis again. (Neglect effect of gravity)
View SolutionA charge particle of mass m and charge q is projected with velocity v along y-axis at t=0.

Find the velocity vector and position vector of the particle →v(t)and→r(t) in relation with time.
View SolutionThere exist uniform magnetic and electric fields of manitudes 1T and 1Vm−1 respectively, along positive y-axis. A charged particle of mass 1kg and charge 1C is having velocity 1ms−1 along x-axis and is at origin at t=0. Then, the coordinates of the particle at time πs will be
View SolutionThere exist uniform magnetic and electric fields of manitudes 1T and 1Vm−1 respectively, along positive y-axis. A charged particle of mass 1kg and charge 1C is having velocity 1ms−1 along x-axis and is at origin at t=0. Then, the coordinates of the particle at time πs will be
View SolutionA charged particle (charge q, mass m) has velocity v0 at origin in +x direction. In space there is a uniform magnetic field B in -z direction. Find the y coordinate of particle when is crosses y axis.
View SolutionA non-uniform magnetic field →B=B0(1+yd)(−ˆk) is present in the region of space between y=0 and y=d. A particle of mass m and positive charge q has velocity →v=3qB0dmˆi at origin O. Find the angle made by velocity of the particle withe the positive x-axis when it leaves the field. (Ignore any interaction other than magnetic field)
View SolutionElectric field and magnetic field n a region of space is given by E=E0ˆj and B=B0ˆj. A particle of specific charge alpha is released from origin with velocity v=v0ˆi. Then path of particle
View SolutionA charged particle of mass m and charge q is released from rest the position (x0,0) in a uniform electric field E0ˆj. The angular momentum of the particle about origin.
View SolutionThere exists a uniform magnetic field →B=+B0ˆk for x>0and→B=0 for all x<0. A charged particle placed at (0,−a,0) is given an initial velocity →v=v0ˆi. What is the magnitude and nature of charge on the particle such that it crosses through the origin ?
Strategy: When the charge is projected perpendicular to a uniform magnetic field, it follows a circular path. In this case, the force acting on it will be directed either towards +y-axis. or y-axis. It is given that it crosses point O. Thus, the force at (0,−a,0) must be towards y-axis
View SolutionIn a certain region, uniform electric field E=−E0ˆk and magnetic field B=B0ˆk are present. At time t=0, a particle of mass m and charge q is given a velocity v=vj+vk. Find the minimum speed of the particle and the time when it happens so.
View Solution
