If the mid points of consecutive of a quadrilateral connected by straight lines prove that the resulting quadrilateral is a parallelogram.
To prove that the quadrilateral formed by the midpoints of the sides of a given quadrilateral is a parallelogram, we will follow these steps:
Step 1: Define the Quadrilateral
Let the vertices of the quadrilateral be A,B,C,D. We will denote the midpoints of the sides as follows:
- E is the midpoint of AB
- F is the midpoint of BC
- G is the midpoint of CD
- H is the midpoint of DA
Step 2: Apply the Midpoint Theorem
According to the midpoint theorem, the line segment joining the midpoints of two sides of a triangle is parallel to the third side and is half as long.
Triangle ABC:
- E and F are midpoints of AB and AC, respectively.
- Therefore, by the midpoint theorem:
EF∥ACandEF=12AC(Equation 1)
Triangle ADC:
- H and G are midpoints of AD and DC, respectively.
- Therefore, by the midpoint theorem:
HG∥ACandHG=12AC(Equation 2)
Step 3: Establish Parallelism and Equality
From Equations 1 and 2, we have:
- EF∥AC
- HG∥AC
Since both EF and HG are parallel to the same line AC, it follows that:
EF∥HG
Step 4: Show that the Lengths are Equal
From the midpoint theorem, we also have:
- EF=12AC
- HG=12AC
Thus, we can conclude:
EF=HG
Step 5: Conclusion
Since we have established that:
1. EF∥HG
2. EF=HG
By the definition of a parallelogram (a quadrilateral with opposite sides that are both parallel and equal), we can conclude that quadrilateral EFGH is a parallelogram.
Final Statement
Therefore, the quadrilateral formed by the midpoints of the sides of any quadrilateral is a parallelogram.
---
Let the vertices of the quadrilateral be A,B,C,D. We will denote the midpoints of the sides as follows:
- E is the midpoint of AB
- F is the midpoint of BC
- G is the midpoint of CD
- H is the midpoint of DA
According to the midpoint theorem, the line segment joining the midpoints of two sides of a triangle is parallel to the third side and is half as long.
- E and F are midpoints of AB and AC, respectively.
- Therefore, by the midpoint theorem:
EF∥ACandEF=12AC(Equation 1)
- H and G are midpoints of AD and DC, respectively.
- Therefore, by the midpoint theorem:
HG∥ACandHG=12AC(Equation 2)
From Equations 1 and 2, we have:
- EF∥AC
- HG∥AC
EF∥HG
From the midpoint theorem, we also have:
- EF=12AC
- HG=12AC
EF=HG
Since we have established that:
1. EF∥HG
2. EF=HG
Therefore, the quadrilateral formed by the midpoints of the sides of any quadrilateral is a parallelogram.
Similar Questions
If each diagonals of a quadrilateral separates it into two triangles of equal area then show that the quadrilateral is a parallelogram.
View SolutionIf each diagonals of a quadrilateral separates it into two triangles of equal area then show that the quadrilateral is a parallelogram.
View SolutionProve that the quadrilateral formed by joining the mid-points of the pairs of consecutive sides of a quadrilateral is a parallelogram.
View SolutionIf the mid-points of the sides of a quadrilateral are joined in order, prove that the area of the parallelogram, so formed will be half of the area of the given quadrilateral (figure).
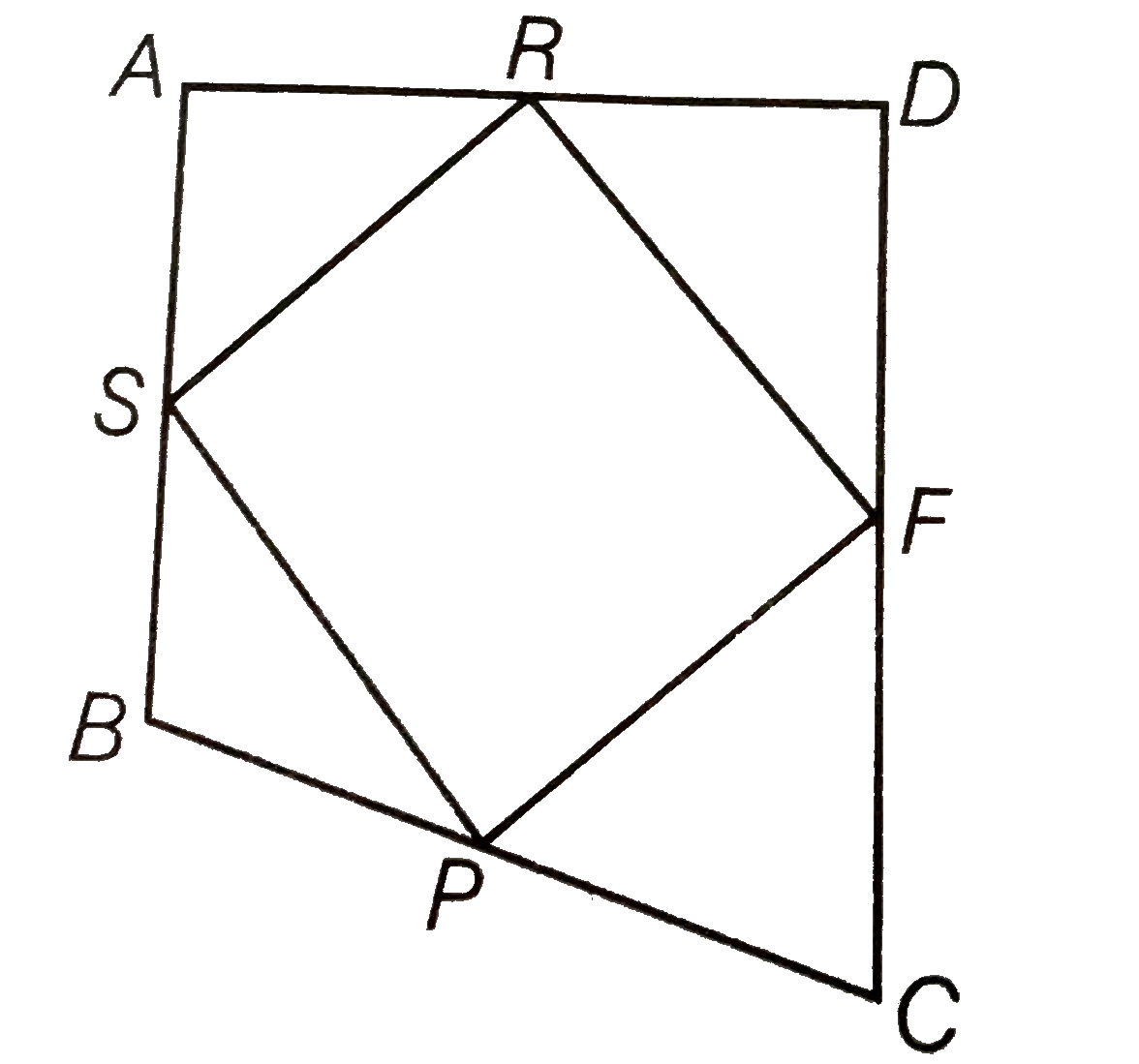
View SolutionIf the diagonals of a quadrilateral bisect each other, then the quadrilateral is a parallelogram.
View SolutionIf the diagonals of a quadrilateral bisect each other at right angle, then the quadrilateral is a (a) parallelogram (b) rectangle (c) rhombus (d) kite
View SolutionIf each diagonal of a quadrilateral separates it into two triangles of equal area then show that the quadrilateral is a parallelogram. GIVEN : A quadrilateral ABCD such that its diagonals AC and BD are such that ar(ABD)=ar(CDB ) and ar(ABC)=ar(ACD). TO PROVE: Quadrilateral ABCD is a parallelogram.
View SolutionIf the diagonals AC,BD of a quadrilateral ABCD, intersect at O, and seqarate the quadrilateral into four triangles of equal area, show that quadrilateral ABCD is a parallelogram. GIVEN : A quadrilateral ABCD such that its diagonals AC and BD intersect at O and separate it into four parts such that ar(AOB)=ar(BOC)=ar(COD)=ar(AOD) TO PROVE : Quadrilateral ABCD is a parallelogram.
View SolutionIf the diagonals AC, BD of a quadrilateral ABCD, intersect at O, and separate the quadrilateral into four triangles of equal area, show that quadrilateral ABCD is a parallelogram.
View SolutionE, F, G and H are the mid-points of the sides of a parallelogram ABCD. Show that area of quadrilateral EFGH is half of the area of parallelogram ABCD.
View Solution
