A dipole of 2μC charges each, consists of the positive charge at the point P (1, -1) and the negative charge is placed at the point Q(-1, 1). The work done in displacing a charge of +1μC from point A(−3,−3) to B (4, 4) is
1.6×10−19J
3.2×10−19J
zero
4.8eV
The correct Answer is:C
To find the work done in displacing a charge of +1μC from point A(−3,−3) to point B(4,4) in the presence of a dipole consisting of charges +2μC at point P(1,−1) and −2μC at point Q(−1,1), we can follow these steps:
Step 1: Understand the Configuration
- We have a dipole with charges:
- Positive charge +2μC at point P(1,−1)
- Negative charge −2μC at point Q(−1,1)
- We need to calculate the work done in moving a charge of +1μC from point A(−3,−3) to point B(4,4).
Step 2: Calculate the Electric Potential at Points A and B
The electric potential \( V \) at a point due to a dipole is given by the formula:
\(
V = k \left( \frac{q_1}{r_1} - \frac{q_2}{r_2} \right)
\)
where:
- \( k \) is Coulomb's constant (\( 9 \times 10^9 \, \text{N m}^2/\text{C}^2 \))
- \( q_1 \) and \( q_2 \) are the charges of the dipole
- \( r_1 \) and \( r_2 \) are the distances from the point of interest to each charge.
Calculate the potential at point A:
1. Distance from A to P:
\(
r_{AP} = \sqrt{(1 - (-3))^2 + (-1 - (-3))^2} = \sqrt{(4)^2 + (2)^2} = \sqrt{16 + 4} = \sqrt{20} = 2\sqrt{5}
\)
2. Distance from A to Q:
\(
r_{AQ} = \sqrt{(-1 - (-3))^2 + (1 - (-3))^2} = \sqrt{(2)^2 + (4)^2} = \sqrt{4 + 16} = \sqrt{20} = 2\sqrt{5}
\)
3. Potential at A:
\(
V_A = k \left( \frac{2 \times 10^{-6}}{2\sqrt{5}} - \frac{(-2) \times 10^{-6}}{2\sqrt{5}} \right) = k \left( \frac{2 \times 10^{-6} + 2 \times 10^{-6}}{2\sqrt{5}} \right) = k \left( \frac{4 \times 10^{-6}}{2\sqrt{5}} \right) = \frac{2k \times 10^{-6}}{\sqrt{5}}
\)
Calculate the potential at point B:
1. Distance from B to P:
\(
r_{BP} = \sqrt{(1 - 4)^2 + (-1 - 4)^2} = \sqrt{(-3)^2 + (-5)^2} = \sqrt{9 + 25} = \sqrt{34}
\)
2. Distance from B to Q:
\(
r_{BQ} = \sqrt{(-1 - 4)^2 + (1 - 4)^2} = \sqrt{(-5)^2 + (-3)^2} = \sqrt{25 + 9} = \sqrt{34}
\)
3. Potential at B:
\(
V_B = k \left( \frac{2 \times 10^{-6}}{\sqrt{34}} - \frac{-2 \times 10^{-6}}{\sqrt{34}} \right) = k \left( \frac{2 \times 10^{-6} + 2 \times 10^{-6}}{\sqrt{34}} \right) = \frac{4k \times 10^{-6}}{\sqrt{34}}
\)
Step 3: Calculate the Work Done
The work done \( W \) in moving the charge is given by:
\(
W = q(V_B - V_A)
\)
Substituting the values:
\(
W = 1 \times 10^{-6} \left( \frac{4k \times 10^{-6}}{\sqrt{34}} - \frac{2k \times 10^{-6}}{\sqrt{5}} \right)
\)
Step 4: Simplify the Expression
Since the potential energy at both points A and B is derived from the same dipole configuration, we find that the work done is equal to the change in potential energy, which can be shown to be zero due to symmetry in the dipole field. Thus:
\(
W = 0
\)
Conclusion
The work done in displacing the charge from point A to point B is:
\(
\boxed{0}
\)
- We have a dipole with charges:
- Positive charge +2μC at point P(1,−1)
- Negative charge −2μC at point Q(−1,1)
- We need to calculate the work done in moving a charge of +1μC from point A(−3,−3) to point B(4,4).
The electric potential \( V \) at a point due to a dipole is given by the formula:
\(
V = k \left( \frac{q_1}{r_1} - \frac{q_2}{r_2} \right)
\)
where:
- \( k \) is Coulomb's constant (\( 9 \times 10^9 \, \text{N m}^2/\text{C}^2 \))
- \( q_1 \) and \( q_2 \) are the charges of the dipole
- \( r_1 \) and \( r_2 \) are the distances from the point of interest to each charge.
1. Distance from A to P:
\(
r_{AP} = \sqrt{(1 - (-3))^2 + (-1 - (-3))^2} = \sqrt{(4)^2 + (2)^2} = \sqrt{16 + 4} = \sqrt{20} = 2\sqrt{5}
\)
\(
r_{AQ} = \sqrt{(-1 - (-3))^2 + (1 - (-3))^2} = \sqrt{(2)^2 + (4)^2} = \sqrt{4 + 16} = \sqrt{20} = 2\sqrt{5}
\)
\(
V_A = k \left( \frac{2 \times 10^{-6}}{2\sqrt{5}} - \frac{(-2) \times 10^{-6}}{2\sqrt{5}} \right) = k \left( \frac{2 \times 10^{-6} + 2 \times 10^{-6}}{2\sqrt{5}} \right) = k \left( \frac{4 \times 10^{-6}}{2\sqrt{5}} \right) = \frac{2k \times 10^{-6}}{\sqrt{5}}
\)
1. Distance from B to P:
\(
r_{BP} = \sqrt{(1 - 4)^2 + (-1 - 4)^2} = \sqrt{(-3)^2 + (-5)^2} = \sqrt{9 + 25} = \sqrt{34}
\)
\(
r_{BQ} = \sqrt{(-1 - 4)^2 + (1 - 4)^2} = \sqrt{(-5)^2 + (-3)^2} = \sqrt{25 + 9} = \sqrt{34}
\)
\(
V_B = k \left( \frac{2 \times 10^{-6}}{\sqrt{34}} - \frac{-2 \times 10^{-6}}{\sqrt{34}} \right) = k \left( \frac{2 \times 10^{-6} + 2 \times 10^{-6}}{\sqrt{34}} \right) = \frac{4k \times 10^{-6}}{\sqrt{34}}
\)
The work done \( W \) in moving the charge is given by:
\(
W = q(V_B - V_A)
\)
Substituting the values:
\(
W = 1 \times 10^{-6} \left( \frac{4k \times 10^{-6}}{\sqrt{34}} - \frac{2k \times 10^{-6}}{\sqrt{5}} \right)
\)
Since the potential energy at both points A and B is derived from the same dipole configuration, we find that the work done is equal to the change in potential energy, which can be shown to be zero due to symmetry in the dipole field. Thus:
\(
W = 0
\)
The work done in displacing the charge from point A to point B is:
\(
\boxed{0}
\)
Similar Questions
Find the work done in taking a charge 100μC from C & D.
View SolutionAs charge q=1μC is placed at point (1m,2m,4m). Find the electric field at point P(0,−4m,3m)
View SolutionA positive point charge q1 is placed at (a,0) and another positive point charge q2 is placed at (0,a) . Find the force acting on q1.
View SolutionAs per this diagram a point charge +q is placed at the origin O. Work done in taking another point charge −Q from the point A(0,a) to another point B(a,0) along the staight path AB is:
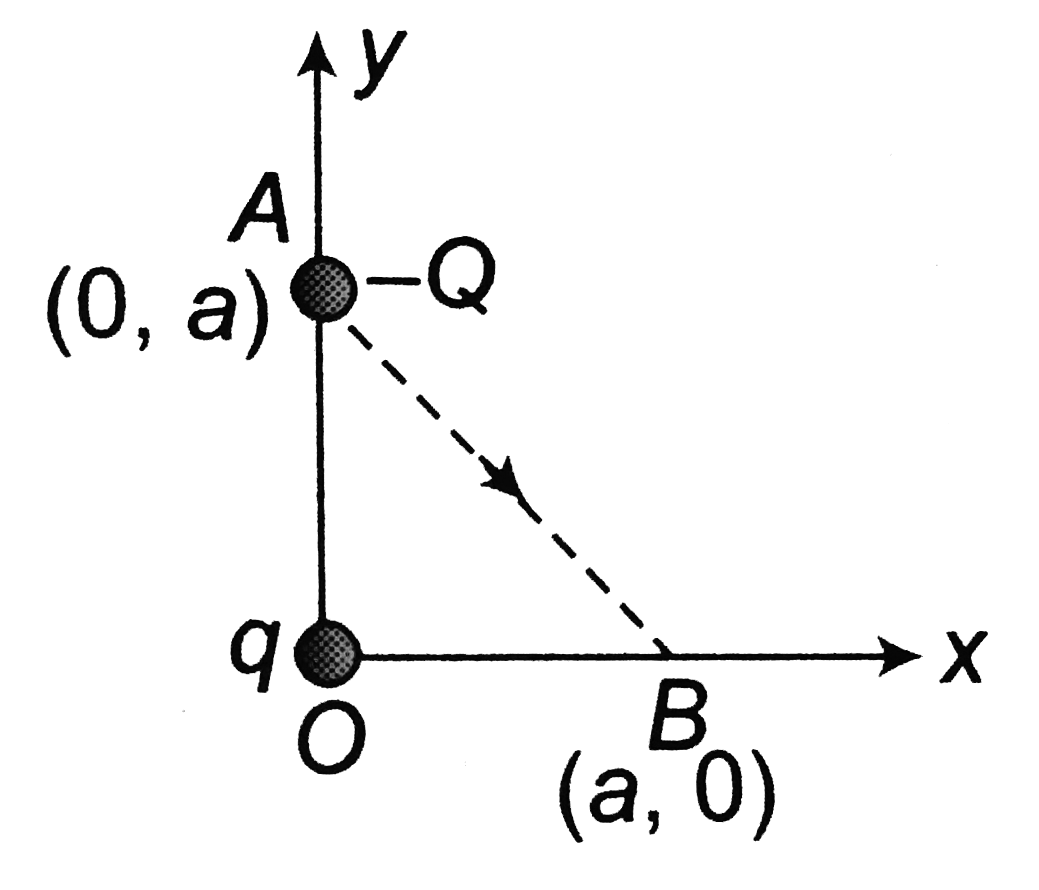
View SolutionAs per this diagram a point charge +q is placed at the origin O. Work done in taking another point charge −Q from the point A(0,a) to another point B(a,0) along the staight path AB is:

View SolutionA point charge +q is placed at origin O. The work done in taking another point charge -q from the point A to point B along the circular arc as shown in the figure is
_PHY_E05_122_Q01.png) .
.
View SolutionA charge Q is placed at the origin. The electric potential due to this charge at a given point in space is V. The work done by an external force in bringing another charge q from infinity up to the point is
View SolutionA short dipole of dipole moment P is placed near a point charge Q as shown in figure. Find force on the dipole due to the point charge
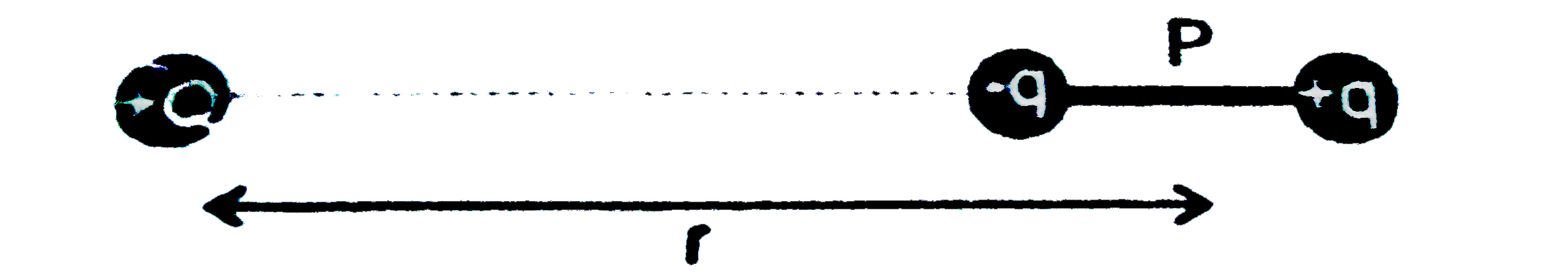
View SolutionA short dipole of dipole moment P is placed near a point charge Q as shown in figure. Find force on the dipole due to the point charge

View SolutionSuppose a charge +Q1 is given to the positive plates and a charge −Q2 to the negative plate of a capacitor. What is the "charge on the capacitor"?
View Solution

