Consider two positive point charges, each of magnitude q, placed on the y-axis at the points (0, a) and (0, -a). A positively charged particle of charge q' and mass m is displaced slightly from the origin in the direction of the positive x-axis.
Speed of charge q' at infinity is
√qq'πε0m
√q'πε0am
√qq'ε0am
√qq'πε0am
The correct Answer is:D
To solve the problem, we need to analyze the forces acting on the charged particle q′ and apply the principle of conservation of energy.
1. Understanding the Setup:
- We have two positive point charges q located at coordinates (0,a) and (0,−a) on the y-axis.
- A positively charged particle q′ with mass m is initially at the origin (0,0) and is displaced slightly in the positive x-direction.
2. Initial Conditions:
- The initial kinetic energy KEi of the charge q′ is zero since it starts from rest.
- The initial potential energy PEi can be calculated using the formula for the electric potential energy between point charges.
3. Calculating Initial Potential Energy:
- The distance from the charge q′ at the origin to each charge q is a.
- The potential energy PE due to one charge q is given by:
PE=14πϵ0q⋅q′r
- Since there are two charges, the total initial potential energy PEi is:
PEi=2⋅14πϵ0q⋅q′a=q⋅q′2πϵ0a
4. Final Conditions:
- At infinity, the potential energy PEf is zero because the distance between charges becomes infinitely large.
- The final kinetic energy KEf can be expressed as:
KEf=12mv2
- Here, v is the speed of the charge q′ at infinity.
5. Applying Conservation of Energy:
- According to the conservation of mechanical energy:
KEi+PEi=KEf+PEf
- Substituting the known values:
0+q⋅q′2πϵ0a=12mv2+0
6. Solving for Speed v:
- Rearranging the equation gives:
12mv2=q⋅q′2πϵ0a
- Multiplying both sides by 2:
mv2=q⋅q′πϵ0a
- Dividing by m:
v2=q⋅q′mπϵ0a
- Taking the square root to find v:
v=√q⋅q′mπϵ0a
Final Answer:
The speed of charge q′ at infinity is:
v=√q⋅q′mπϵ0a
- We have two positive point charges q located at coordinates (0,a) and (0,−a) on the y-axis.
- A positively charged particle q′ with mass m is initially at the origin (0,0) and is displaced slightly in the positive x-direction.
- The initial kinetic energy KEi of the charge q′ is zero since it starts from rest.
- The initial potential energy PEi can be calculated using the formula for the electric potential energy between point charges.
- The distance from the charge q′ at the origin to each charge q is a.
- The potential energy PE due to one charge q is given by:
PE=14πϵ0q⋅q′r
- Since there are two charges, the total initial potential energy PEi is:
PEi=2⋅14πϵ0q⋅q′a=q⋅q′2πϵ0a
- At infinity, the potential energy PEf is zero because the distance between charges becomes infinitely large.
- The final kinetic energy KEf can be expressed as:
KEf=12mv2
- Here, v is the speed of the charge q′ at infinity.
- According to the conservation of mechanical energy:
KEi+PEi=KEf+PEf
- Substituting the known values:
0+q⋅q′2πϵ0a=12mv2+0
- Rearranging the equation gives:
12mv2=q⋅q′2πϵ0a
- Multiplying both sides by 2:
mv2=q⋅q′πϵ0a
- Dividing by m:
v2=q⋅q′mπϵ0a
- Taking the square root to find v:
v=√q⋅q′mπϵ0a
The speed of charge q′ at infinity is:
v=√q⋅q′mπϵ0a
Similar Questions
Consider two positive point charges, each of magnitude q, placed on the y-axis at the points (0, a) and (0, -a). A positively charged particle of charge q' and mass m is displaced slightly from the origin in the direction of the positive x-axis.
At the origin, charge q' will be in
View SolutionPoint charges +4q,−q and +4q are kept on the x-axis at points x=0,x=a and x=2a respectively, then
View SolutionTwo positive point charges of unequal magnitude are placed at a certain distance apart. A small positive test charge is placed at null point, then
View SolutionTwo point charges each 50μC are fixed on y-axis at y=+4m and y=−4m. Another charged particle having charge −50μC and mass 20g is moving along the positive x-axis. When it is at x=−3m , its speed is 20m/sec. Find the speed of charged particle when it reaches to origin. Also , find distance of charged particle from origin , when its kinetic energy becomes zero.
View SolutionIn the figure, two point charges +qand−q are placed on the x-axis at (+a,0)and(−a,0) respectively. A tiny dipole of dipolem moment (p) is kept at the origin along the y-axis. The torque on the dipole equals

View SolutionA positive point charge Q is bruought mear an isolated metal cube.
View SolutionTwo point charges (Q each are placed at (0,y) and (0-y) A point charge q of the same polarity can move along the x-axis. Then
View SolutionCharges 4Q, q and Q and placed along x-axis at positions x=0,x=1/2 and x=1, respectively. Find the value of q so that force on charge Q is zero
View SolutionA positive charge q is placed in a spherical cavity made in a positively charged sphere. The centres of sphere and cavity are displaced by a small distance →l. Force on charge q is :
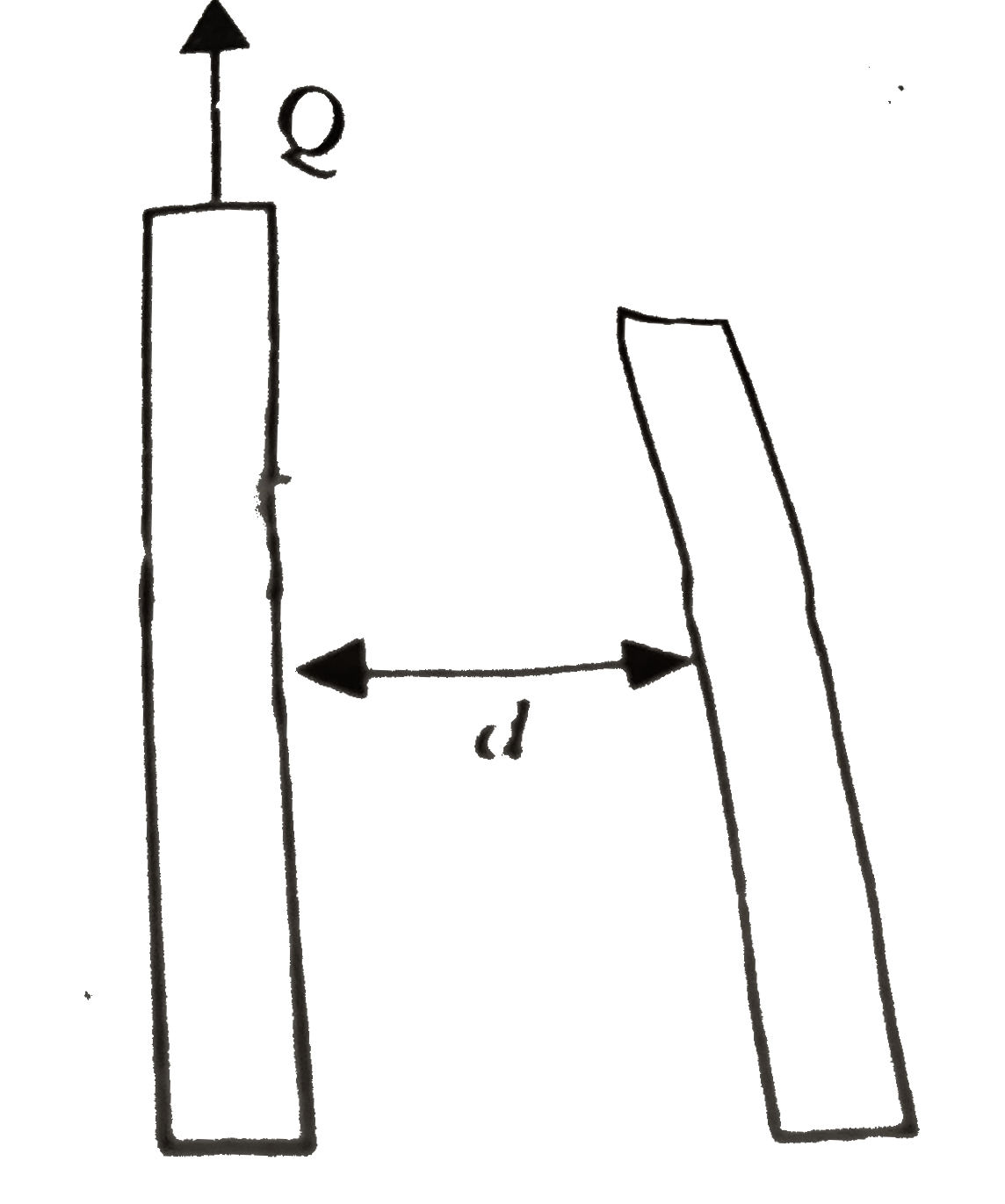
View SolutionTwo parallel conducting plates, each of area A, are separated by a distance d. Now, the left plate is given a positive charge Q. A positive charge q of mass m is released from a point near the left plate. Find the time taken by the charge to reach the right plate.
View Solution
