Which of the following is differentiable in the interval (−1,2)?
∫2xx(logx)2dx
∫2xxsinxxdx
∫2xx1−t+t21+t+t2dt
none of these
The correct Answer is:C
To determine which of the given functions is differentiable in the interval (−1,2), we will analyze each function using the properties of differentiability and the Fundamental Theorem of Calculus.
1. Identify the Functions:
Let's denote the functions provided in the problem as f1(x), f2(x), and f3(x). We need to analyze each of these functions for differentiability in the interval (−1,2).
2. Check the First Function:
- Function: f1(x)=∫2xxlog(t2)dt
- Differentiability: The logarithm function log(t2) is not defined for t≤0. Since t ranges from x to 2x, if x is negative (which it is in the interval (−1,0)), the function is not differentiable in that interval.
- Conclusion: f1(x) is not differentiable in (−1,2).
3. Check the Second Function:
- Function: f2(x)=∫2xxsin(t)dt
- Differentiability: The sine function is defined for all real numbers, so we can differentiate this function using the Fundamental Theorem of Calculus.
- Differentiation:
f′2(x)=sin(2x)⋅2−sin(x)⋅1
- Since both sin(2x) and sin(x) are defined for all x, f2(x) is differentiable in (−1,2).
- Conclusion: f2(x) is differentiable in (−1,2).
4. Check the Third Function:
- Function: f3(x)=∫2x11−t+t21+t+t2dt
- Differentiability: The integrand 1−t+t21+t+t2 is a rational function and is defined for all t (the denominator does not equal zero for real t).
- Differentiation:
f′3(x)=1−(2x)+(2x)21+(2x)+(2x)2⋅2
- This derivative is also defined for all x in (−1,2).
- Conclusion: f3(x) is differentiable in (−1,2).
Final Conclusion:
Among the functions analyzed:
- f1(x) is not differentiable in (−1,2).
- f2(x) is differentiable in (−1,2).
- f3(x) is differentiable in (−1,2).
Thus, the answer to the question "Which of the following is differentiable in the interval (−1,2)?" is f2(x) and f3(x).
Let's denote the functions provided in the problem as f1(x), f2(x), and f3(x). We need to analyze each of these functions for differentiability in the interval (−1,2).
- Function: f1(x)=∫2xxlog(t2)dt
- Differentiability: The logarithm function log(t2) is not defined for t≤0. Since t ranges from x to 2x, if x is negative (which it is in the interval (−1,0)), the function is not differentiable in that interval.
- Conclusion: f1(x) is not differentiable in (−1,2).
- Function: f2(x)=∫2xxsin(t)dt
- Differentiability: The sine function is defined for all real numbers, so we can differentiate this function using the Fundamental Theorem of Calculus.
- Differentiation:
f′2(x)=sin(2x)⋅2−sin(x)⋅1
- Since both sin(2x) and sin(x) are defined for all x, f2(x) is differentiable in (−1,2).
- Conclusion: f2(x) is differentiable in (−1,2).
- Function: f3(x)=∫2x11−t+t21+t+t2dt
- Differentiability: The integrand 1−t+t21+t+t2 is a rational function and is defined for all t (the denominator does not equal zero for real t).
- Differentiation:
f′3(x)=1−(2x)+(2x)21+(2x)+(2x)2⋅2
- This derivative is also defined for all x in (−1,2).
- Conclusion: f3(x) is differentiable in (−1,2).
Among the functions analyzed:
- f1(x) is not differentiable in (−1,2).
- f2(x) is differentiable in (−1,2).
- f3(x) is differentiable in (−1,2).
Similar Questions
Which of the following fiunction is not differentiable at x=1?
View SolutionLet f,g:[−1,2]→R be continuous functions which are twice differentiable on the interval (-1, 2). Let the values of f and g at the points –1, 0 and 2 be as given in the following table : x=−1x=0x=2f(x)360g(x)01−1 In each of the intervals (-1,0) and (0, 2) the function (f – 3g)'' never vanishes. Then the correct statement(s) is(are)
View Solution
Knowledge Check
Which of the following is an example of differentiation ?
ALignocellulosic wall thickenings of tracheidsBLoss of nucleus, vacuolisation and end wall perofrations in sieve tube elementsCElongation, thickening and emptying of sclerenchyma fibersDAll of these
Let f,g:[−1,2]→ be continuous functions which are twice differentiable on the interval (-1,2). Let the values of f and g at the points and 2 be as given in the following table:
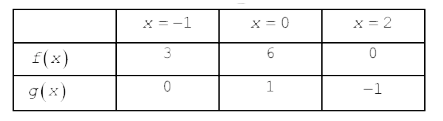
In each of the intervals (-1,0) and (0, 2) the function (f-3g) never vanishes. Then the correct statements(s) is(are) :
View SolutionThe following functions are differentiable on (-1,2)
View SolutionWhich of the following is distributed at regular intervals on the tropomyosin ?
View SolutionWhich of the following differential equations has y=c1ex+c2e−x as the general solution ?
View SolutionIf f(x)=|x+1|(|x|+|x−1|), then at what point(s) is the function not differentiable over the interval [−2,2]?
View SolutionWhich of the following plant flowers repeatedly at intervals?
View SolutionThe number of points at which the function f(x)=(x−|x|)2(1−x+|x|)2 is not differentiable in the interval (−3,4) is ___
View Solution
