A particle travels two and a half revolutions of the circle of radius R in time t. The ratio of the average speed of the particle to the magnitude of the average velocity in this time interval is
π2
5π√2
5π2
π5√2
The correct Answer is:C
To solve the problem, we need to find the ratio of the average speed of a particle to the magnitude of its average velocity after it travels two and a half revolutions around a circle of radius R in time t.
1. Calculate the Total Distance Traveled:
- The distance traveled in one complete revolution of a circle is given by the circumference, which is 2πR.
- For two and a half revolutions, the total distance D is:
D=2.5×(2πR)=5πR
2. Calculate the Average Speed:
- Average speed is defined as the total distance traveled divided by the total time taken. Therefore, the average speed vavg is:
vavg=Dt=5πRt
3. Calculate the Displacement:
- The displacement is the shortest distance from the initial position to the final position. After two and a half revolutions, the particle ends up at a point directly opposite its starting point on the circle.
- The displacement S is equal to the diameter of the circle, which is:
S=2R
4. Calculate the Magnitude of Average Velocity:
- Average velocity is defined as the total displacement divided by the total time taken. Thus, the magnitude of average velocity vavg,mag is:
vavg,mag=St=2Rt
5. Calculate the Ratio of Average Speed to Magnitude of Average Velocity:
- Now, we can find the ratio of average speed to the magnitude of average velocity:
Ratio=vavgvavg,mag=5πRt2Rt=5πR2R
- Simplifying this gives:
Ratio=5π2
Final Answer:
The ratio of the average speed of the particle to the magnitude of the average velocity is:
5π2
- The distance traveled in one complete revolution of a circle is given by the circumference, which is 2πR.
- For two and a half revolutions, the total distance D is:
D=2.5×(2πR)=5πR
- Average speed is defined as the total distance traveled divided by the total time taken. Therefore, the average speed vavg is:
vavg=Dt=5πRt
- The displacement is the shortest distance from the initial position to the final position. After two and a half revolutions, the particle ends up at a point directly opposite its starting point on the circle.
- The displacement S is equal to the diameter of the circle, which is:
S=2R
- Average velocity is defined as the total displacement divided by the total time taken. Thus, the magnitude of average velocity vavg,mag is:
vavg,mag=St=2Rt
- Now, we can find the ratio of average speed to the magnitude of average velocity:
Ratio=vavgvavg,mag=5πRt2Rt=5πR2R
- Simplifying this gives:
Ratio=5π2
The ratio of the average speed of the particle to the magnitude of the average velocity is:
5π2
Similar Questions
A point traversed 3/4 th of the circle of radius R in time t. The magnitude of the average velocity of the particle in this time interval is
View SolutionIf magnitude of average speed and average velocity over a time interval are same, then
View SolutionA particle travels 336 mi in 6 hn, its 1 average velocity is
View SolutionThe magnitude of average velocity is equal to the average speed when a particle moves:-
View SolutionA particle is projected with a speed v and an angle θ to the horizontal. After a time t, the magnitude of the instantaneous velocity is equal to the magnitude of the average velocity from 0 to t. Find t.
View SolutionA particle is moving in a circle of radius R with constant speed. The time period of the particle is T. In a time t=T6
Average velocity of the particle is…..
View SolutionUnder what condition is the magnitude of the average velocity of a particle moving in onbe dimension smaller than the average speed over same time interval?
View SolutionRead each statement below carefully and state with reasons, with it is true or false :
(a) The magnitude of vector is always a scalar.
(b) Each component of a vector is always a scalar.
(c) The total path length is always equal to the magnitude of the displacement vector of a particle.
(d) The average speed of a particle (defined as total path length divided by the time taken to cover the path) is greater or equal to the magnitude of average velocity of the particle over the same interval of time.
(e) three vectors not lying in a plane can never add up to give a null vector.
View SolutionFrom the velocity-time plot shown in Fig. find the distance travelled by the particle during the first 40s. Also find the average velocity during this period.
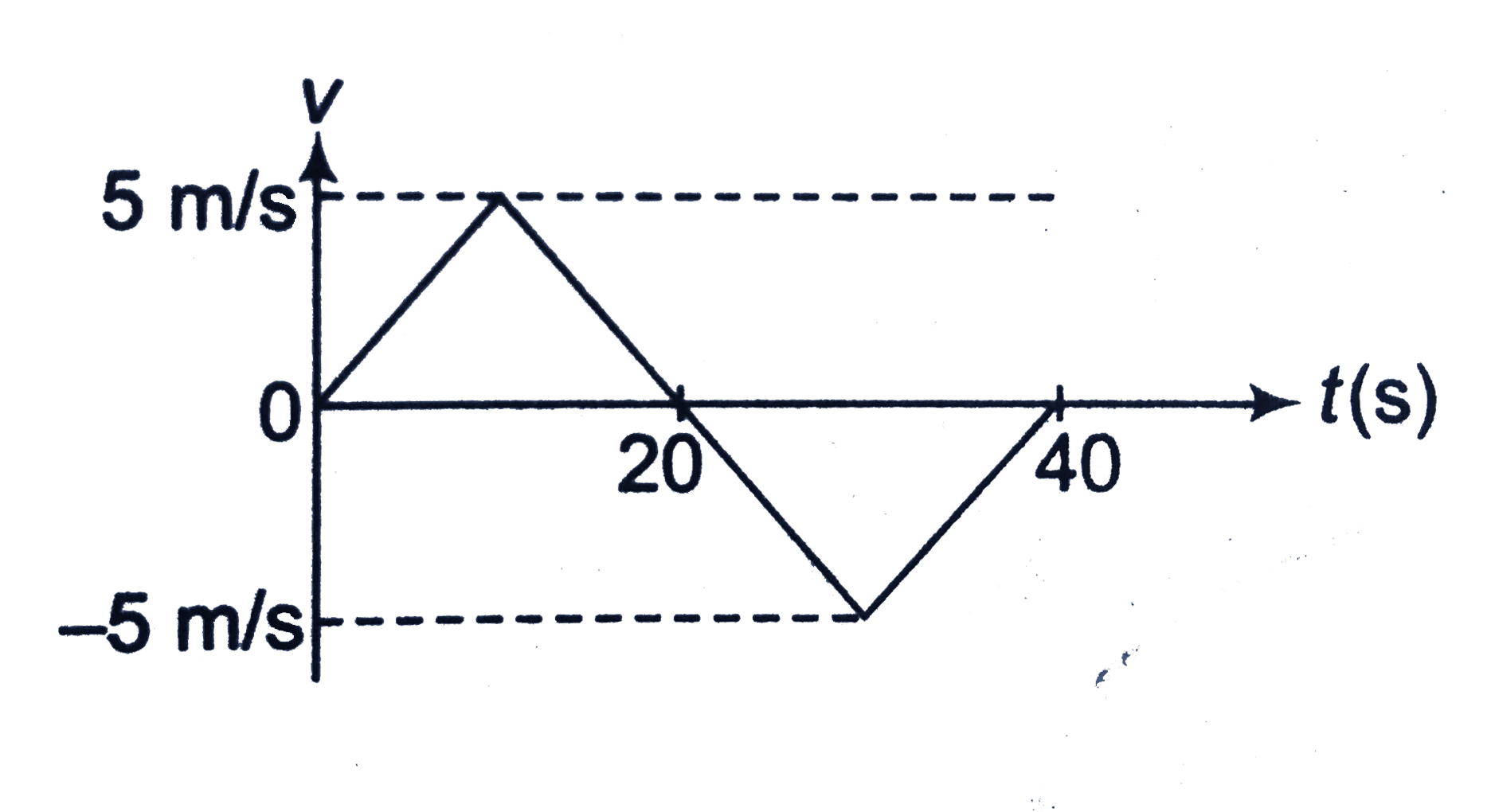
View SolutionA particle is moving with speed v in a circle of radius R. Find the magnitude for average velocity of point
for the half revolution
View Solution
