Two shells are fired from cannon with speed u each, at angles of α of β respectively with the horizontal.The time interval between the shots is T.They collide in mid air after time t from the first shot.Which of the following conditions must be satisfied?
α>β
tcosα=(t−T)cosβ
(t−T)cosα=tcosβ
(usinα)t−(1/2)g(t)2=(usinβ)(t−T)−(1/2)g(t−T)2
The correct Answer is:A,B,D
To solve the problem, we need to analyze the motion of the two shells fired from the cannon. Let's break down the steps to derive the conditions that must be satisfied for the shells to collide in mid-air.
Step 1: Determine the horizontal and vertical components of the velocities
For both shells, the horizontal and vertical components of their velocities can be expressed as follows:
1. First shell (fired at angle α):
- Horizontal component: ux=ucosα
- Vertical component: vy=usinα
2. Second shell (fired at angle β):
- Horizontal component: ux=ucosβ
- Vertical component: vy=usinβ
Step 2: Calculate the horizontal distance traveled by both shells
After time t from the first shot, the horizontal distance traveled by the first shell is:
x1=ucosα⋅t
The second shell is fired after a time interval T, so by the time the first shell has been in the air for t, the second shell has been in the air for t−T. The horizontal distance traveled by the second shell is:
x2=ucosβ⋅(t−T)
Step 3: Set the horizontal distances equal for collision
For the shells to collide, they must be at the same horizontal position:
ucosα⋅t=ucosβ⋅(t−T)
Dividing both sides by u (assuming u≠0):
cosα⋅t=cosβ⋅(t−T)
Step 4: Calculate the vertical distance traveled by both shells
The vertical distance for the first shell after time t is:
y1=usinα⋅t−12gt2
For the second shell, after time t−T:
y2=usinβ⋅(t−T)−12g(t−T)2
Step 5: Set the vertical distances equal for collision
For the shells to collide, their vertical positions must also be equal:
usinα⋅t−12gt2=usinβ⋅(t−T)−12g(t−T)2
Step 6: Analyze the conditions
From the horizontal distance equation, we have:
cosα⋅t=cosβ⋅(t−T)
From the vertical distance equation, we can derive another condition.
Conclusion
The conditions that must be satisfied for the shells to collide are:
1. cosα⋅t=cosβ⋅(t−T)
2. The vertical distance condition derived from the second equation.
For both shells, the horizontal and vertical components of their velocities can be expressed as follows:
- Horizontal component: ux=ucosα
- Vertical component: vy=usinα
- Horizontal component: ux=ucosβ
- Vertical component: vy=usinβ
After time t from the first shot, the horizontal distance traveled by the first shell is:
x1=ucosα⋅t
x2=ucosβ⋅(t−T)
For the shells to collide, they must be at the same horizontal position:
ucosα⋅t=ucosβ⋅(t−T)
Dividing both sides by u (assuming u≠0):
cosα⋅t=cosβ⋅(t−T)
The vertical distance for the first shell after time t is:
y1=usinα⋅t−12gt2
y2=usinβ⋅(t−T)−12g(t−T)2
For the shells to collide, their vertical positions must also be equal:
usinα⋅t−12gt2=usinβ⋅(t−T)−12g(t−T)2
From the horizontal distance equation, we have:
cosα⋅t=cosβ⋅(t−T)
The conditions that must be satisfied for the shells to collide are:
1. cosα⋅t=cosβ⋅(t−T)
2. The vertical distance condition derived from the second equation.
Similar Questions
Two shells are fired from a cannon with a speed u each, at angles of α and β respectively to the horizontal. The time interval between the shots is T. they collide in mid-air after time t from the first shot. Which of the following conditions must be satisfied ?
View SolutionWhat is the average velocity of a particle projected from the ground with speed u at an angle alpha with horizontal over a time interval from beginning till it strikes the ground again?
View Solution
Knowledge Check
Two projectiles are fired from the same point with the same speed at angles of projection 60∘and30∘ respectively. Which one of the following is true?
ATheir range will be the same.BTheir maximum height will be the same.CTheir velocity at the heighest point will be the same.DTheir time of flight will be the same.Two particls are projected in air with speed u at angles θ1andθ2 (both acute) to the horizontal, respectively. If the height reached by the first particle is greater than that of the second, then which one of the following is correct? where T1andT2 are the time of flight.
Aθ1>θ2Bθ1=θ2CT1<T2DT1=T2
A body of mass m is projected with intial speed u at an angle θ with the horizontal. The change in momentum of body after time t is :-
View SolutionSuppose two particles 1and2 are projected in vertical plane simultaneously.
Their angles of projection are 30∘andθ, respectively, with the horizontal. Let they collide after a timet in air. Then
 .
.
View SolutionTwo projectiles are fired from the same point with the same speed at angles of projection 60∘(B)and30∘(A) respectively. Which one of the following is true?
View Solution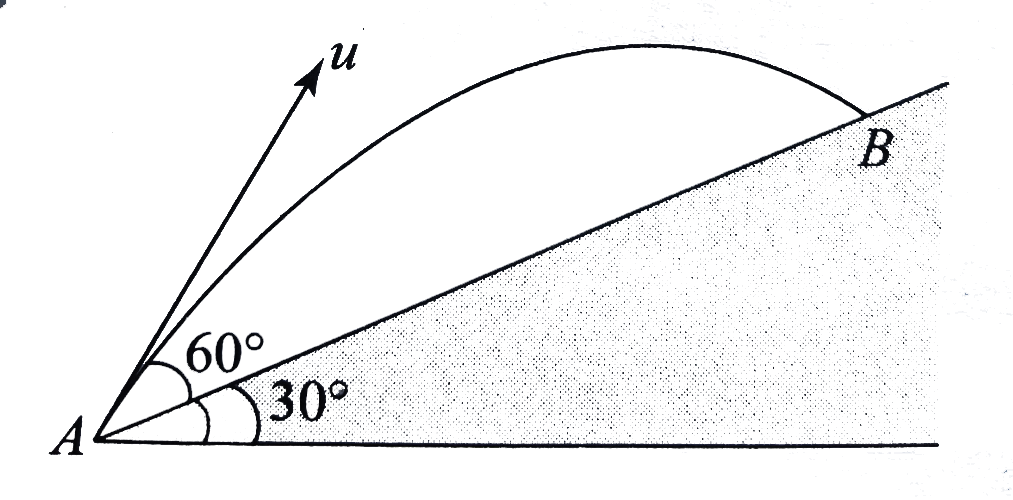
A stone is projected from point A with speed u making an angle 60∘ with horizontal as shown. The fixed inclined suface makes an angle 30∘ with horizontal. The stone lands at B after time t. Then the distance AB is equal to
View Solution
A stone is projected from point A with speed u making an angle 60∘ with horizontal as shown. The fixed inclined suface makes an angle 30∘ with horizontal. The stone lands at B after time t. Then the distance AB is equal to
View SolutionA shell is fired from a cannon with a velocity V at an angle θ with the horizontal direction. A the highest point i its path, it explodes into two pieces of equal masses. One of the pieces retraces its path to the cannon. The speed of the other piece immediately after the explosion is
View Solution
