Question

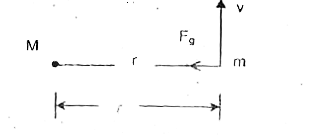
Two massive particles of masses $M$ & $m$ $(M>m)$ are separated by a distance $l$. They rotate with equal angular velocity under their gravitational attraction. The linear speed of the particle of mass $m$ is

Options

A. `sqrt((GMm)/((M+m)l))`
B. `sqrt((GM^(2))/((M+m)l))`
C. `sqrt((Gm)/(l))`
D. `sqrt((Gm^(2))/((M+m)l))`

Answer

B

Explanation

The system rotates about the centre of mass. The gravitational force acting on the particle `m` accelerates it towards the centre of the circular path, which has the radius
`R=(Ml)/(M+m)`
`impliesF=(mv^(2))/(r )implies(GMm)/(l^(2))=(mv^(2))/((Ml)/(M+m))`
`impliesv=sqrt((GM^(2))/((M+m)l))`