A rigid rod of mass m & length l is pivoted at one of its ends. If it is released from its horizontal position, find the speed of the centre of mass of the rod when it becomes vertical.
To solve the problem of finding the speed of the center of mass of a rigid rod of mass m and length l when it becomes vertical after being released from a horizontal position, we can follow these steps:
Step 1: Understand the System
The rod is pivoted at one end and released from a horizontal position. The center of mass of the rod is located at a distance of l2 from the pivot.
Step 2: Calculate the Work Done by Gravity
When the rod falls from the horizontal to the vertical position, the center of mass drops a vertical distance of l2. The work done by gravity can be calculated as:
W=Force×Distance=mg⋅l2
where g is the acceleration due to gravity.
Step 3: Relate Work Done to Kinetic Energy
The work-energy theorem states that the work done by the forces acting on an object is equal to the change in kinetic energy of that object. Initially, the rod is at rest, so its initial kinetic energy is zero. When the rod becomes vertical, it has rotational kinetic energy given by:
KE=12Iω2
where I is the moment of inertia of the rod about the pivot point.
Step 4: Calculate the Moment of Inertia
For a rod pivoted at one end, the moment of inertia I is given by:
I=13ml2
Step 5: Set Up the Equation
From the work-energy principle, we can set up the equation:
mg⋅l2=12Iω2
Substituting I into the equation:
mg⋅l2=12(13ml2)ω2
Step 6: Simplify the Equation
Cancel m from both sides (assuming m≠0):
g⋅l2=16l2ω2
Multiplying both sides by 6:
3gl=l2ω2
Dividing both sides by l (assuming l≠0):
3g=lω2
Thus, we can express ω2 as:
ω2=3gl
Step 7: Find the Speed of the Center of Mass
The speed v of the center of mass is related to the angular speed ω by the formula:
v=ω⋅r
where r is the distance from the pivot to the center of mass, which is l2:
v=ω⋅l2
Substituting ω from the previous step:
v=(√3gl)⋅l2
This simplifies to:
v=l2√3gl=√3gl2
Final Answer
The speed of the center of mass of the rod when it becomes vertical is:
v=√3gl2
---
The rod is pivoted at one end and released from a horizontal position. The center of mass of the rod is located at a distance of l2 from the pivot.
When the rod falls from the horizontal to the vertical position, the center of mass drops a vertical distance of l2. The work done by gravity can be calculated as:
W=Force×Distance=mg⋅l2
where g is the acceleration due to gravity.
The work-energy theorem states that the work done by the forces acting on an object is equal to the change in kinetic energy of that object. Initially, the rod is at rest, so its initial kinetic energy is zero. When the rod becomes vertical, it has rotational kinetic energy given by:
KE=12Iω2
where I is the moment of inertia of the rod about the pivot point.
For a rod pivoted at one end, the moment of inertia I is given by:
I=13ml2
From the work-energy principle, we can set up the equation:
mg⋅l2=12Iω2
Substituting I into the equation:
mg⋅l2=12(13ml2)ω2
Cancel m from both sides (assuming m≠0):
g⋅l2=16l2ω2
Multiplying both sides by 6:
3gl=l2ω2
Dividing both sides by l (assuming l≠0):
3g=lω2
Thus, we can express ω2 as:
ω2=3gl
The speed v of the center of mass is related to the angular speed ω by the formula:
v=ω⋅r
where r is the distance from the pivot to the center of mass, which is l2:
v=ω⋅l2
Substituting ω from the previous step:
v=(√3gl)⋅l2
This simplifies to:
v=l2√3gl=√3gl2
The speed of the center of mass of the rod when it becomes vertical is:
v=√3gl2
Similar Questions
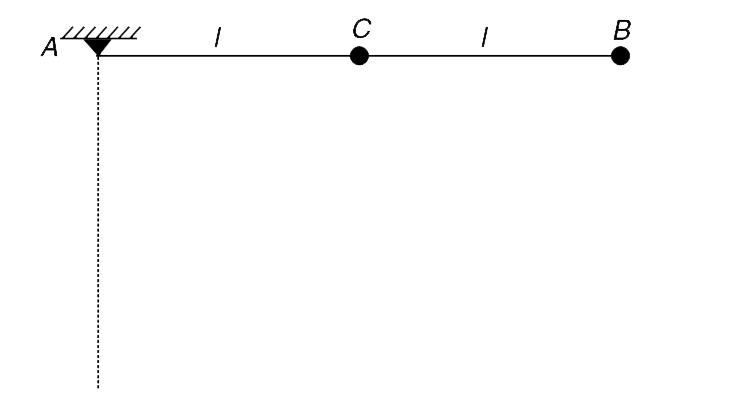
AB is a mass less rigid rod of length 2l. It is free to rotate in vertical plane about a horizontal axis passing through its end A. Equal point masses (m each) are stuck at the centre C and end Bof the rod. The rod is released from horizontal position. Write the tension in the rod when it becomes vertical.
View SolutionA uniform rod smoothly pivoted at one of its ends is released from rest. If it swings in vertical plane, the maximum speed of the end P of the rod is.
 .
.
View SolutionA rod of length l is pivoted about a horizontal , frictionless pin through one end. The rod is released from ret in a vertical position. Find the velocity of the CM of the rod when the rod is inclined at an angle θ from the vertical.

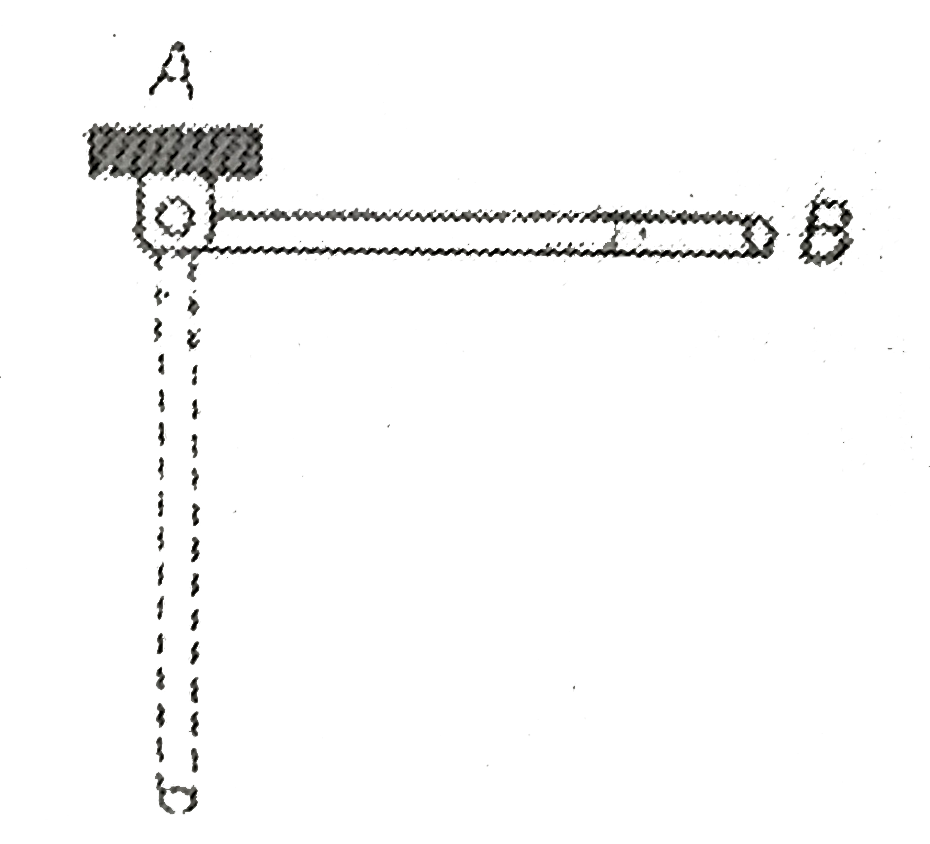
View SolutionOne end of a uniform rod of length l and mass m is hinged at A. It is released from the rest from horizontal position AB as shown in figure. The force exerted by the rod on the hinge when it becomes verticle is
View SolutionA rod of length L is hinged from one end. It is brought to a horizontal position and released. The angular velocity of the rod, when it is in vertical position, is
View SolutionTwo particles each of mass m are attached at end points of a massless rod AB of length l. Rod is hinged at point C as shown. Rod is released from rest from horizontal position. At the instant when rod reaches its vertical position as shown, which of the following is / are correct:
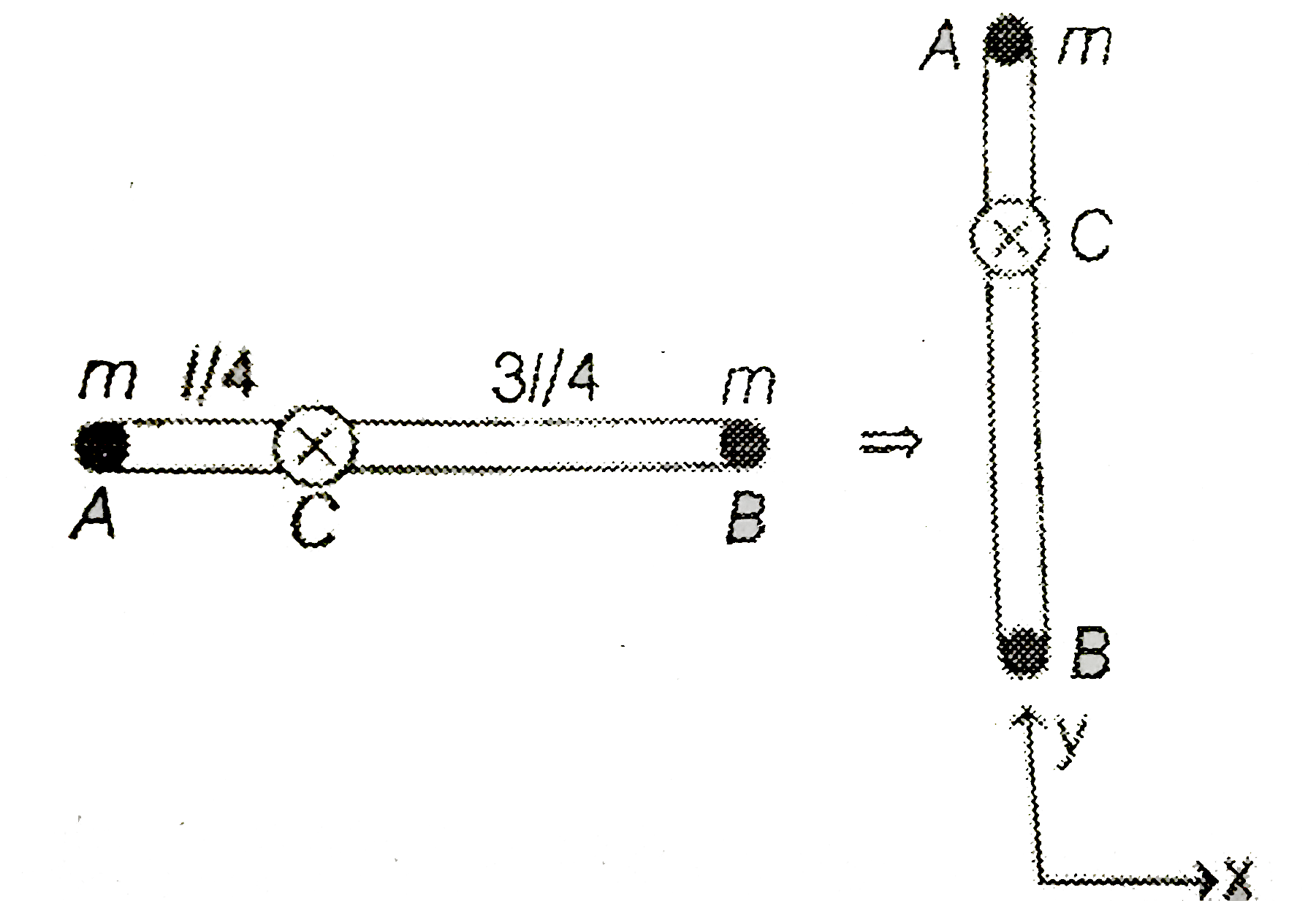
View SolutionA light rigid rod of mass M, and length L is pivoted at one of its end. The rod can swing freely in the vertical plane through a horizontal axis passing through the pivot. Show that the time period of the rod about the pivot is T=π√2L3g
View SolutionA rod of length L is hinged from one end. It is brought to the horizontal position and released. The angular velocity of the rod when it is in the vertical position is √Kg/L. Then find the value of K.
View SolutionA uniform rod of mass m, hinged at its upper end, is released from rest from a horizontal position. When it passes through the vertical position, the force on the hinge is
View SolutionA uniform thin rod of length l and mass m is hinged at a distance l/4 from one of the end and released from horizontal position as shown in Fig. The angular velocity of the rod as it passes the vertical position is

View Solution
