A small ball strikes at one end of a stationary uniform frictionless rod of mass m and length l which is free to rotate, in gravity-free space. The impact elastic. Instantaneous axis of rotation of the rod will pass through
its center of mass
the center of mass of rod plus ball.
the point of impact of the ball on the rod
the point which is at a distance 2l/3 from the striking end.
The correct Answer is:D
To solve the problem of determining the instantaneous axis of rotation of a uniform frictionless rod after being struck by a small ball, we can follow these steps:
1. Identify the System:
- We have a uniform rod of mass m and length l that is initially stationary and free to rotate in gravity-free space. A small ball strikes one end of the rod.
2. Understand the Impact:
- The impact is elastic, meaning both momentum and kinetic energy are conserved. The ball strikes the rod at one end, imparting a velocity to the rod.
3. Define the Variables:
- Let v be the velocity of the ball just before the impact.
- Let ω be the angular velocity of the rod after the impact.
- The center of mass of the rod is at a distance of l2 from either end.
4. Apply Conservation of Linear Momentum:
- The change in momentum due to the impact can be expressed as:
mv=I⋅ω
- Here, I is the moment of inertia of the rod about its center of mass, which is given by I=13ml2 when considering rotation about the center.
5. Calculate the Angular Impact:
- The angular impact can be expressed as:
Angular impact=Force×Distance
- The distance from the center of mass to the end of the rod is l2.
6. Determine the Instantaneous Axis of Rotation:
- Let x0 be the distance from the end of the rod where the ball strikes to the instantaneous axis of rotation. The point P at x0 is stationary after the impact.
- The velocity of point P can be expressed as:
v=ω(x0−l2)
7. Set Up the Equation:
- Since point P is stationary, we have:
0=ω(x0−l2)
- This implies:
x0=l2
8. Solve for x0:
- From the conservation equations and the geometry of the problem, we find:
x0=2l3
- Thus, the instantaneous axis of rotation is located at a distance of 2l3 from the end where the impulse is applied.
Final Answer:
The instantaneous axis of rotation of the rod will pass through a point located at a distance of 2l3 from the end where the ball strikes.
- We have a uniform rod of mass m and length l that is initially stationary and free to rotate in gravity-free space. A small ball strikes one end of the rod.
- The impact is elastic, meaning both momentum and kinetic energy are conserved. The ball strikes the rod at one end, imparting a velocity to the rod.
- Let v be the velocity of the ball just before the impact.
- Let ω be the angular velocity of the rod after the impact.
- The center of mass of the rod is at a distance of l2 from either end.
- The change in momentum due to the impact can be expressed as:
mv=I⋅ω
- Here, I is the moment of inertia of the rod about its center of mass, which is given by I=13ml2 when considering rotation about the center.
- The angular impact can be expressed as:
Angular impact=Force×Distance
- The distance from the center of mass to the end of the rod is l2.
- Let x0 be the distance from the end of the rod where the ball strikes to the instantaneous axis of rotation. The point P at x0 is stationary after the impact.
- The velocity of point P can be expressed as:
v=ω(x0−l2)
- Since point P is stationary, we have:
0=ω(x0−l2)
- This implies:
x0=l2
- From the conservation equations and the geometry of the problem, we find:
x0=2l3
- Thus, the instantaneous axis of rotation is located at a distance of 2l3 from the end where the impulse is applied.
The instantaneous axis of rotation of the rod will pass through a point located at a distance of 2l3 from the end where the ball strikes.
Similar Questions
An impulse J=mv at one end of a stationary uniform frictionless rod of mass m and length l which is free to rotate in a gravity-free space. The impact is elastic. Instantaneous axis of rotation of the rod will pass through
View SolutionA thin unifrom rod of mass m and length l is free to rotate about its upper end When it is at rest. It receives an impulse J as its lowest point, normal to its length immediately after impact.
View SolutionFind the moment of inertia A of a spherical ball of mass m and radius r attached at the end of a straight rod of mass M and length l, if this system is free to rotate about an axis passing through the end of the rod (end of the rod opposite to sphere).

View SolutionA thin uniform wire of mass m and length l is bent into to shape of a semicircle. The moment of inertia of that wire about an axis passing through its free ends is
View SolutionA uniform thin rod of length l is suspended from one of its ends and is rotated at f rotations per second. The rotational kinetic energy of the rod will be
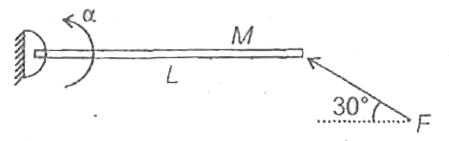
View SolutionThe thin rod shown below has mases M and length L. A force F acts at one end as shown and the rod is free to rotate about the other end in horizontal plane. Initial angular acceleration of the rod is :
View SolutionA thin uniform rod of mass M and length L. Find the radius of gyration for rotation about an axis passing through a point at a distance ofL4 from centre and perpendicular to rod.
View SolutionA thin uniform rod of mass M and length L. Find the radius of gyration for rotation about an axis passing through a point at a distance ofL4 from centre and perpendicular to rod.
View SolutionA L shaped rod of mass M is free to rotate in a vertical plane about axis A A as shown in figure. Maximum angular acceleration of rod is

View SolutionCalculate the moment of inertia of a rod of mass M, and length l about an axis perpendicular to it passing through one of its ends.
View Solution
