Three resistors and are to be combined to form an electrical circuit as shown in the figure. It is found that when are put respectively in positions A, B and C, the effective resistance of the circuit is 70 When are put respectively in position A, B and C the effective resistance is 35 and when are respectively put in the position A, B and C, the effective resistance is
If andare put in series, the effective resistance will be
The correct Answer is:C
To solve the problem, we need to find the effective resistance when three resistors R1,R2, and R3 are connected in series. We are given three different configurations of these resistors and their corresponding effective resistances. Let's denote the effective resistances in the three configurations as follows:
1. Configuration 1: R1,R2,R3 in positions A, B, C results in Reff1=70Ω
2. Configuration 2: R2,R3,R1 in positions A, B, C results in Reff2=35Ω
3. Configuration 3: R3,R1,R2 in positions A, B, C results in Reff3=42Ω
Let's denote the resistances as follows:
- R1=x
- R2=y
- R3=z
From the configurations, we can set up the following equations based on the rules for resistors in series and parallel:
1. For Configuration 1:
Reff1=R1+11R2+1R3=70
This simplifies to:
70=x+11y+1z⟹70=x+yzy+z
2. For Configuration 2:
Reff2=R2+11R3+1R1=35
This simplifies to:
35=y+11z+1x⟹35=y+zxz+x
3. For Configuration 3:
Reff3=R3+11R1+1R2=42
This simplifies to:
42=z+11x+1y⟹42=z+xyx+y
Now we have three equations:
1. 70=x+yzy+z
2. 35=y+zxz+x
3. 42=z+xyx+y
Next, we will solve these equations simultaneously to find the values of x,y, and z.
Step 1: Solve the equations
From the first equation, we can express yzy+z:
yzy+z=70−x(1)
From the second equation:
zxz+x=35−y(2)
From the third equation:
xyx+y=42−z(3)
Step 2: Substitute and solve for one variable
We can express z in terms of x and y using equation (3):
z=42−xyx+y
Step 3: Substitute z back into equations (1) and (2)
Substituting z into equations (1) and (2) will give us two equations in terms of x and y. This will allow us to solve for x and y.
Step 4: Solve for R1,R2,R3
After solving the equations, we will find the values of R1,R2, and R3.
Step 5: Calculate the effective resistance in series
Once we have the values of R1,R2, and R3, the effective resistance Reff when they are connected in series is given by:
Reff=R1+R2+R3
Final Calculation
After substituting the values of R1,R2, and R3 into the equation for Reff, we will arrive at the final answer.
2. Configuration 2: R2,R3,R1 in positions A, B, C results in Reff2=35Ω
3. Configuration 3: R3,R1,R2 in positions A, B, C results in Reff3=42Ω
- R1=x
- R2=y
- R3=z
Reff1=R1+11R2+1R3=70
This simplifies to:
70=x+11y+1z⟹70=x+yzy+z
Reff2=R2+11R3+1R1=35
This simplifies to:
35=y+11z+1x⟹35=y+zxz+x
Reff3=R3+11R1+1R2=42
This simplifies to:
42=z+11x+1y⟹42=z+xyx+y
1. 70=x+yzy+z
2. 35=y+zxz+x
3. 42=z+xyx+y
yzy+z=70−x(1)
zxz+x=35−y(2)
xyx+y=42−z(3)
z=42−xyx+y
Reff=R1+R2+R3
Similar Questions
Three resistors R1,R2 and R3 are to be combined to form an electrical circuit as shown in the figure. It is found that when R1R2andR3 are put respectively in positions A, B and C, the effective resistance of the circuit is 70 ΩWhen R2,R3andR1 are put respectively in position A, B and C the effective resistance is 35Ω and when R3,R1andR2 are respectively put in the position A, B and C, the effective resistance is 42Ω
If R1,R2andR3 are all connected in parallel, the effective resistance will be
View SolutionThree resistors R1,R2 and R3 are to be combined to form an electrical circuit as shown in the figure. It is found that when R1R2andR3 are put respectively in positions A, B and C, the effective resistance of the circuit is 70 ΩWhen R2,R3andR1 are put respectively in position A, B and C the effective resistance is 35Ω and when R3,R1andR2 are respectively put in the position A, B and C, the effective resistance is 42Ω
The resistanceR1:R2:R3are in the ratio
View Solution
Knowledge Check
In the shown in the given figure, the resistances R1andR2 are respectively
 A14Ωand40ΩB40Ωand44ΩC40Ωand30ΩD14Ωand30Ω
A14Ωand40ΩB40Ωand44ΩC40Ωand30ΩD14Ωand30Ω
In the given circuit, if I1andI2 be the current in resistances R1andR2, respectively, then
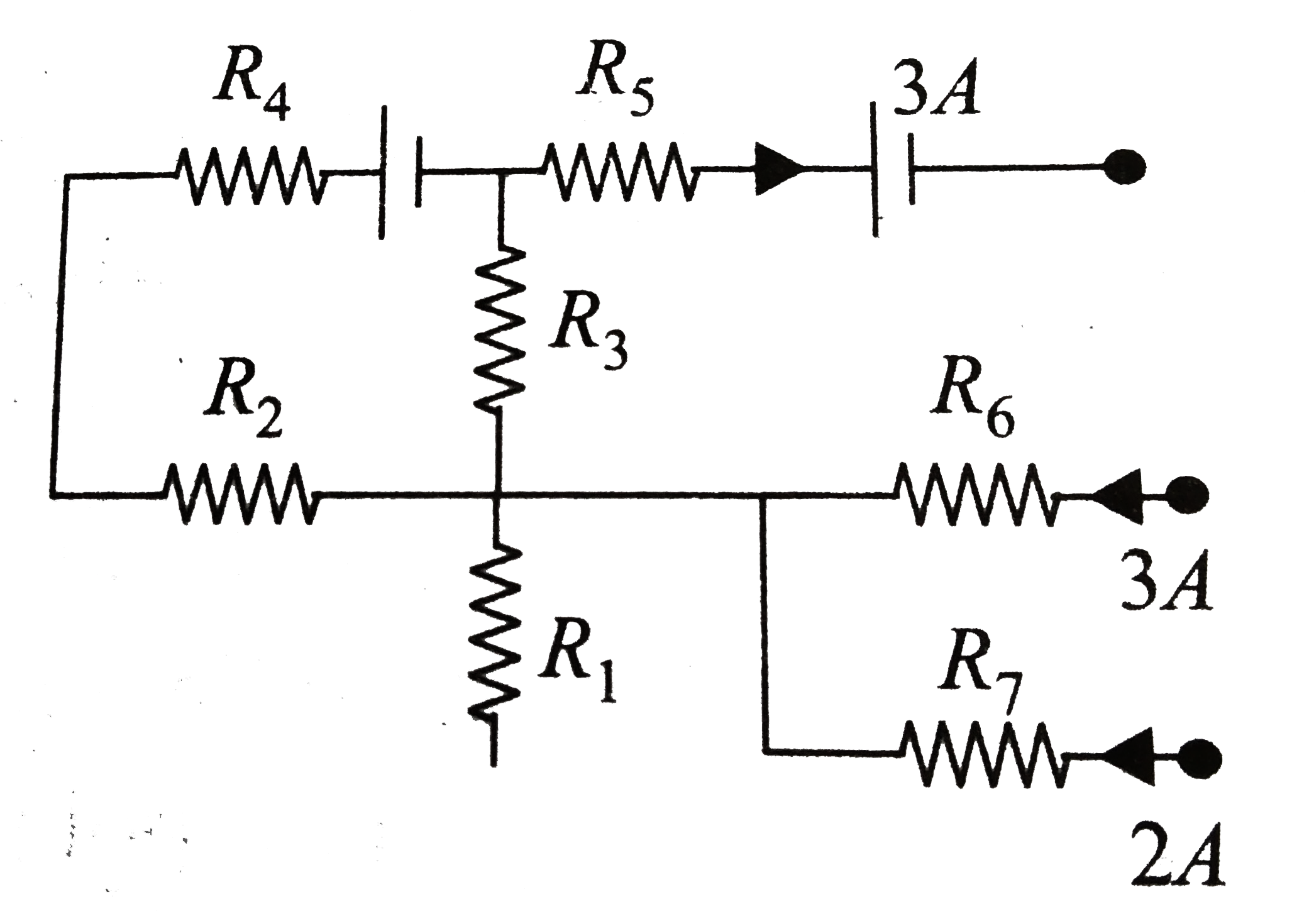 .
.
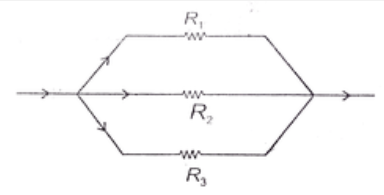
View SolutionIn the situation shown, resistance R1,R2 and R3 are in the ratio 3:2:1 , then
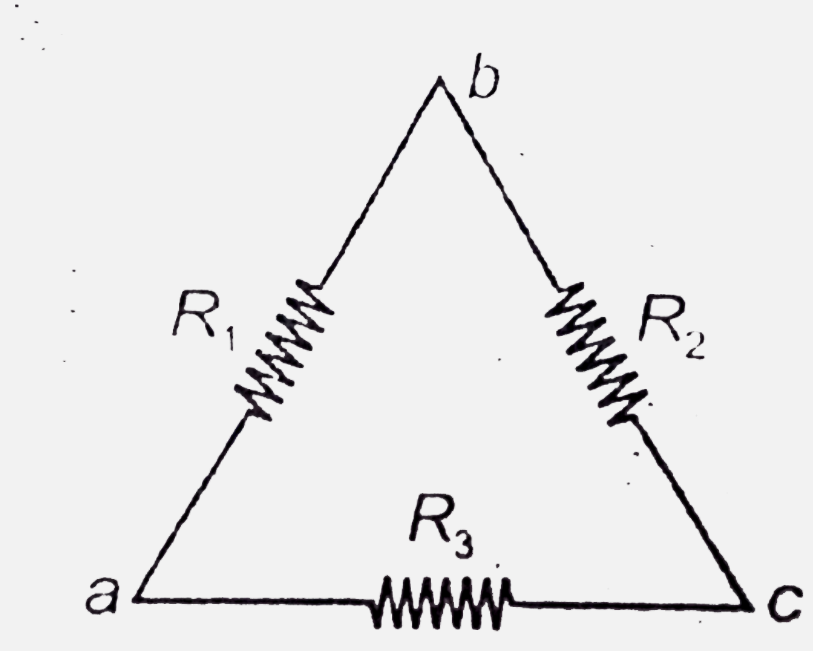
View SolutionConsider the circuit shown in the figure. Equivalent resistance between terminals ab,ac,c equals 30Ω,60Ω and 70Ω respectively. Find the respectively . Find the resistance R1,R2and R3
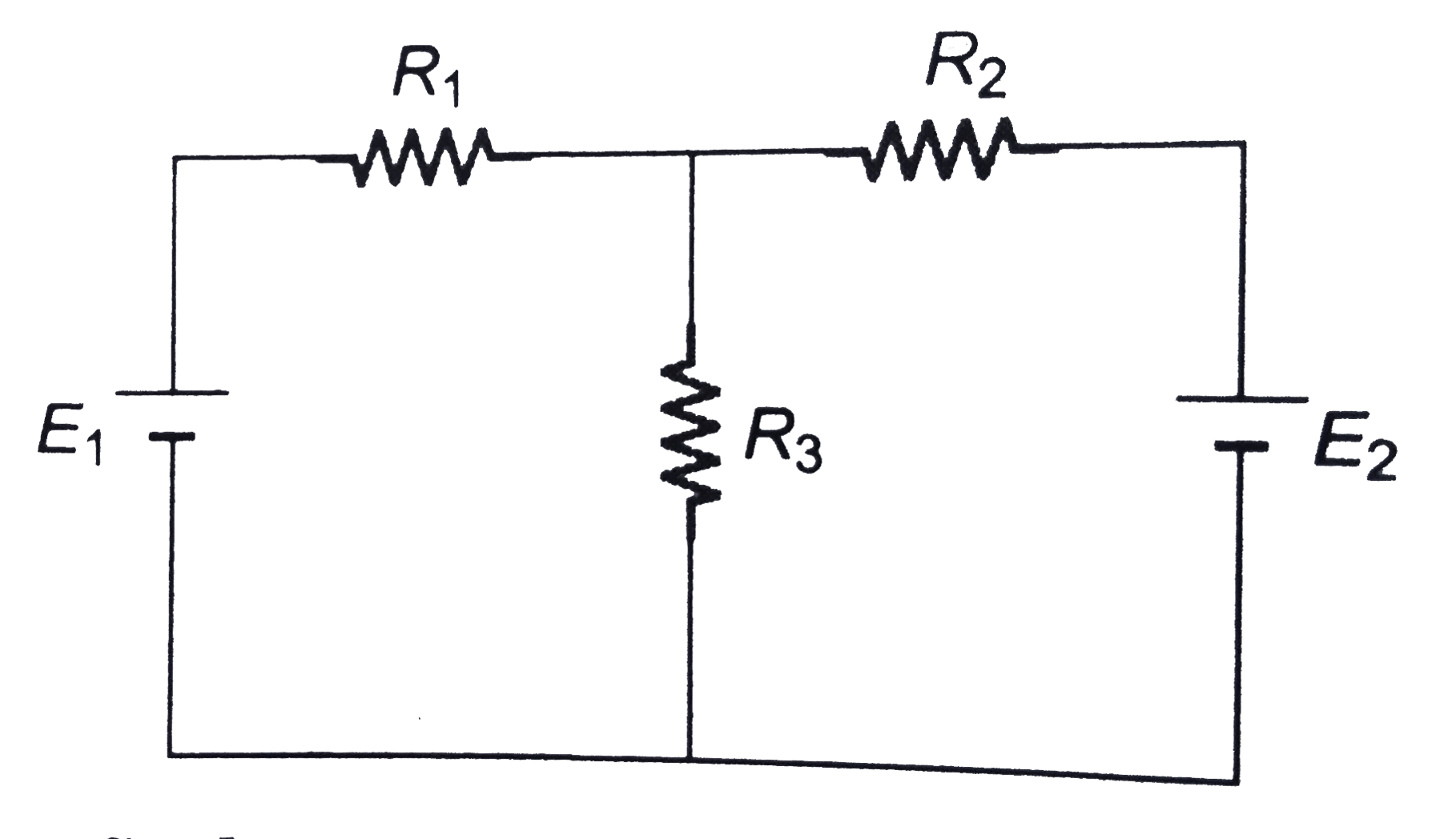
View SolutionIn the circuit shown in figure E1=7V,E2=1V,R1=2Ω,R2=2Ω and R3=3Ω respectively. Find the power supplied by the two batteries.
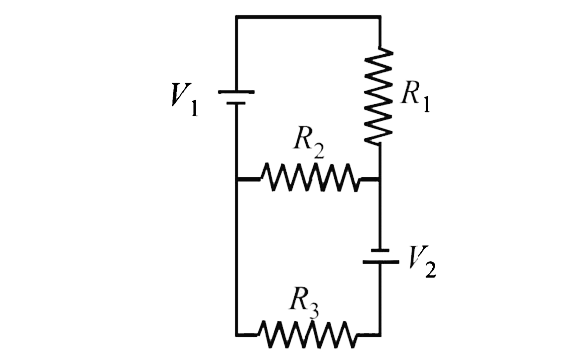
View SolutionTwo ideal batteries of emf V1andV2 and three resistances R1,R2andR3 are connected as shown in the figure. The current in resistance R2 would be zero if
View SolutionFigure shows three resistor configurations R1,R2 and R3 connected to 3 V battery .

If the powers dissipated by the configuration R1,R2 and R3 are P1,P2 and P3, respectively, then
View SolutionFigure shows three resistor configurations R1,R2 and R3 connected to 3 V battery .

If the powers dissipated by the configuration R1,R2 and R3 are P1,P2 and P3, respectively, then
View Solution
