Three equal resistors connected in series across a source of e.m.f. together dissipate 10 W of power. What should be the power dissipated if the same resistors are connected in parallel across the same source of e.m.f.
9 W
90 W
10 W
100 W
The correct Answer is:B
To solve the problem, we will follow these steps:
Step 1: Understand the series connection of resistors
When three equal resistors (let's denote each as R) are connected in series, the total or equivalent resistance (R_eq) is given by:
Req=R1+R2+R3=R+R+R=3R
Step 2: Relate power to resistance in series
The power dissipated (P) in a circuit can be expressed using the formula:
P=V2Req
Given that the power dissipated in the series connection is 10 W, we can write:
10=V23R
Step 3: Rearranging the equation to find V²/R
From the equation above, we can rearrange it to find:
V2=10×3R=30R
Thus, we have:
V2R=30
Step 4: Understand the parallel connection of resistors
When the same three resistors are connected in parallel, the equivalent resistance (R_eq_parallel) is given by:
1Req=1R+1R+1R=3R
Thus, the equivalent resistance for the parallel connection is:
Req=R3
Step 5: Calculate power in the parallel connection
Using the power formula again for the parallel connection:
Pparallel=V2Req
Substituting Req=R3:
Pparallel=V2R3=3V2R
Step 6: Substitute the value of V²/R
From Step 3, we know that V2R=30. Therefore:
Pparallel=3×30=90 W
Conclusion
The power dissipated when the resistors are connected in parallel across the same source of e.m.f. is:
90 W
---
When three equal resistors (let's denote each as R) are connected in series, the total or equivalent resistance (R_eq) is given by:
Req=R1+R2+R3=R+R+R=3R
The power dissipated (P) in a circuit can be expressed using the formula:
P=V2Req
Given that the power dissipated in the series connection is 10 W, we can write:
10=V23R
From the equation above, we can rearrange it to find:
V2=10×3R=30R
Thus, we have:
V2R=30
When the same three resistors are connected in parallel, the equivalent resistance (R_eq_parallel) is given by:
1Req=1R+1R+1R=3R
Thus, the equivalent resistance for the parallel connection is:
Req=R3
Using the power formula again for the parallel connection:
Pparallel=V2Req
Substituting Req=R3:
Pparallel=V2R3=3V2R
From Step 3, we know that V2R=30. Therefore:
Pparallel=3×30=90 W
The power dissipated when the resistors are connected in parallel across the same source of e.m.f. is:
90 W
Similar Questions
Three equal resistors connected in series across a source of emf together dissipate 10W of power. What would be the power dissipated if te same resistors are connected in parallel across the same source of emf?
View SolutionThree equal resistor connected in series across a source of enf together dissipate 10Wa. If the same resistors aer connected in parallel across the same emf, then the power dissipated will be
View Solution
Knowledge Check
Power dissipated in an L−C−R series circuit connected to an AC source of emf ε is
Aε2√R2+(ωL−1ωC)2RBε2[R2+(ωL−1ωC)2]RCε2R√R2+(ωL−1ωC)2Dε2R[R2+(ωL−1ωC)2]
Three equal resistances connected is series across a source of e.m.f consume 20 watt. If the same resistor are connected in parallel across the same source of e.m.f., what would be the power dissipated ?
View SolutionThree identical resistors are connected in series . When a certain potential difference is applied across the combination , the total power would be dissipated is 27W. How many times the power would be dissipated if the three resistors were connected in parallel across the same potential difference ?
View SolutionFour equal resistance dissipated 5 W of power together when connected in series to a battery of negligible internal resistance . The total power dissipated in these resistance when connected in parallel across the same battery would be .
View SolutionWhen an a.c source is connected across a resistor
View Solution3 identical bulbs are connected in series and these together dissipate a power P . If now the bulbs are connected in parallel, then the power dissipated will be
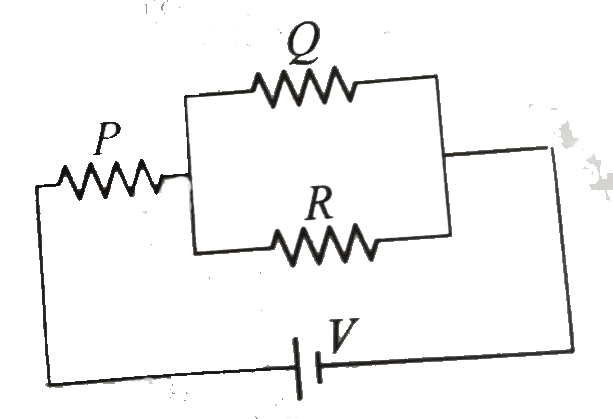
View SolutionResistors P,Q,andR in the circuit have equal resistances. If the battery is supplying a total power of 12W , what is the power dissipated as heat in resistors R?
View SolutionWhat is the value of power factor for a pure resistor connected to an alternating current source?
View Solution
