In case of induced electric field due to a time-dependent magnetic field in a cylindrical region, which of the statement is correct?
It obeys the Gauss' law for electric flux.
The work done by induced electric field while moving a charged particle in a closed loop is zero.
The induced electric field and time-varying magnetic field are parallel to each other at any instant of time.
Electrostatic potential can be defined for the induced electric field.
The correct Answer is:A
To solve the question regarding the induced electric field due to a time-dependent magnetic field in a cylindrical region, we will analyze the statements provided and determine which one is correct.
1. Understanding Induced Electric Field:
- An induced electric field is generated in a region where there is a time-varying magnetic field. According to Faraday's law of electromagnetic induction, a changing magnetic field induces an electric field.
2. Statement Analysis:
- Statement A: "It obeys Gauss's law of electric flux."
- This statement is correct. The induced electric field does obey Gauss's law, which states that the electric flux through a closed surface is proportional to the charge enclosed. The induced electric field can be analyzed using the integral form of Gauss's law.
- Statement B: "The work done by the induced electric field while moving a charged particle in a closed loop is zero."
- This statement is incorrect. The work done by an electric field in moving a charge around a closed loop is not zero if the electric field is induced. The induced electric field is non-conservative, meaning that the work done depends on the path taken.
- Statement C: "The induced electric field and time-varying magnetic field are parallel to each other at any instant of time."
- This statement is also incorrect. The induced electric field is perpendicular to the magnetic field lines. According to the right-hand rule, the direction of the induced electric field is tangential to the loop around which the magnetic field is changing.
- Statement D: "Electrostatic potential can be defined for induced electric field."
- This statement is incorrect. Since the induced electric field forms closed loops, it is non-conservative. Electrostatic potential is defined only for conservative fields, where the work done is path-independent.
3. Conclusion:
- After analyzing all the statements, we find that only Statement A is correct. The induced electric field obeys Gauss's law of electric flux.
Final Answer:
The correct statement is A: "It obeys Gauss's law of electric flux."
- An induced electric field is generated in a region where there is a time-varying magnetic field. According to Faraday's law of electromagnetic induction, a changing magnetic field induces an electric field.
- Statement A: "It obeys Gauss's law of electric flux."
- This statement is correct. The induced electric field does obey Gauss's law, which states that the electric flux through a closed surface is proportional to the charge enclosed. The induced electric field can be analyzed using the integral form of Gauss's law.
- Statement B: "The work done by the induced electric field while moving a charged particle in a closed loop is zero."
- This statement is incorrect. The work done by an electric field in moving a charge around a closed loop is not zero if the electric field is induced. The induced electric field is non-conservative, meaning that the work done depends on the path taken.
- Statement C: "The induced electric field and time-varying magnetic field are parallel to each other at any instant of time."
- This statement is also incorrect. The induced electric field is perpendicular to the magnetic field lines. According to the right-hand rule, the direction of the induced electric field is tangential to the loop around which the magnetic field is changing.
- Statement D: "Electrostatic potential can be defined for induced electric field."
- This statement is incorrect. Since the induced electric field forms closed loops, it is non-conservative. Electrostatic potential is defined only for conservative fields, where the work done is path-independent.
- After analyzing all the statements, we find that only Statement A is correct. The induced electric field obeys Gauss's law of electric flux.
The correct statement is A: "It obeys Gauss's law of electric flux."
Similar Questions
Electric Field due to Dipole
View SolutionElectric field induced by changing magnetic fields are
View SolutionA particle moves in a gravity free space where an electric field of strength E and a magnetic field of induction B exit. Which of the following statement is are correct?
View SolutionThe spatial distribution of the electric field due to charges (A,B) is shown in figure. Which of the following statements is correct?
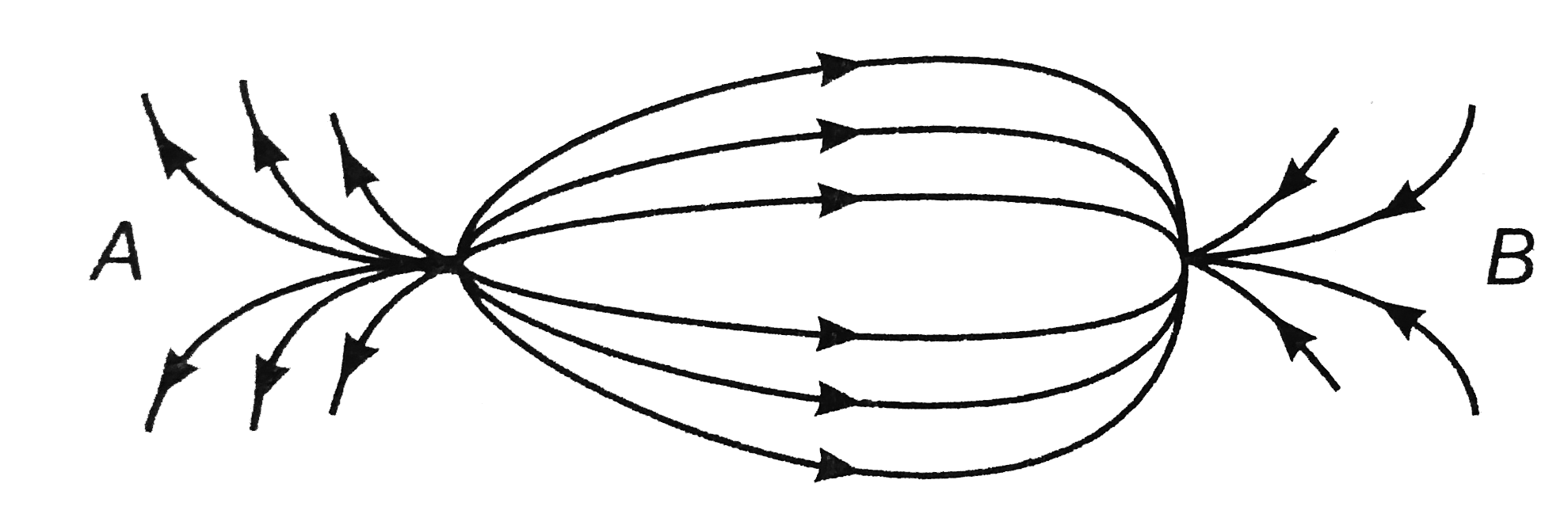
View SolutionCan a photon be deflected by an electric field ? By a magnetic field ?
View SolutionFigure shows electric field lines in which an electric dipole p is placed as shown. Which of the following statements is correct ?
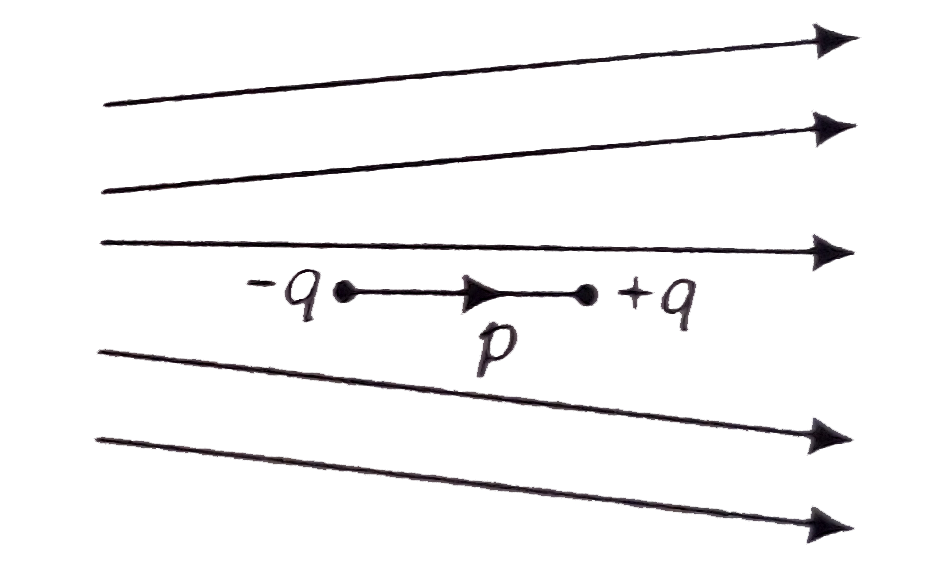
View SolutionFigure shows electric field lines in which an electric dipole p is placed as shown. Which of the following statements is correct ?

View SolutionFigure shows electric field lines in which an electric dipole →P is placed as shown. Which of the following statements is correct?

View SolutionA uniform but increasing with time magnetic field exists in as cylindrical region. The direction of force on an electron at P as

View SolutionAn electric field (vec E) and a magnetic field (vec B)exist in a region . The fields are not perpendicular to each other.
View Solution
