A cylindrical region of radius R contains a uniform magnetic field parallel to axis with magnitude that is changing linearly with time. If r is the radial distance of a point from axis of cylinder in a plane perpendicular to axis then the magnitude of the induced electrical field outside the cylinder is directly proportional to
r
r2
1/r
1/r2
The correct Answer is:C
To solve the problem, we need to determine how the induced electric field (E) outside a cylindrical region with a changing magnetic field is related to the radial distance (r) from the axis of the cylinder.
1. Understanding the Setup:
We have a cylinder with a uniform magnetic field (B) parallel to its axis, which is changing linearly with time. We denote the radius of the cylinder as R and the radial distance from the axis as r.
2. Using Faraday's Law of Electromagnetic Induction:
According to Faraday's law, the induced electromotive force (EMF) around a closed loop is equal to the negative rate of change of magnetic flux through the loop:
∮E⋅dL=−dΦBdt
where ΦB is the magnetic flux.
3. Calculating Magnetic Flux (ΦB):
The magnetic flux through the area A of the cylinder is given by:
ΦB=B⋅A⋅cos(θ)
Here, θ=0 degrees because the magnetic field is parallel to the axis of the cylinder, thus:
ΦB=B⋅πR2
4. Finding the Rate of Change of Magnetic Flux:
Since the magnetic field B is changing with time, we can express the rate of change of flux as:
dΦBdt=dBdt⋅πR2
5. Setting Up the Integral:
The closed loop integral of the electric field around a circle of radius r (where r>R) is:
∮E⋅dL=E⋅(2πr)
Therefore, we can write:
E⋅(2πr)=−dΦBdt
6. Substituting the Rate of Change of Flux:
Substituting the expression for dΦBdt into the equation gives:
E⋅(2πr)=−dBdt⋅πR2
7. Solving for the Electric Field (E):
Rearranging the equation to solve for E:
E=−R22r⋅dBdt
The negative sign indicates the direction of the induced electric field, but we are interested in the magnitude.
8. Final Relation:
The magnitude of the induced electric field E is directly proportional to:
E∝1r
Conclusion:
Thus, the magnitude of the induced electric field outside the cylinder is directly proportional to 1r.
We have a cylinder with a uniform magnetic field (B) parallel to its axis, which is changing linearly with time. We denote the radius of the cylinder as R and the radial distance from the axis as r.
According to Faraday's law, the induced electromotive force (EMF) around a closed loop is equal to the negative rate of change of magnetic flux through the loop:
∮E⋅dL=−dΦBdt
where ΦB is the magnetic flux.
The magnetic flux through the area A of the cylinder is given by:
ΦB=B⋅A⋅cos(θ)
Here, θ=0 degrees because the magnetic field is parallel to the axis of the cylinder, thus:
ΦB=B⋅πR2
Since the magnetic field B is changing with time, we can express the rate of change of flux as:
dΦBdt=dBdt⋅πR2
The closed loop integral of the electric field around a circle of radius r (where r>R) is:
∮E⋅dL=E⋅(2πr)
Therefore, we can write:
E⋅(2πr)=−dΦBdt
Substituting the expression for dΦBdt into the equation gives:
E⋅(2πr)=−dBdt⋅πR2
Rearranging the equation to solve for E:
E=−R22r⋅dBdt
The negative sign indicates the direction of the induced electric field, but we are interested in the magnitude.
The magnitude of the induced electric field E is directly proportional to:
E∝1r
Thus, the magnitude of the induced electric field outside the cylinder is directly proportional to 1r.
Similar Questions
A cylinder of radius R and length l is placed in a uniform electric field E parallel to the axis of the cylinder. The total flux over the curved surface of the cylinder is
View SolutionA cylinder of radius R and length L is placed in a uniform electric field E parallel to the axis. The total flux for the surface of the cylinder is given by
View SolutionA cylinder of radius R and length L is placed in a uniform electric field E parallel to the axis. The total flux for the surface of the cylinder is given by
View SolutionA long, cylindrical wire of radius b carries a current i distributed uniformly over its cross section. Find the magnitude of the magnetic field at a point inside the wire at a distance a from the axis.
View SolutionA cylindrical space of radius R is filled with a uniform magnetic induction parallel to the axis of the cylinder. If B charges at a constant rate, the graph showin the variation of induced electric field with distance r from the axis of cylinder is
View SolutionConsider a cylindrical surface of radius R and length l in a uniform electric field E. Compute the electric flux if the axis of the cylinder is parallel to the field direction.
View SolutionLet r be the distance of a point on the axis of a bar magnet from its center. The magnetic field at such a point is proportional to
View SolutionFigure shows a circular region of radius R in which uniform magnetic field B exists. The magnetic field is increasing at a rate dBdt. The induced electric field at a distance r from the centre for r<R is

View SolutionThe ratio of the magnetic field at the centre of a current carrying coil of the radius a and at distance 'a' from centre of the coil and perpendicular to the axis of coil is
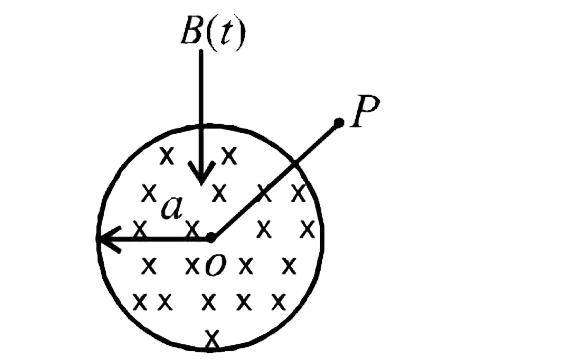
View SolutionA uniform but time-varying magnetic field B(t) exists in a circular region of radius a and is directed into the plane of the paper, as shown. The magnitude of the induced electric field at point P at a distance r from the centre of the circular region
View Solution