STATEMENT-1: The capacitance of the capacitor remains constant irrespective of the charge present on it. because
STATEMENT-2: Capacitance depends on the size and the shape of the capacitor and also on the surrounding medium.
Statement-1 is True , Statement-2 is True , Statement-2 is a correct explanation for Statement-1.
Statement-1 is True , Statement-2 is True , Statement-2 is NOT a correct explanation for Statement-1.
Statement-1 is True , Statement-2 is False
Statement-1 is False , Statement-2 is True
The correct Answer is:A
To analyze the given statements, we need to break down the concepts of capacitance and how it relates to charge and the physical characteristics of a capacitor.
1. Understanding Capacitance:
- Capacitance (C) is defined as the ability of a capacitor to store charge (Q) per unit voltage (V). Mathematically, it is expressed as:
C=QV
- This means that for a given charge and voltage, capacitance is a constant value.
2. Dependence on Physical Characteristics:
- The capacitance of a capacitor depends on its physical characteristics, specifically:
- The area (A) of the plates: Larger plates can store more charge.
- The distance (d) between the plates: Closer plates can store more charge.
- The permittivity (ϵ) of the medium between the plates: Different materials can affect the ability to store charge.
- The formula for the capacitance of a parallel plate capacitor is:
C=ϵAd
- This shows that capacitance is determined by the size and shape of the capacitor and the surrounding medium.
3. Independence from Charge:
- The charge (Q) on a capacitor can change when a voltage (V) is applied, but the capacitance (C) itself remains constant as long as the physical characteristics (A, d, and ϵ) do not change.
- Therefore, the capacitance does not depend on the amount of charge stored on the capacitor.
4. Conclusion:
- Statement 1 is true: The capacitance of the capacitor remains constant irrespective of the charge present on it.
- Statement 2 is also true: Capacitance depends on the size and shape of the capacitor and the surrounding medium.
- Statement 2 correctly explains Statement 1.
Final Answer:
- Both statements are true, and Statement 2 is the correct explanation for Statement 1.
---
- Capacitance (C) is defined as the ability of a capacitor to store charge (Q) per unit voltage (V). Mathematically, it is expressed as:
C=QV
- This means that for a given charge and voltage, capacitance is a constant value.
- The capacitance of a capacitor depends on its physical characteristics, specifically:
- The area (A) of the plates: Larger plates can store more charge.
- The distance (d) between the plates: Closer plates can store more charge.
- The permittivity (ϵ) of the medium between the plates: Different materials can affect the ability to store charge.
- The formula for the capacitance of a parallel plate capacitor is:
C=ϵAd
- This shows that capacitance is determined by the size and shape of the capacitor and the surrounding medium.
- The charge (Q) on a capacitor can change when a voltage (V) is applied, but the capacitance (C) itself remains constant as long as the physical characteristics (A, d, and ϵ) do not change.
- Therefore, the capacitance does not depend on the amount of charge stored on the capacitor.
- Statement 1 is true: The capacitance of the capacitor remains constant irrespective of the charge present on it.
- Statement 2 is also true: Capacitance depends on the size and shape of the capacitor and the surrounding medium.
- Statement 2 correctly explains Statement 1.
- Both statements are true, and Statement 2 is the correct explanation for Statement 1.
Similar Questions
STATEMENT 1: Energy stored in the capacitor is less than the energy battery during the charging of capacitor. because
STATEMENT-2: Some of the energy is converted into heat.
View SolutionThe capacitance of a parallel plate capacitor is 2μF and the charge on its positive plate is 2μC. If the charge on its plates is doubled, the capacitance of the capacitor
View SolutionThe capacitance of the capacitors of plate areas A1 and A2(A1<A2) at a distance d is

View SolutionSTATEMENT-1 : When the plates of a charged capacitor are connected to a resistor, a current starts flowing in the resistor.
and
STATEMENT-2 : A charged capacitor acts as a battery of steady emf.
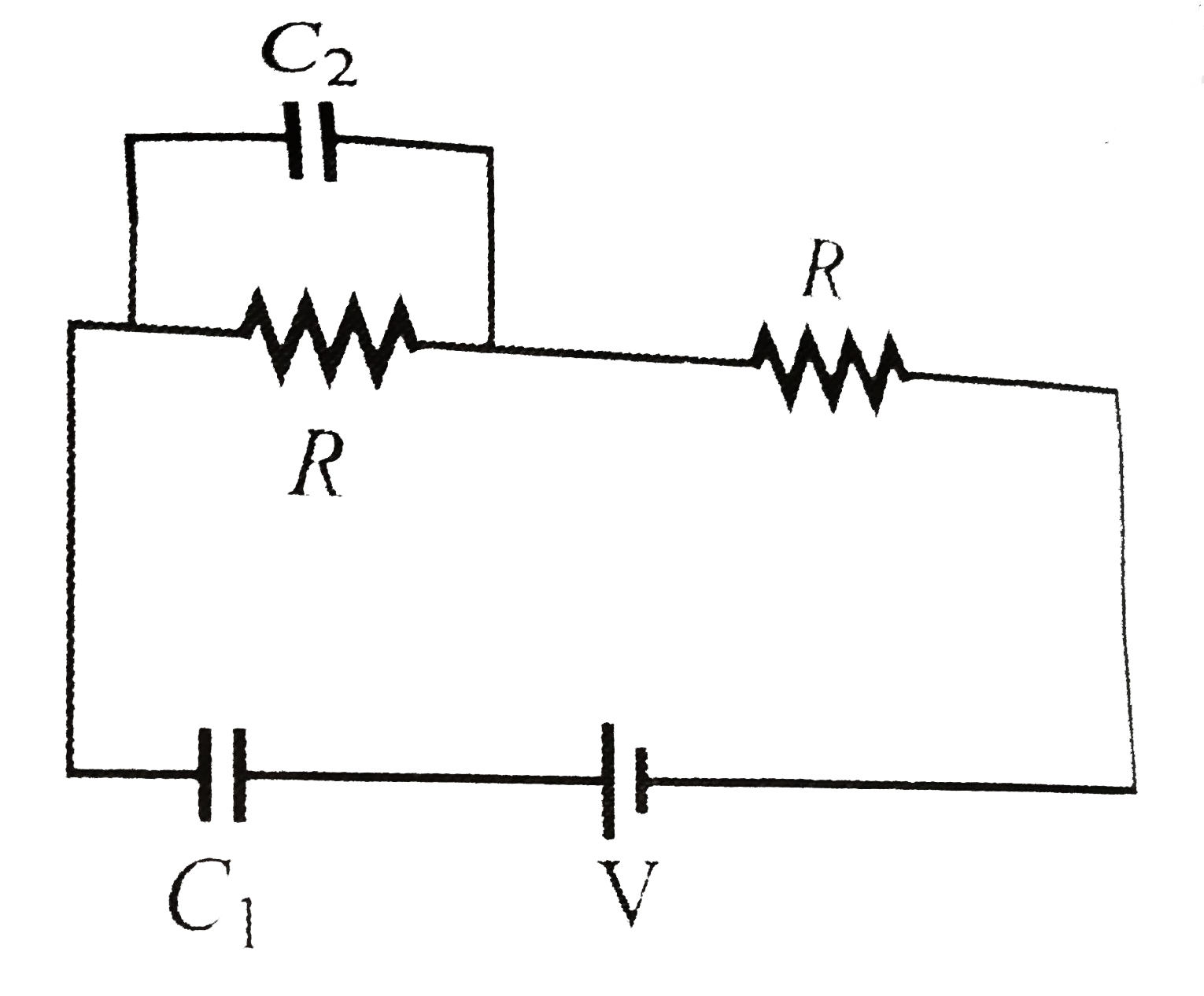
View SolutionCharge on the capacitor having capacitance C2 in steady state is
View SolutionConsider these three statements for a capacitor
STATEMENT-1 : Capacitance of a capacitor must increase when a dielectric is inserted in between the capacitor plates.
STATEMENT-2 : Potential differenece across a charged capacitor must increase if a dielectric is inserted in between the capacitor plates.
STATEMENT-3 : Electrostatic energy stored by the capacitor may increase if a dielectric inserted in between the capacitor plates
View SolutionSTATEMENT-1 : If the distance plates of a charged isolated capacitor increases, then the potential energy in the electric field of capacitor increases.
and
STATEMENT-2 : The energy stored in a capacitor is always directly proportional to separation between the plates.
View SolutionThe capacitance of capacitor of plate areas A1 and A2 (A1<A2 at a distance d is

View SolutionAssertion : If the distance between parallel plates of a capacitor is halved and dielectric constant is made three times, then the capacitance becomes 6 times.
Reason : Capacity of the capacitor depends upon the nature of the material between the plates.
View SolutionTwo parallel conducting plates of a capacitor of capacitance C containing charges Q and -2Q at a distance d apart. Find out potential difference between the plates of capacitors.
View Solution
