A uniformly tapering circular bar has its diameter ranging from (D-a) to (D+a) along its length L. The bar is subjected to an axial force P and young's modulus is Y. The percentage error in the calculation of elongation of bar if mean diameter is used in calculation instead of actual diameter.
2a2D2×100
(10aD)2
(a10D)2
aD×100
The correct Answer is:B
To solve the problem of calculating the percentage error in the elongation of a uniformly tapering circular bar when using the mean diameter instead of the actual diameter, we can follow these steps:
1. Identify the diameters:
- Let the larger diameter be D1=D+a and the smaller diameter be D2=D−a.
- The mean diameter Dm can be calculated as:
Dm=D1+D22=(D+a)+(D−a)2=D
2. Calculate the change in length for the tapering bar:
- The change in length ΔL for an element of the bar can be expressed as:
ΔL=P⋅dxA⋅Y
- The area A of the element with diameter d is:
A=πd24
- Since the diameter varies linearly from D1 to D2, we can express the diameter d at a distance x from D1 as:
d=D1−(D1−D2L⋅x)=D1−(2aL⋅x)
3. Integrate to find total elongation:
- Substitute d into the expression for ΔL and integrate from 0 to L:
ΔL=∫L0P⋅dx(π4⋅d2)⋅Y
- This leads to:
ΔL=4PπY∫L0dxd2
- After performing the integration, we find:
ΔL=4PLπY⋅1D1D2
4. Calculate elongation using mean diameter:
- If we consider the bar to have a uniform diameter Dm, the elongation ΔL′ would be:
ΔL′=4PLπY⋅1D2m
- Given that Dm=D, we have:
ΔL′=4PLπY⋅1D2
5. Calculate the percentage error:
- The percentage error in elongation when using the mean diameter instead of the actual diameter is given by:
Percentage Error=(ΔL−ΔL′ΔL)×100
- Substituting the values:
Percentage Error=⎛⎜⎝4PLπY⋅1D1D2−4PLπY⋅1D24PLπY⋅1D1D2⎞⎟⎠×100
- Simplifying this expression leads to:
Percentage Error=(D2−D1D2D2)×100
- Finally, substituting D1=D+a and D2=D−a:
Percentage Error=(D2−(D2−a2)D2)×100=(a2D2)×100
Final Result:
The percentage error in the calculation of elongation of the bar when using the mean diameter instead of the actual diameter is:
Percentage Error=a2D2×100
- Let the larger diameter be D1=D+a and the smaller diameter be D2=D−a.
- The mean diameter Dm can be calculated as:
Dm=D1+D22=(D+a)+(D−a)2=D
- The change in length ΔL for an element of the bar can be expressed as:
ΔL=P⋅dxA⋅Y
- The area A of the element with diameter d is:
A=πd24
- Since the diameter varies linearly from D1 to D2, we can express the diameter d at a distance x from D1 as:
d=D1−(D1−D2L⋅x)=D1−(2aL⋅x)
- Substitute d into the expression for ΔL and integrate from 0 to L:
ΔL=∫L0P⋅dx(π4⋅d2)⋅Y
- This leads to:
ΔL=4PπY∫L0dxd2
- After performing the integration, we find:
ΔL=4PLπY⋅1D1D2
- If we consider the bar to have a uniform diameter Dm, the elongation ΔL′ would be:
ΔL′=4PLπY⋅1D2m
- Given that Dm=D, we have:
ΔL′=4PLπY⋅1D2
- The percentage error in elongation when using the mean diameter instead of the actual diameter is given by:
Percentage Error=(ΔL−ΔL′ΔL)×100
- Substituting the values:
Percentage Error=⎛⎜⎝4PLπY⋅1D1D2−4PLπY⋅1D24PLπY⋅1D1D2⎞⎟⎠×100
- Simplifying this expression leads to:
Percentage Error=(D2−D1D2D2)×100
- Finally, substituting D1=D+a and D2=D−a:
Percentage Error=(D2−(D2−a2)D2)×100=(a2D2)×100
The percentage error in the calculation of elongation of the bar when using the mean diameter instead of the actual diameter is:
Percentage Error=a2D2×100
Similar Questions
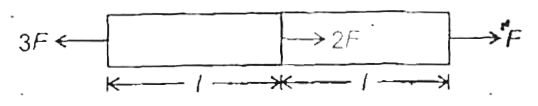
A bar is subjected to axial forces as shown. is the modulus of elasticity of the bar is E and A is it cross-section area. Its elongation will be
View SolutionA bar of mass m and length l is hanging from point A as shown in figure.Find the increase in its length due to its own weight. The young's modulus of elasicity of the wire is Y and area of cross-section of the wire is A.

View SolutionA bar of mass m and length l is hanging from point A as shown in figure.Find the increase in its length due to its own weight. The young,s modulus of elasicity of the wire is Y and area of cross-section of the wire is A.

View SolutionThe length of a cylinder is measured with a meter rod having least count 0.1cm. Its diameter is measured with Vernier calipers having least count 0.01cm. Given that length is 5.0cm and radius is 2cm. Find the percentage error in the calculated value of the volume.
View SolutionThe diameter of a brass rod is 4 mm and Young's modulus of brass is 9×1010N/m2. The force required to stretch by 0.1 % of its length is
View SolutionThe length of a cylinder is measured with a meter rod having least count 0.1cm. Its diameter is measured with Vernier calipers having least count 0.01cm. Given that length is 5.0cm and radius is 2cm. Find the percentage error in the calculated value of the volume.
View SolutionA bar of mass m and length l is hanging from point A as shown in the figure . If the Young's modulus of elasticity of the bar is Y and area of cross - section of the wire is A, then the increase in its length due to its own weight will be

View SolutionThe bar shown in the figure is made of a single piece of material. It is fixed at one end. It consists of two segments of equal lengh L/2 each but different cross sectional area A and 2A. Find the ratio of total elongation in the bar to the elongation produced in thicker segment under the action of an axial force F. Consider the shape of the joint to remain circular. (Given :Y is Young's modulus.
View SolutionA wire of length l and cross-sectional are A is suspended at one of its ends from a ceiling. What will be its strain energy due to its own weight, if the density and Young's modulus of the material of the wire be d and Y?
View SolutionA long solenoid of length L has a mean diameter D. It has n layers of windings of N turns each. If it carries a current ‘i’ the magnetic field at its centre will be
View Solution
