Statement 1: Heat supplied to a gas is process is 100 J and work done by the gas in the same process is 120 J, then pressure of he gas in the process should increase.
because
Statement2: Work done by the gas is greater than the heat supplied to the gas. Hence, internal energy of the gas should decrease.
Statement -1 is true, Statement -2, is true, Statement-2 is a correct explanation for statement-1.
Statement -1 is true, Statement -2 is true, Statement -2 isnot a correct explanation for statement -1
Statement -1 is true, Statement -2 is false.
Statement -1 is false, Statement -2 is true.
The correct Answer is:D
To analyze the statements provided, we will use the First Law of Thermodynamics, which states:
ΔU=Q−W
Where:
- ΔU is the change in internal energy,
- Q is the heat supplied to the system,
- W is the work done by the system.
Step 1: Identify the values given in the problem.
- Heat supplied to the gas (Q) = 100 J
- Work done by the gas (W) = 120 J
Step 2: Apply the First Law of Thermodynamics.
Using the First Law of Thermodynamics, we can calculate the change in internal energy (ΔU):
ΔU=Q−W
Substituting the values:
ΔU=100J−120J=−20J
Step 3: Analyze the change in internal energy.
The negative value of ΔU indicates that the internal energy of the gas decreases by 20 J. This supports Statement 2, which claims that since the work done by the gas is greater than the heat supplied, the internal energy should decrease.
Step 4: Evaluate Statement 1 regarding pressure.
Statement 1 claims that the pressure of the gas should increase. However, we need to consider that the work done by the gas is greater than the heat supplied. This means that the gas is doing more work than the energy it is receiving as heat, which typically leads to a decrease in internal energy and potentially a decrease in temperature, depending on the specific process.
In many cases, if the gas expands (doing work), it may not necessarily lead to an increase in pressure. The pressure could remain constant or even decrease if the volume increases significantly. Thus, we cannot definitively conclude that the pressure increases based solely on the information given.
Conclusion:
- Statement 1 is false: The pressure of the gas does not necessarily increase.
- Statement 2 is true: The internal energy of the gas decreases because the work done exceeds the heat supplied.
Final Answer:
- Statement 1: False
- Statement 2: True
---
- ΔU is the change in internal energy,
- Q is the heat supplied to the system,
- W is the work done by the system.
- Heat supplied to the gas (Q) = 100 J
- Work done by the gas (W) = 120 J
Using the First Law of Thermodynamics, we can calculate the change in internal energy (ΔU):
ΔU=Q−W
Substituting the values:
ΔU=100J−120J=−20J
The negative value of ΔU indicates that the internal energy of the gas decreases by 20 J. This supports Statement 2, which claims that since the work done by the gas is greater than the heat supplied, the internal energy should decrease.
Statement 1 claims that the pressure of the gas should increase. However, we need to consider that the work done by the gas is greater than the heat supplied. This means that the gas is doing more work than the energy it is receiving as heat, which typically leads to a decrease in internal energy and potentially a decrease in temperature, depending on the specific process.
- Statement 1 is false: The pressure of the gas does not necessarily increase.
- Statement 2 is true: The internal energy of the gas decreases because the work done exceeds the heat supplied.
- Statement 1: False
- Statement 2: True
Similar Questions
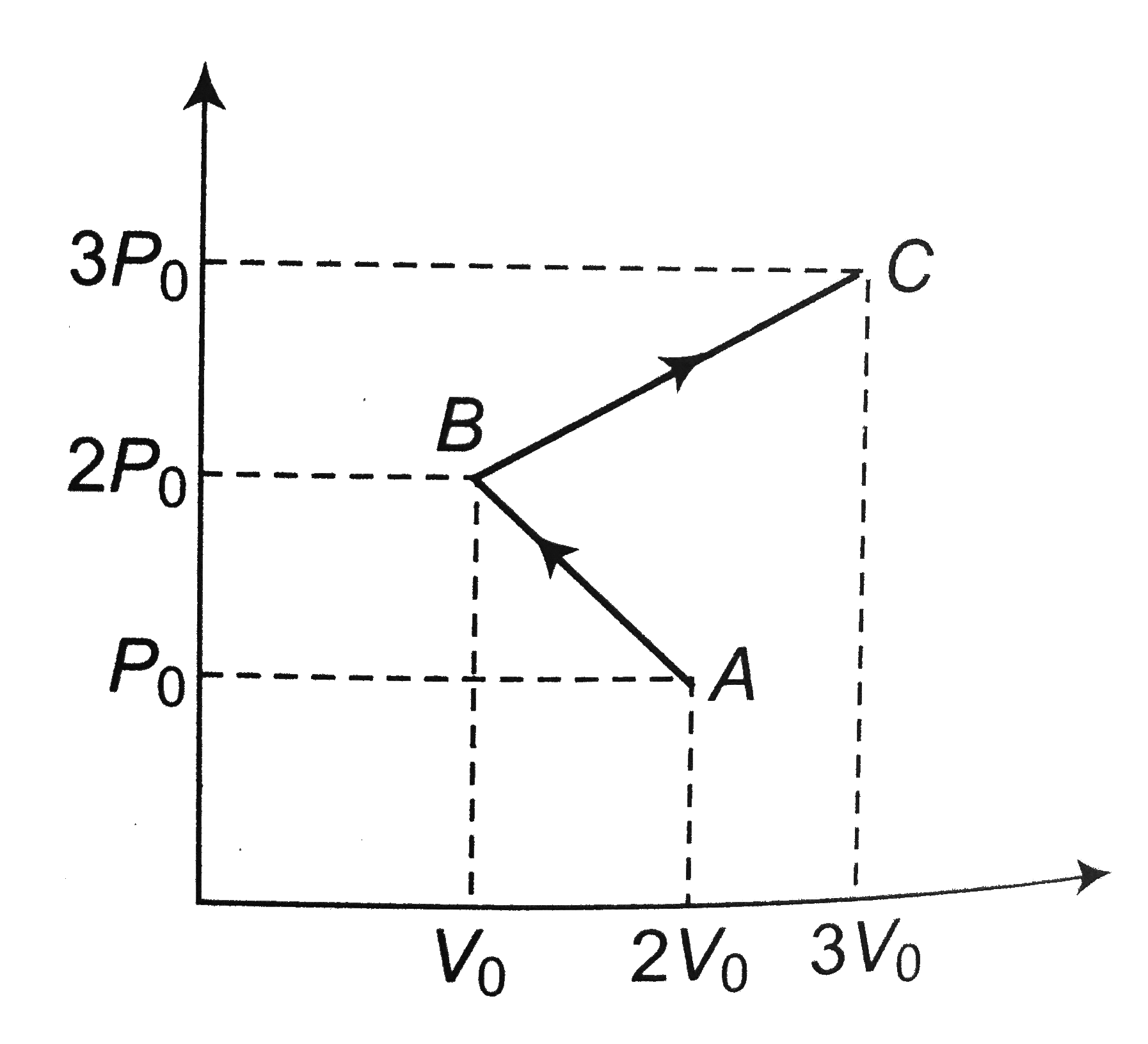
Find the work done by the gas in the process ABC.
View SolutionFind work done by the gas in the process shown in figure.

View SolutionFind work done by the gas in the process shown in figure.

View SolutionWork done by a gas in a given process is −20J. Heat given to the gas is 60J. Find change in internal energy of the gas.
View SolutionHeat taken from a gas in a process is 80 J and increase in internal energy of the gas is 20J. Find work done by the gas in the given process.
View SolutionIn a thermodynamic process on an ideal diatomic gas, work done by the gas is η times the heat supplied (η<1). The molar heat capacity of the gas for the process is
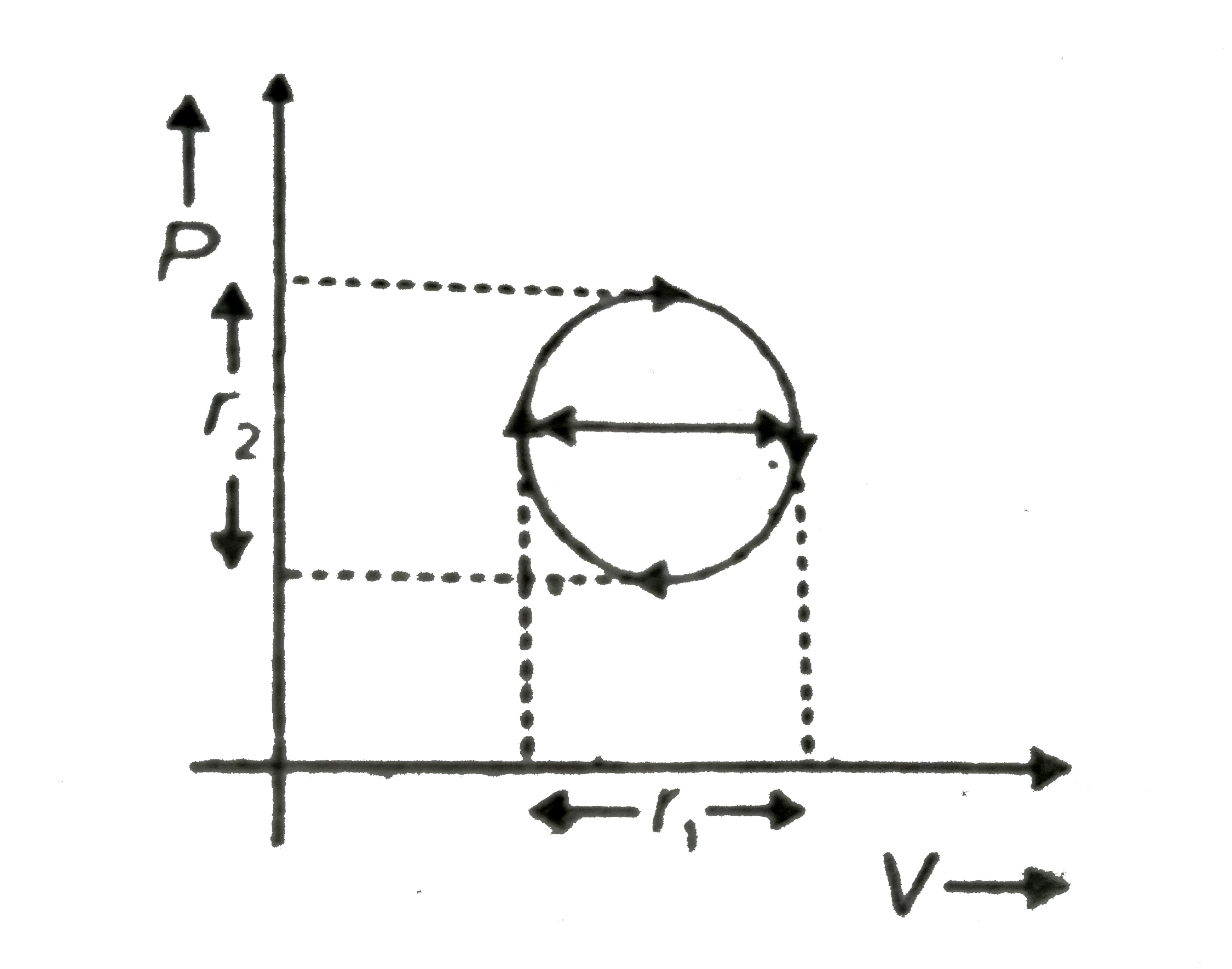
View SolutionA sample of gas is going through cyclic process, work done by gas is
View SolutionThe thermodynamic process in which no work is done on or by the gas is
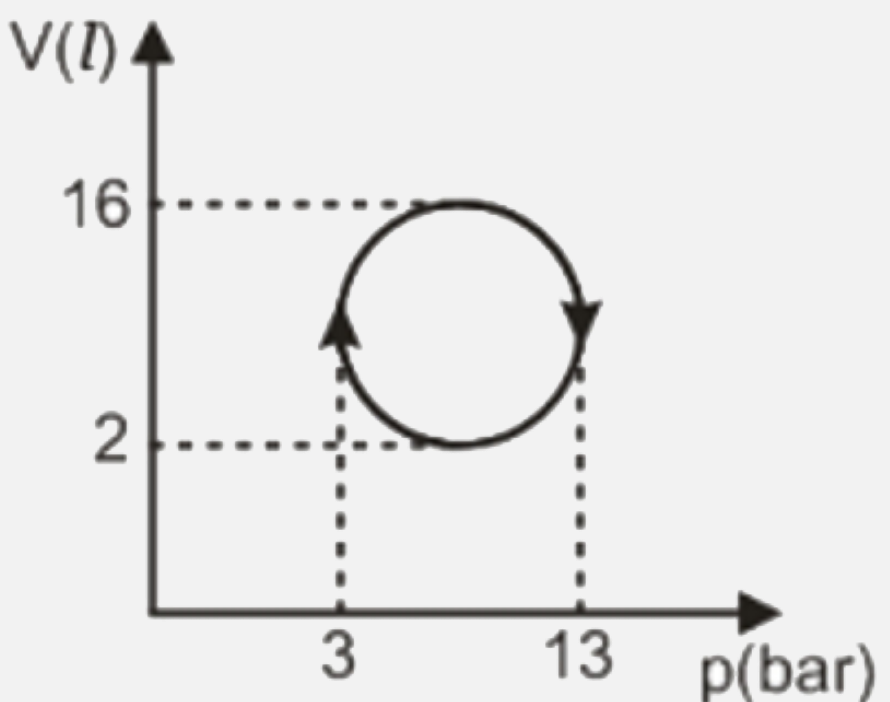
View SolutionWork done (in kJ) by the gas in the following cyclic process is
View SolutionIf heat is supplied to an ideal gas in an isothermal process.
View Solution
