Question

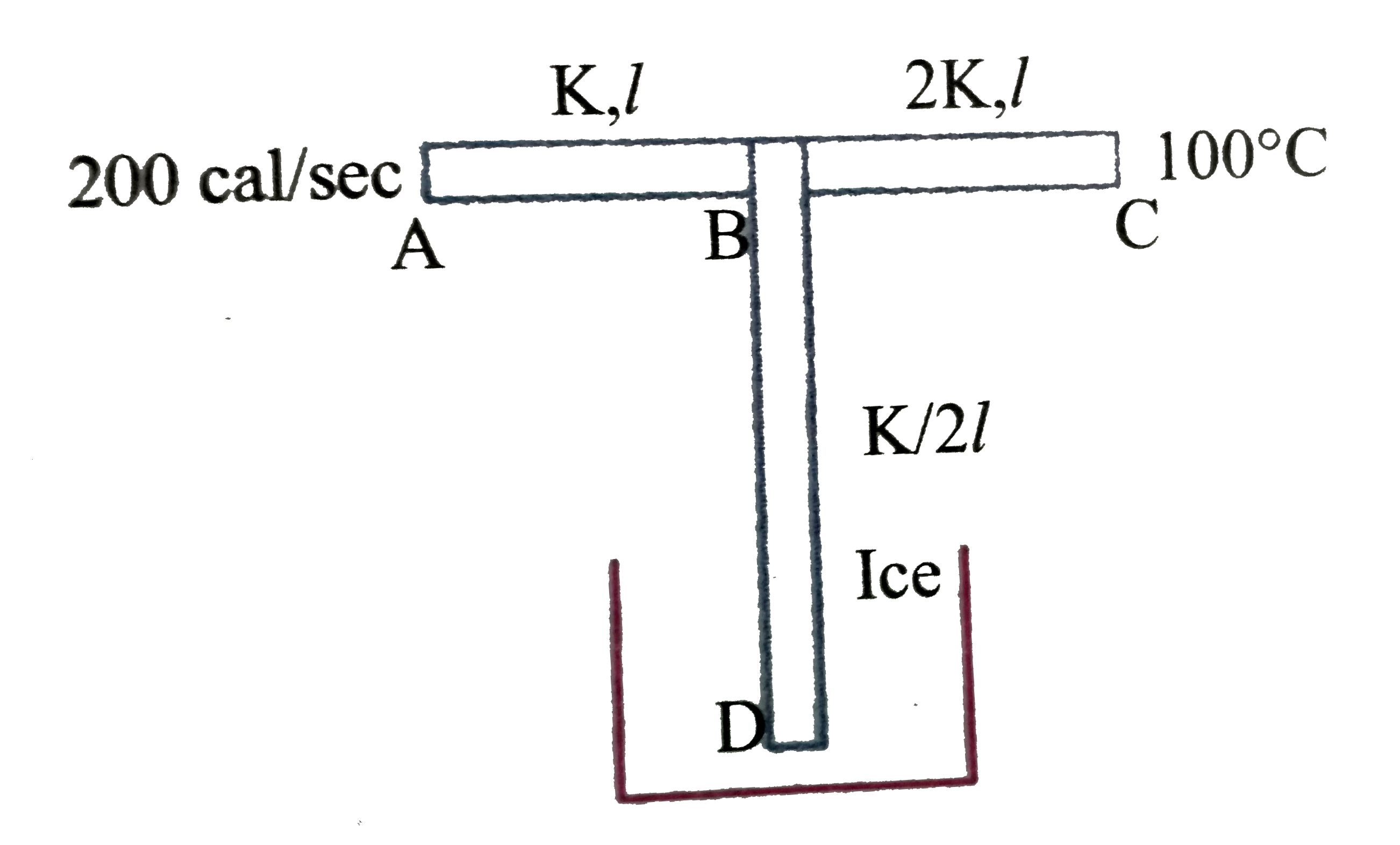
Three rods AB,BC and BD of same length l and cross sectional area A are arranged as shown. The end D is immersed in ice whose mass is 440 gm. Heat is being supplied at constant rate of 200 cal /sec from the end A. Find out time (in sec) in which wholeice will melt. (latent heat of fusion of ice is 80 cal/gm)
Given k (thermal conductivity) =100 cal/m/sec/${}^{\circ }C,A=10c{m}^2,l=1$m

Answer

800 sec

Explanation

To solve the problem step by step, we will follow the heat transfer principles and the given data.

Step 1: Understand the Problem
We have three rods AB, BC, and BD, each of length \( l = 1 \, \text{m} \) and cross-sectional area \( A = 10 \, \text{cm}^2 \). Heat is supplied at a constant rate of \( Q = 200 \, \text{cal/sec} \) from end A, and end D is immersed in ice with a mass of \( m = 440 \, \text{g} \). The latent heat of fusion of ice is \( L = 80 \, \text{cal/g} \).

Step 2: Calculate the Total Heat Required to Melt the Ice
The total heat required to melt the ice can be calculated using the formula:
\( Q_{\text{required}} = m \cdot L \)
Substituting the values:
\( Q_{\text{required}} = 440 \, \text{g} \times 80 \, \text{cal/g} = 35200 \, \text{cal} \)

Step 3: Calculate the Time Required to Melt the Ice
The time required to supply this amount of heat at a rate of \( Q = 200 \, \text{cal/sec} \) can be calculated using the formula:
\( t = \frac{Q_{\text{required}}}{Q} \)
Substituting the values:
\( t = \frac{35200 \, \text{cal}}{200 \, \text{cal/sec}} = 176 \, \text{sec} \)

Step 4: Conclusion
The time required to melt the entire ice is \( t = 176 \, \text{sec} \).

Summary of the Solution
1. Calculate the total heat required to melt the ice: \( Q_{\text{required}} = 35200 \, \text{cal} \).
2. Calculate the time using the heat supply rate: \( t = 176 \, \text{sec} \).