Assertion: Rutherford's gold foil experiment didn't explain hydrogen spectrum
Reason: Bohr's model contradicted Heisenberg's uncertainty principle
Assertion is true, reason is true and reason is correct explanation for assertion
Assertion is true, reason is true and reason is not correct explanation for assertion
Assertion is true and reason is false
Assertion is false and reason is true
To analyze the given statements regarding Rutherford's gold foil experiment and Bohr's model of the atom, we can break down the solution into clear steps.
1. Understanding the Assertion:
- The assertion states that "Rutherford's gold foil experiment didn't explain hydrogen spectrum."
- Rutherford's gold foil experiment was pivotal in discovering the nucleus of the atom. However, it did not provide an explanation for the spectral lines observed in hydrogen, which are the result of electron transitions between energy levels.
2. Understanding the Reason:
- The reason states that "Bohr's model contradicted Heisenberg's uncertainty principle."
- Bohr's model introduced the idea of quantized orbits for electrons around the nucleus, where the position and momentum of an electron could be defined. However, this contradicts Heisenberg's uncertainty principle, which states that it is impossible to know both the exact position and momentum of a particle simultaneously.
3. Evaluating the Truth of the Statements:
- Assertion: True. Rutherford's experiment did not explain the hydrogen spectrum; this was later addressed by Bohr.
- Reason: True. Bohr's model indeed contradicts the Heisenberg uncertainty principle because it assumes that both position and momentum of electrons can be precisely defined.
4. Conclusion:
- While both the assertion and reason are true, the reason does not serve as a correct explanation for the assertion. The assertion is about the limitations of Rutherford's experiment, while the reason discusses a different aspect of quantum mechanics related to Bohr's model.
Final Answer:
- Assertion: True
- Reason: True
- Explanation: The reason does not correctly explain the assertion.
- The assertion states that "Rutherford's gold foil experiment didn't explain hydrogen spectrum."
- Rutherford's gold foil experiment was pivotal in discovering the nucleus of the atom. However, it did not provide an explanation for the spectral lines observed in hydrogen, which are the result of electron transitions between energy levels.
- The reason states that "Bohr's model contradicted Heisenberg's uncertainty principle."
- Bohr's model introduced the idea of quantized orbits for electrons around the nucleus, where the position and momentum of an electron could be defined. However, this contradicts Heisenberg's uncertainty principle, which states that it is impossible to know both the exact position and momentum of a particle simultaneously.
- Assertion: True. Rutherford's experiment did not explain the hydrogen spectrum; this was later addressed by Bohr.
- Reason: True. Bohr's model indeed contradicts the Heisenberg uncertainty principle because it assumes that both position and momentum of electrons can be precisely defined.
- While both the assertion and reason are true, the reason does not serve as a correct explanation for the assertion. The assertion is about the limitations of Rutherford's experiment, while the reason discusses a different aspect of quantum mechanics related to Bohr's model.
- Assertion: True
- Reason: True
- Explanation: The reason does not correctly explain the assertion.
Similar Questions
Association OF de-broglie concept with bohr''''s model,heisenberg''''s uncertainty principle,quantum numbers
View SolutionQuestions based on Derivation of energy of electron| Spectrum of hydrogen likes species and drawbacks of Bohr's model of Atom| Wave nature of particles and de-brogile hypothesis| Heisenberg's uncertainity principle and wave mechanical model of Atom
View Solution
Knowledge Check
Given below are two statements :
Statement I : Rutherfods's gold foil experiment cannot explain the line spectrum of hydrogen atom.
Statement II : Bohr's model of hydrogen atom contradicts Heisenberg's uncertainity principle.
In the light of the above statements, choose the most appropriate answr from the options given below :AStatement I is false but statement II is trueBStatement I is true but statement II is falseCBoth statement I and statement II is falseDBoth statement I and statement II is trueBohr's model of atom can explain the spectrum of all except de-Broglie's And Heisenberg Uncertainity Principle
AHBHe+CLi+2DHeAssertion : In Rutherford's gold foil experiment,very few d-particles are deflected back. Reason : Nucleus present inside the atom is heavy.
AIf both assertion and reason are true and reason is the correct explanation of assertion.BIf both assertion and reason are true but reason is not the correct explanation of assertion.CIf assertion is true but reason is false.DIf both assertion and reason are false.
Black Body Radiation|Photoelectric Effect|Dual Behavior Of Electromagnetic Radiation|Bohr's Model For Hydrogen Atom |Significance Of Heisenberg uncertainty Principle|OMR
View SolutionBohr's model of hydrogen atom
Ignores dual behaviour of matter
2 contradicts Heisenberg uncertainty principle
3 Bath 1 and 2
4 None of the above
View SolutionAssertion (A) : In rutherford's gold foil experiment, very few α particle are defected back
Reason (R ) : Nuclear present inside the atom is heavuy
View SolutionAfter the faliure of Bohr atomic theory but its ability to explain to the atomic spectral a need was felt for the new model that could incorporate, the concept of stationary orbit, de Broglie concept, Heisenberg uncertainity principle. The concept that in corporate above facts is called quantum mechanics of the atomic model wave mchanical model. it includes set of quantum number and ∣∣ψ2∣∣ a mathematical expression of the probability of finding an electron at all points in space. This probability function is the best indication available of how the electon behaves, for as a consequence of the Uncertainity Principle, the amount we can know about the electron is limited. While quantum mechanics can tell us the exact probability of finding an electron at any two particular points, it does not tell us how the electron moves from one of these points to the other. Thus the idea of an electron orbit is lost, it is replaced with a description of where the electron is most likely to be found. This total picture of the probability of finding an electron at various points in space is called an orbital.
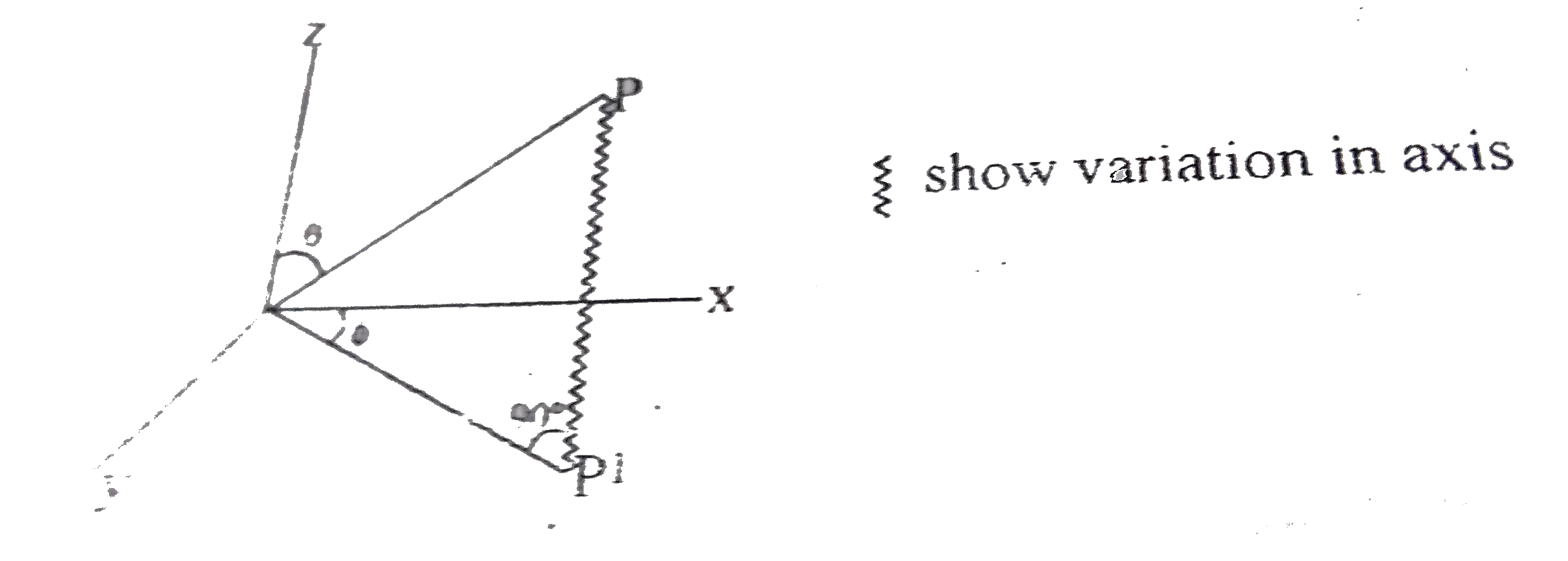
There are various types of orbitals possible, each corresponding to one of the possible combinations of quantum numbers. These orbitals are classified according to the value of n and l associated with them. In order to avoid confusion over the use of two numbers, the numerical velues of l are replaced by letters, electrons in orbitals with l=0 are called s-electrons those occupying orbitals for which l=1 are p-electrons and those for which l=2 are called d-electrons. The numerical and alphabetical correspondences are summarized in table. Using the alphabetical notation for l, we would say that in the ground state of hydrogen atom (n=1,l=0) we have a 1s-electron, or that the electron moves in a 1s-orbital. The relation of the spherical polar co-ordinates r,θ and ϕ to Cartesian coordinates x, y and z. To make the concept of an orbital more meaningful, it is helpful to examine the actual solution of the wave function for the one-electron atom. Because of the spherical sysmmetry of the atom, the wave functions are most simply expressed in terms of a spherical polar-coordinate system, shown in fig., which has its orbit at the nucleus. It is found that the wave function can be expressed as the product of two functions, one of which (the ''angular part'' X) depends only the angle θ and ϕ the other of which (the ''radial part'' R) depends only on the distance from the nucleus. Thus we have
ϕ(r,θ,ϕ)=R(r)X(θ,ϕ)
Angular and radial parts of hydrogen atom wave function
(Angular partX(θ,ϕ),,Radial partRn,l(r)),(X(s)=(14π)1/2,,R(1s)=2(za0)3/2e−σ/2),(X(px)=(34π)1/2sinθcosϕ,,R(2s)=12√2(za0)3/2(2−σ)e−σ/2),(X(py)=(34π)1/2sinθsinϕ,,R(2p)=12√6(za0)3/2σe−σ/2),(X(pz)=(34π)1/2cosθ,,),(X(d22)=(516π)1/2(3cos2θ−1),,),(X(dxz)=(154π)1/2sinθcosθcosϕ,,R(3s)=19√3(za0)3/2(6−6σ+σ2)e−σ/2),(X(dyz)=(154π)1/2sinθcosθsinϕ,,R(3p)=19√6(za0)3/2(4−σ)σe−σ/2),(X(dx2−y2)=(154π)1/2sin2θcos2ϕ,,R(3d)=19√30(za0)3/2σ2e−σ/2),(X(dxy)=(154π)1/2sin2θsin2ϕ,,),(,,σ=2Zrna0,a0=h24π2me2)
This factrorization helps us to visualize the wave function, since it allows us to consider the angular and radial dependences separately. It contains the expression for the angular and radial parts of the one electron atom wave function. Note that the angular part of the wave function for an s-orbital it alwats the same, (1/4π)1/2 regardless of principal quantum number. It is also true that the angular dependence of the p-orbitals and of the d-orbitals is independent of principle quantum number. Thus all orbitals of given types (s, p, or d) have the same angular behavour The table shows, however, that the radial part of the wave function depends both on the principal quantum n and on the angular momentum quantum number l.
To find the wave function for a particular state, we simply multiply the appropriate angular and radial parts togather called normalized wave function.
The probability of finding an electron at a point within an atom is proportional to the square of orbital wave function, i.e., ψ2 at that point. Thus, ψ2 is known as probability density and always a positive quantity.
ψ2dV(orψ2.4πr2dr). represents the probability for finding electron in a small volume dV surrounding the nucleus.
The electron probility density for 1s-orbital best represented by the relation.
View SolutionAfter the faliure of Bohr atomic theory but its ability to explain to the atomic spectral a need was felt for the new model that could incorporate, the concept of stationary orbit, de Broglie concept, Heisenberg uncertainity principle. The concept that in corporate above facts is called quantum mechanics of the atomic model wave mchanical model. it includes set of quantum number and ∣∣ψ2∣∣ a mathematical expression of the probability of finding an electron at all points in space. This probability function is the best indication available of how the electon behaves, for as a consequence of the Uncertainity Principle, the amount we can know about the electron is limited. While quantum mechanics can tell us the exact probability of finding an electron at any two particular points, it does not tell us how the electron moves from one of these points to the other. Thus the idea of an electron orbit is lost, it is replaced with a description of where the electron is most likely to be found. This total picture of the probability of finding an electron at various points in space is called an orbital.
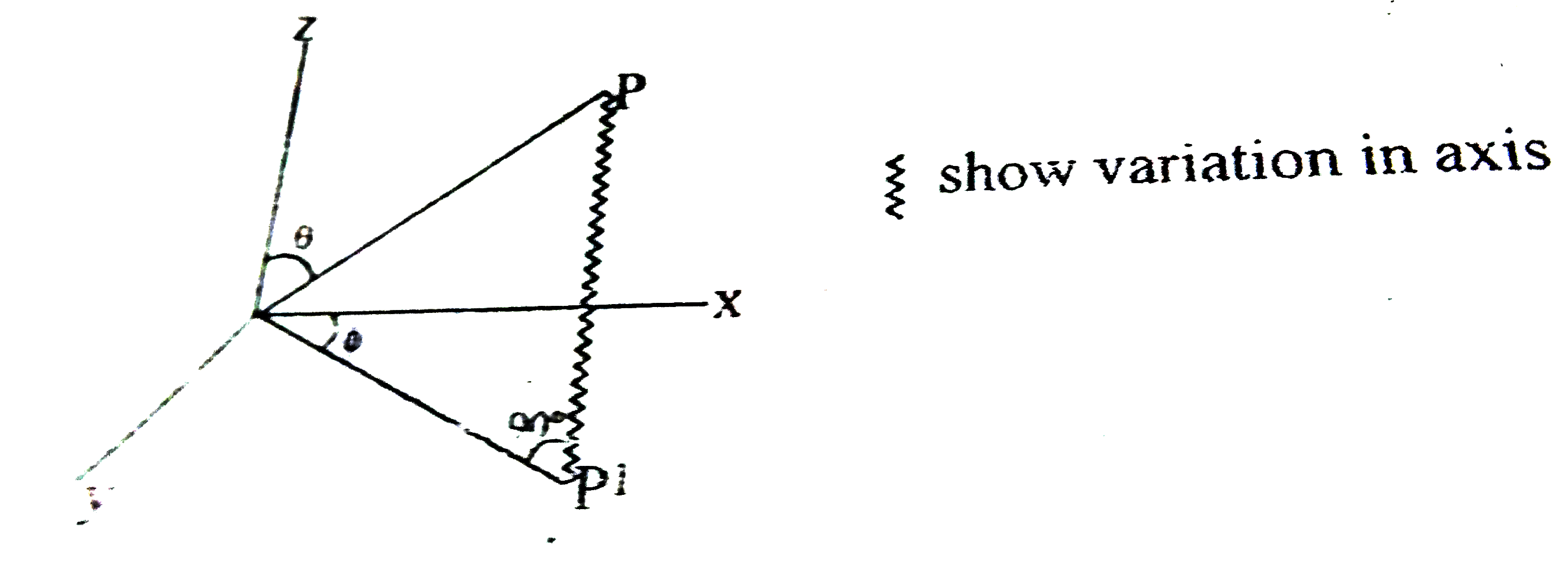
There are various types of orbitals possible, each corresponding to one of the possible combinations of quantum numbers. These orbitals are classified according to the value of n and l associated with them. In order to avoid confusion over the use of two numbers, the numerical velues of l are replaced by letters, electrons in orbitals with l=0 are called s-electrons those occupying orbitals for which l=1 are p-electrons and those for which l=2 are called d-electrons. The numerical and alphabetical correspondences are summarized in table. Using the alphabetical notation for l, we would say that in the ground state of hydrogen atom (n=1,l=0) we have a 1s-electron, or that the electron moves in a 1s-orbital. The relation of the spherical polar co-ordinates r,θ and ϕ to Cartesian coordinates x, y and z. To make the concept of an orbital more meaningful, it is helpful to examine the actual solution of the wave function for the one-electron atom. Because of the spherical sysmmetry of the atom, the wave functions are most simply expressed in terms of a spherical polar-coordinate system, shown in fig., which has its orbit at the nucleus. It is found that the wave function can be expressed as the product of two functions, one of which (the ''angular part'' X) depends only the angle θ and ϕ the other of which (the ''radial part'' R) depends only on the distance from the nucleus. Thus we have
ϕ(r,θ,ϕ)=R(r)X(θ,ϕ)
Angular and radial parts of hydrogen atom wave function
(Angular partX(θ,ϕ),,Radial partRn,l(r)),(X(s)=(14π)1/2,,R(1s)=2(za0)3/2e−σ/2),(X(px)=(34π)1/2sinθcosϕ,,R(2s)=12√2(za0)3/2(2−σ)e−σ/2),(X(py)=(34π)1/2sinθsinϕ,,R(2p)=12√6(za0)3/2σe−σ/2),(X(pz)=(34π)1/2cosθ,,),(X(d22)=(516π)1/2(3cos2θ−1),,),(X(dxz)=(154π)1/2sinθcosθcosϕ,,R(3s)=19√3(za0)3/2(6−6σ+σ2)e−σ/2),(X(dyz)=(154π)1/2sinθcosθsinϕ,,R(3p)=19√6(za0)3/2(4−σ)σe−σ/2),(X(dx2−y2)=(154π)1/2sin2θcos2ϕ,,R(3d)=19√30(za0)3/2σ2e−σ/2),(X(dxy)=(154π)1/2sin2θsin2ϕ,,),(,,σ=2Zrna0,a0=h24π2me2)
This factrorization helps us to visualize the wave function, since it allows us to consider the angular and radial dependences separately. It contains the expression for the angular and radial parts of the one electron atom wave function. Note that the angular part of the wave function for an s-orbital it alwats the same, (1/4π)1/2 regardless of principal quantum number. It is also true that the angular dependence of the p-orbitals and of the d-orbitals is independent of principle quantum number. Thus all orbitals of given types (s, p, or d) have the same angular behavour The table shows, however, that the radial part of the wave function depends both on the principal quantum n and on the angular momentum quantum number l.
To find the wave function for a particular state, we simply multiply the appropriate angular and radial parts togather called normalized wave function.
The probability of finding an electron at a point within an atom is proportional to the square of orbital wave function, i.e., ψ2 at that point. Thus, ψ2 is known as probability density and always a positive quantity.
ψ2dV(orψ2.4πr2dr). represents the probability for finding electron in a small volume dV surrounding the nucleus.
The angular wave function of which orbital with not disturb by the variation with azimuthal angle only
View Solution
