Question

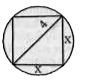
A square is inscribed in a quarter circle in such a way that two of its adjacent vertices on the radius are equidistant from the centre and other two vertices lie on the circumference. If the side of square is $\sqrt{\frac{5}{2}}$ cm, then what is the radius (in cm) of the circle?

Options

A. 2
B. 2.5
C. 5
D. 10

Answer

B

Explanation

To solve the problem of finding the radius of the quarter circle in which a square is inscribed, we can follow these steps:

1. Understanding the Configuration:
- We have a quarter circle with a square inscribed in it.
- Let the center of the quarter circle be point O.
- Let the side length of the square be \( s = \sqrt{\frac{5}{2}} \) cm.
- The square has two vertices (let's call them A and D) on the radii of the quarter circle, and the other two vertices (B and C) on the arc of the quarter circle.

2. Identifying the Triangle:
- Consider triangle OAD, where O is the center, A and D are the vertices of the square on the radii.
- Since A and D are equidistant from O, we can denote the distance from O to A (or D) as \( OA = OD = x \).

3. Applying the Pythagorean Theorem:
- In triangle OAD, we have:
\( AD^2 = OA^2 + OD^2 \)
- Since \( AD = s = \sqrt{\frac{5}{2}} \):
\( \left(\sqrt{\frac{5}{2}}\right)^2 = x^2 + x^2 \)
\( \frac{5}{2} = 2x^2 \)
- Solving for \( x^2 \):
\( x^2 = \frac{5}{4} \)
- Taking the square root:
\( x = \sqrt{\frac{5}{4}} = \frac{\sqrt{5}}{2} \text{ cm} \)

4. Finding the Length of BD:
- Now, consider triangle ABD, where AB = AD = s.
- By the Pythagorean theorem:
\( BD^2 = AB^2 + AD^2 \)
- Substituting \( AB = AD = \sqrt{\frac{5}{2}} \):
\( BD^2 = \left(\sqrt{\frac{5}{2}}\right)^2 + \left(\sqrt{\frac{5}{2}}\right)^2 \)
\( BD^2 = \frac{5}{2} + \frac{5}{2} = 5 \)
- Thus:
\( BD = \sqrt{5} \text{ cm} \)

5. Finding the Radius:
- Now, consider triangle OBD:
\( OB^2 = OD^2 + BD^2 \)
- Substituting \( OD = x = \frac{\sqrt{5}}{2} \) and \( BD = \sqrt{5} \):
\( OB^2 = \left(\frac{\sqrt{5}}{2}\right)^2 + (\sqrt{5})^2 \)
\( OB^2 = \frac{5}{4} + 5 = \frac{5}{4} + \frac{20}{4} = \frac{25}{4} \)
- Thus:
\( OB = \sqrt{\frac{25}{4}} = \frac{5}{2} \text{ cm} = 2.5 \text{ cm} \)

Final Answer
The radius of the quarter circle is \( 2.5 \) cm.