An alternating e.m.f. is applied to a pure inductance. Investigate the phase relationship between current through inductor and em.f. applied to it.

Text Solution

Verified by Experts

Let a source of alternating e.m.f. be connected to a pure circuit containing a pure inductance L only.
Suppose the alternating em.f. supplied is represented by
$E = E_{0} sin ω t$
If $\frac{d I}{d t}$ is the rate of change through L at any instant, then induced e.m.f. in the inductor at the same instant is
$e = - L \frac{d I}{d t}$

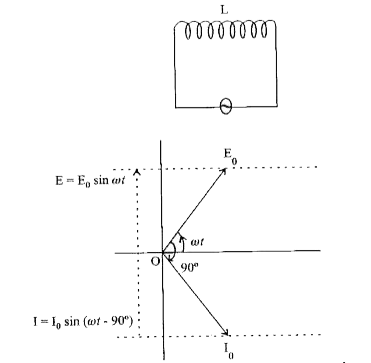
To maintain the flow of current in the circuit, applied voltage must be equal and opposte to the induced voltage.
i.e., $E = - (- L \frac{d I}{d t}) = E_{0} sin ω t$
$d I = \frac{E_{0}}{L} = sin ω t d t$
Integrating both side we get,
$I = \frac{E_{0}}{L} \int sin ω t d t$
$I = \frac{E_{0}}{L} (- \frac{cos ω t}{ω})$
$I = - \frac{E_{0}}{ω L} cos ω t$
$= - \frac{E_{0}}{ω L} sin (\frac{π}{2} - ω t) [∵ sin (90^{\circ} - θ) = cos θ]$
$I = \frac{E_{0}}{ω L} sin (ω t - \frac{π}{2})$
The current will be max.
i.e., $I = I_{0}$ when $sin (ω t - π / 2)$ is maximum
$∴ I_{0} = \frac{E_{0}}{ω L} \times 1 = \frac{E_{0}}{ω L}$
$∴ I = I_{0} sin (ω t - \frac{π}{2})$ ........(2)
conclusion : From equation (1) and (2), it is clear that. In a.c. circuit containing L only, I bags behind E by a phase angle of $\frac{π}{2}$ .
Here, $X_{L} = ω L$

Updated on:21/07/2023

An alternating e.m.f. is applied to purely capacitive circuit. The phase relation between e.m.f. and current flowing in the circuit is or in a circuit containing capacitance only
Ae.m.f. is ahead of current by $π / 2$
BCurrent ahead of e.m.f by $π / 2$
CCurrent lags behind e.m.f. by $π$
DCurrent is ahead of e.m.f. by $π$
An alternating emf, e=300 sin100 $π t$ volt, is applied to a pure resistance of 100 $Ω$ . The rms current through the circuit is
A2.12 A
B0.212 A
C20.12 A
D0.0212 A
If an alternating e.m.f. is applied to a series L-R circuit, the phase angle between e.m.f. and current is given by $tan θ = \frac{ω L}{R}$ . Hence the power factor of the series L-R circuit is
A1. $\frac{ω L}{R}$
B2. $\frac{R}{ω L}$
C3. $\frac{R}{R + ω L}$
D4. $\frac{R}{\sqrt{R^{2} + ω^{2} L^{2}}}$