Prove that the ratio of the areas of two similar triangles is equal to the square of the ratio of their corresponding medians.Solution in Punjabi
Step by step video & image solution for Prove that the ratio of the areas of two similar triangles is equal to the square of the ratio of their corresponding medians. by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
- TRIANGLESBOOK - SWAN PUBLICATIONCHAPTER - TRIANGLESEXERCISE - EXERCISE 6.5
15 Videos
- TRIANGLESBOOK - SWAN PUBLICATIONCHAPTER - TRIANGLESEXERCISE - EXERCISE 6.6 (OPTIONAL)
8 Videos
- TRIANGLESBOOK - SWAN PUBLICATIONCHAPTER - TRIANGLESEXERCISE - EXERCISE 6.3
15 Videos
- SURFACE AREAS AND VOLUMES BOOK - SWAN PUBLICATIONCHAPTER - SURFACE AREAS AND VOLUMES EXERCISE - EXERCISE 13.5(Optional)
7 Videos
Similar Questions
PQR is a triangle right angled at P and M is a point on QR such that PM ⊥ QR. Show that PM2 = QM.MR.
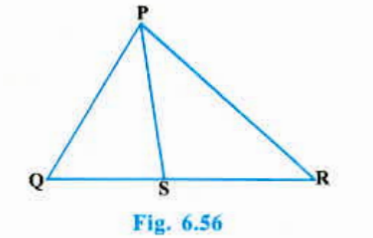
View SolutionIn Fig. 6.56, PS is the bisector of ∠QPR of ΔPQR. Prove that QSSR=PQPR
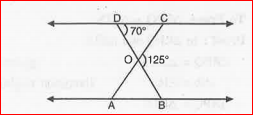
View SolutionIn fig., △ODC−△OBA, ∠BOC=125∘0 and ∠CDO=70∘0. Find ∠DOC, ∠DCO and ∠OAB .
View SolutionA copper wire 3 mm in diameter is wound about a cylinder whose length is 12 cm, and diameter 10 cm,so as to cover the curved surface of the cylinder. Find the length and mass of the wire, assuming the density of copper to 8.88 g per cm3.
View SolutionThe areas of the two similar triangles are in the ratio of the square of the corresponding medians.
View Solutionसिद्ध कीजिए कि दो समरूप त्रिभुजों के क्षेत्रफलों का अनुपात इनकी संगत माध्यिकाओ के अनुपात का वर्ग होता है
View Solutionसिद्ध कीजिए कि दो समरूप त्रिभुजों के क्षेत्रफलों का अनुपात इनकी संगत माध्यिकाओं के अनुपात का वर्ग होता है ।
View SolutionProve that the ratio of areas of two similar triangle is equal to the square of the ratio of their corresponding medians.
View SolutionThe ratio of areas of two similar triangles is equal to the ratio of the squares of corresponding…….
View SolutionProve that the ratio of the areas of two similar triangles is equal to the square of the ratio of their corresponding medians.
View SolutionProve that the ratio of areas of two similar triangles is equal to the square of the ratio of their corresponding medians.
View Solutionprove that The areas of the two similar triangles are in the ratio of the square of the corresponding medians.
View SolutionProve that the ratio at the areas of two similar triangles is equal to the square of the ratio of their corresponding medians.
View SolutionProve that the ratio of the areas of two similar triangles is equal to the square of the ratio of their corresponding medians.
View SolutionProve that the ratio of the areas of two similar triangles is equal to the square of the ratio of their corresponding medians.
View SolutionProve that the ratio of the areas of two similar triangles is equal to the square of the ratio of their corresponding medians.
View Solution
