If M is the mid point of XY,what can you say about the lengths MX and XY?Solution in Punjabi
Step by step video & image solution for If M is the mid point of XY,what can you say about the lengths MX and XY? by Maths experts to help you in doubts & scoring excellent marks in Class 6 exams.
Updated on:21/07/2023Topper's Solved these Questions
- PRACTICAL GEOMETRY BOOK - NAND LAL PUBLICATIONCHAPTER - PRACTICAL GEOMETRY EXERCISE - EXERCISE 14.6
14 Videos
- PRACTICAL GEOMETRY BOOK - NAND LAL PUBLICATIONCHAPTER - PRACTICAL GEOMETRY EXERCISE - ADDITIONAL QUESTIONS FOR PRACTICE (VERY SHORT ANSWER TYPE QUESTIONS )
8 Videos
- PRACTICAL GEOMETRY BOOK - NAND LAL PUBLICATIONCHAPTER - PRACTICAL GEOMETRY EXERCISE - EXERCISE 14.4
1 Videos
- PLAYING WITH NUMBERS BOOK - NAND LAL PUBLICATIONCHAPTER - PLAYING WITH NUMBERS EXERCISE - SAMPLE PAPER FOR PRACTICE
22 Videos
- RATIO AND PROPORTION BOOK - NAND LAL PUBLICATIONCHAPTER - RATIO AND PROPORTION EXERCISE - SAMPLE PAPER FOR PRACTICE
13 Videos
Similar Questions
Draw ∠(POQ) of measure 75∘ and find its line of symetery.
View SolutionLine of symmetry of angle is its
View SolutionDraw any line segment ¯¯¯AB.Mark any piont M on it.Through M draw a perpendicular to ¯¯¯AB.(use ruler and compass).
View SolutionEvery counting number has finite number of
View SolutionFind the number which has the same ratio to 32 as 18 has to 24?
View SolutionWe have AC=XD , C is the mid point of AB and D is the mid point of XY, Using Euclid's axiom ,show that AB=XY
View SolutionProve that the locus of the mid-points of chords of length 2d unit of the hyperbola xy=c2 is (x2+y2)(xy−c2)=d2xy.
View SolutionIf one of the lines of my^(2)+(1-m^(2))xy-mx^(2)=0 is a bisector of the angle between the lines xy=0 then m is
View SolutionThe mid-points of AB and AC of a ΔABC are respectively X and Y. If BC + XY = 12 units, then the value of BC - XY is
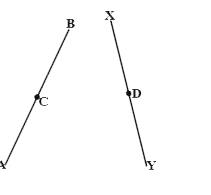
View SolutionIn the adjacent figure, we have AC = XD, C and D are mid points of AB and XY respectively. Show that AB = XY.
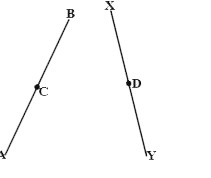
View SolutionIn the adjacent figure, we have AC = XD, C and D are mid points of AB and XY respectively. Show that AB = XY.
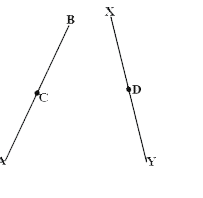
View SolutionIn the adjacent figure, we have AC = XD, C and D are mid points of AB and XY respectively. Show that AB = XY.
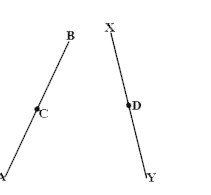
View SolutionIn the adjacent figure, we have AC = XD, C and D are mid points of AB and XY respectively. Show that AB = XY.
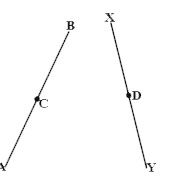
View SolutionIn the adjacent figure, we have AC = XD, C and D are mid points of AB and XY respectively. Show that AB = XY.
View SolutionWhat can you say about the resistance of an ammeter?
View SolutionWhat can you say about the resistance of a voltmeter?
View Solution
