Questions |Maximum Compression Of A Spring|Questions
Step by step video solution for Questions |Maximum Compression Of A Spring|Questions by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Similar Questions
Knowledge Check
Initially spring is at it's natural length and collision is elastic. Then find maximum compression of spring during motion :
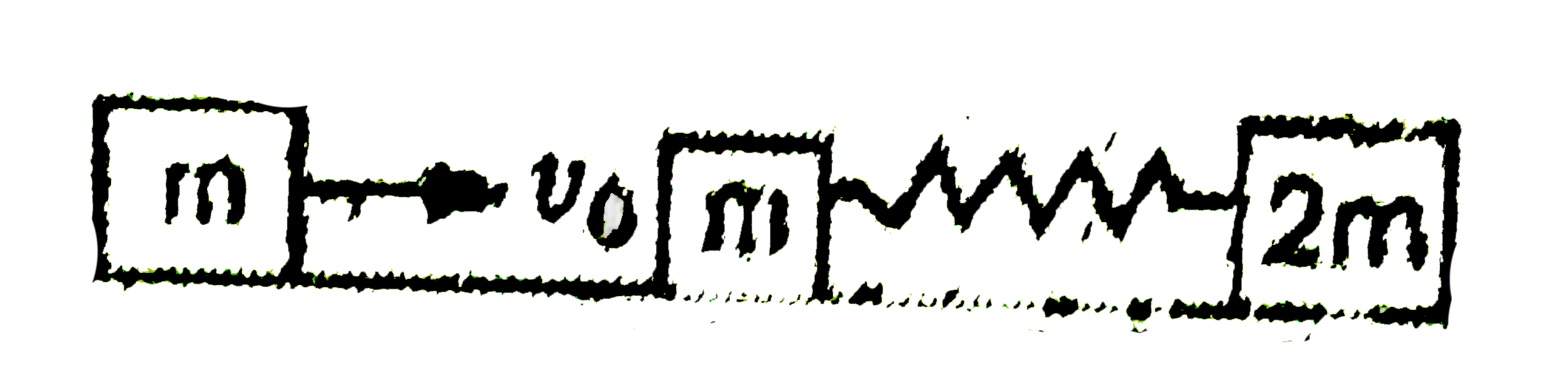 A√2m3kυ0B√3m2kυ0C√m2kυ0D√mkυ0
A√2m3kυ0B√3m2kυ0C√m2kυ0D√mkυ0Consider the system shown below, with two equal masses m and a spring with spring constant K. The coefficient of friction between the left mass and horizontal table is μ=1/4, and the pulley is frictionless. The spring connecting both the blocks is massless and inelastic. The system is held with the spring at its unstretched length and then released.
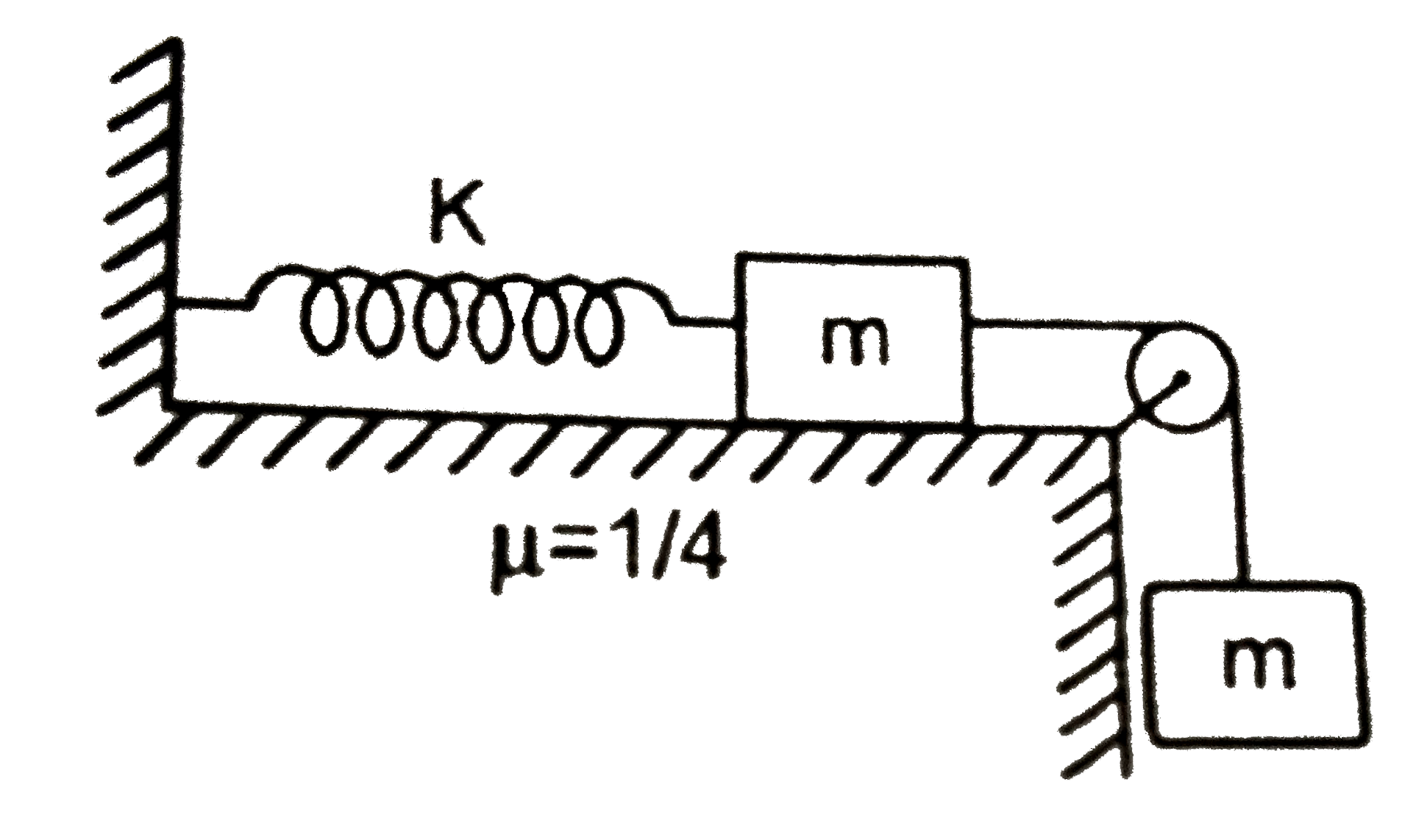
If the string connecting both the masses is cut just at the instant both masses came to momentary rest for the first time in question 5, then maximum compression of spring during resulting motion is (Take μ=1/4)A2mg3KBmg2KCmgKD1mg3KA particle of mass m collides elastically with the pan of mass (M = 2m) of a spring balance, as shown in figure. Pan is in equillibrium before collision. Spring constant is k and speed of the particle before collision is v0 . Answer the following three questions regarding this collision.
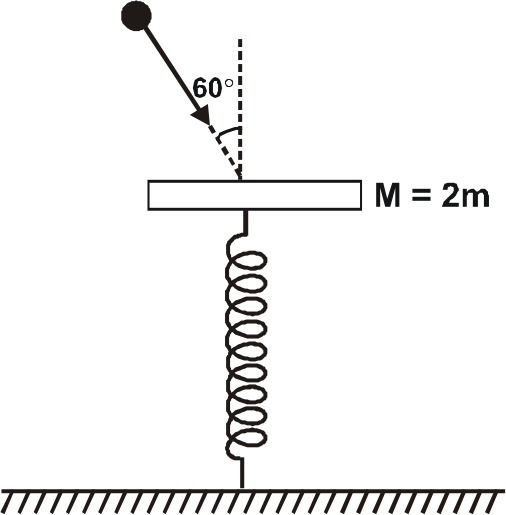
Maximum compression in the spring after the collision theA√2mkv03B√2m3kv0C√m3kv0D√mkv0
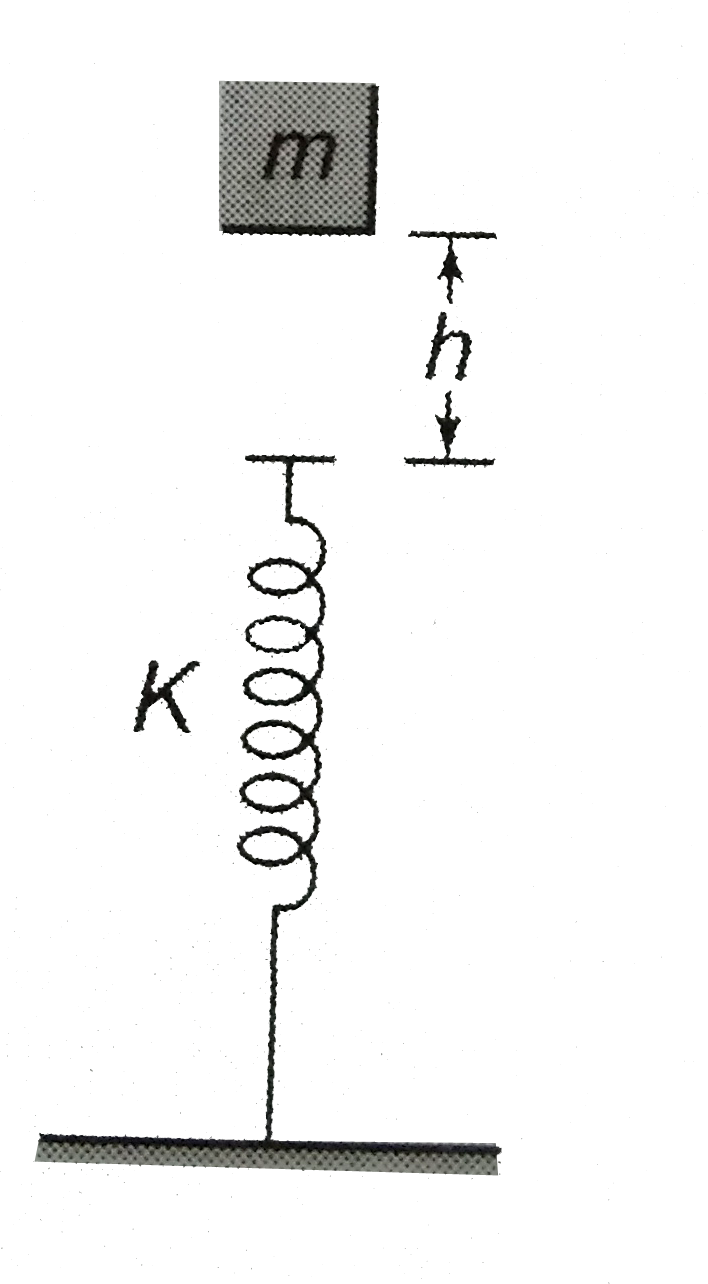
A block is released from height h, find the maximum compression of spring of spring constant k.
View SolutionExercise Questions|Combination Of Spring|Parallel Combination Of Spring|Series Combination Of Spring
View SolutionConsider Example 22. Takin the coefficient of friction, μ to be 0.5, calculate the maximum compression of the spring.
View SolutionIf blocks collide elastically head - on , the ratio of maximum compression of the left spring and the right spring will be
View SolutionA block of mass m attached with a spring and held by a person such that the spring is in natural length l0. Now the man releases the block, the ratio of maximum compression in the spring in the given situation , to that of the compression at equilibrium position.

View Solution