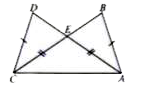
In figure AB=CD,∠PAC=∠QBD, Prove that ΔAPC≅ΔBQD

Video Solution
|
Answer
Step by step video & image solution for In figure AB=CD, anglePAC= angleQBD, Prove that DeltaAPC~=DeltaBQD by Maths experts to help you in doubts & scoring excellent marks in Class 9 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
Knowledge Check
In the figure, AB||CD then
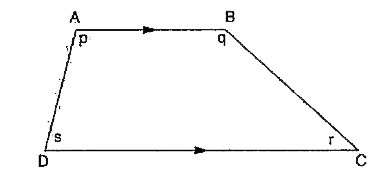 Ap+r=q+sBp−r=q−sCp+s=q+rDp−q=s−r
Ap+r=q+sBp−r=q−sCp+s=q+rDp−q=s−r
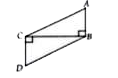
In the given figure, Ab = CD and ∠ABC=∠DCB=90∘. Prove that AC = DB.
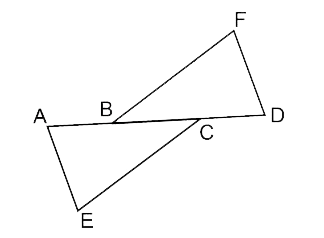
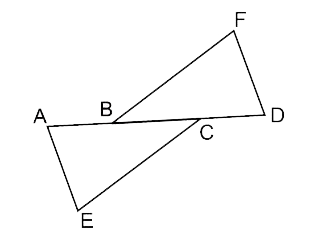
View SolutionIn the given figure , AB=CD,CE=BF and ∠ACE=∠DBF . Prove that
△ACE≅△DBF
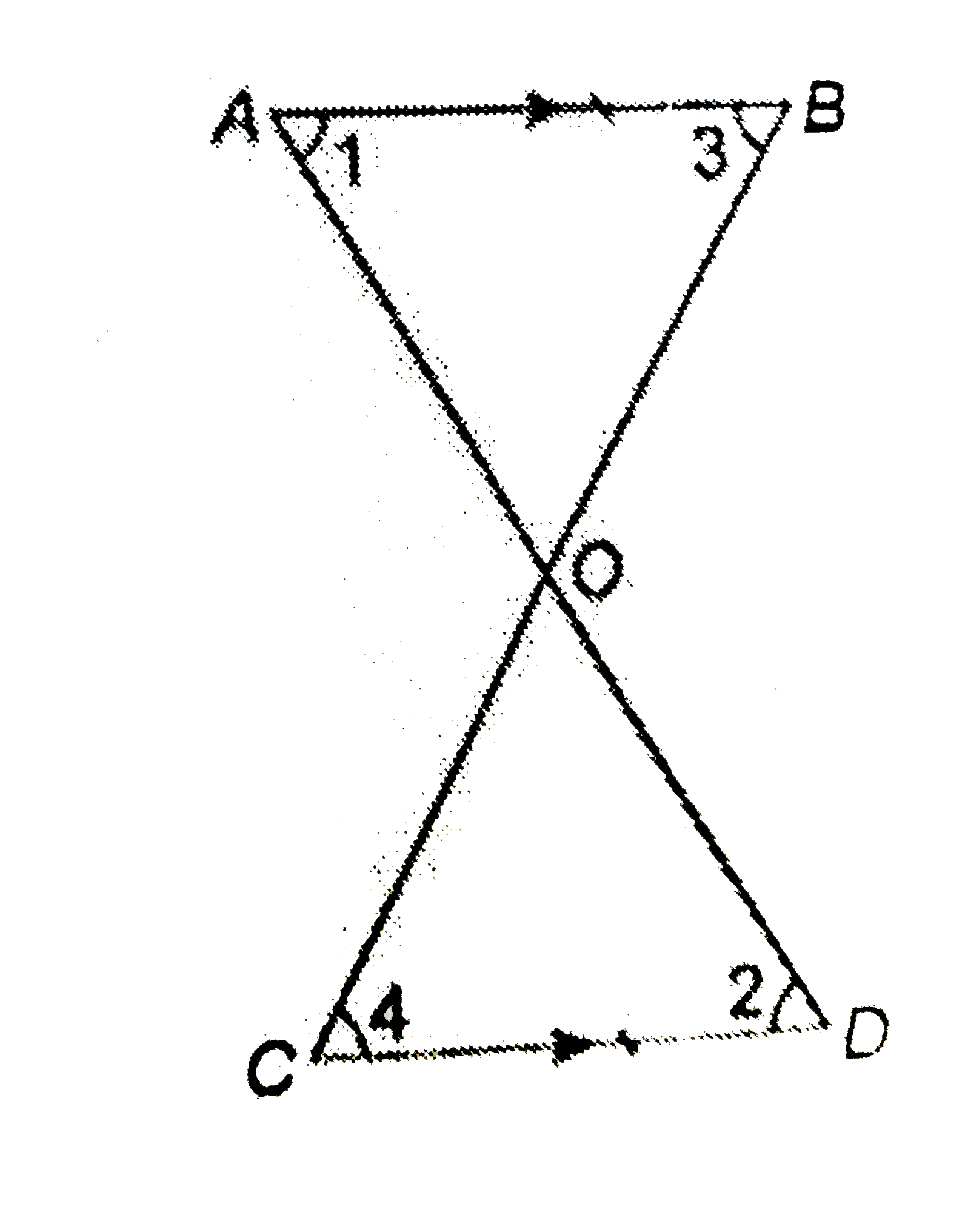
View SolutionIn the adjoining figure, AB = CD and AB||CD prove that
(i) ΔAOB≅ΔDOC
(ii) AD and BC bisect each other at the point O.
View SolutionIn the given figure , AB=CD,CE=BF and ∠ACE==∠DBF . Prove that
AE=DF
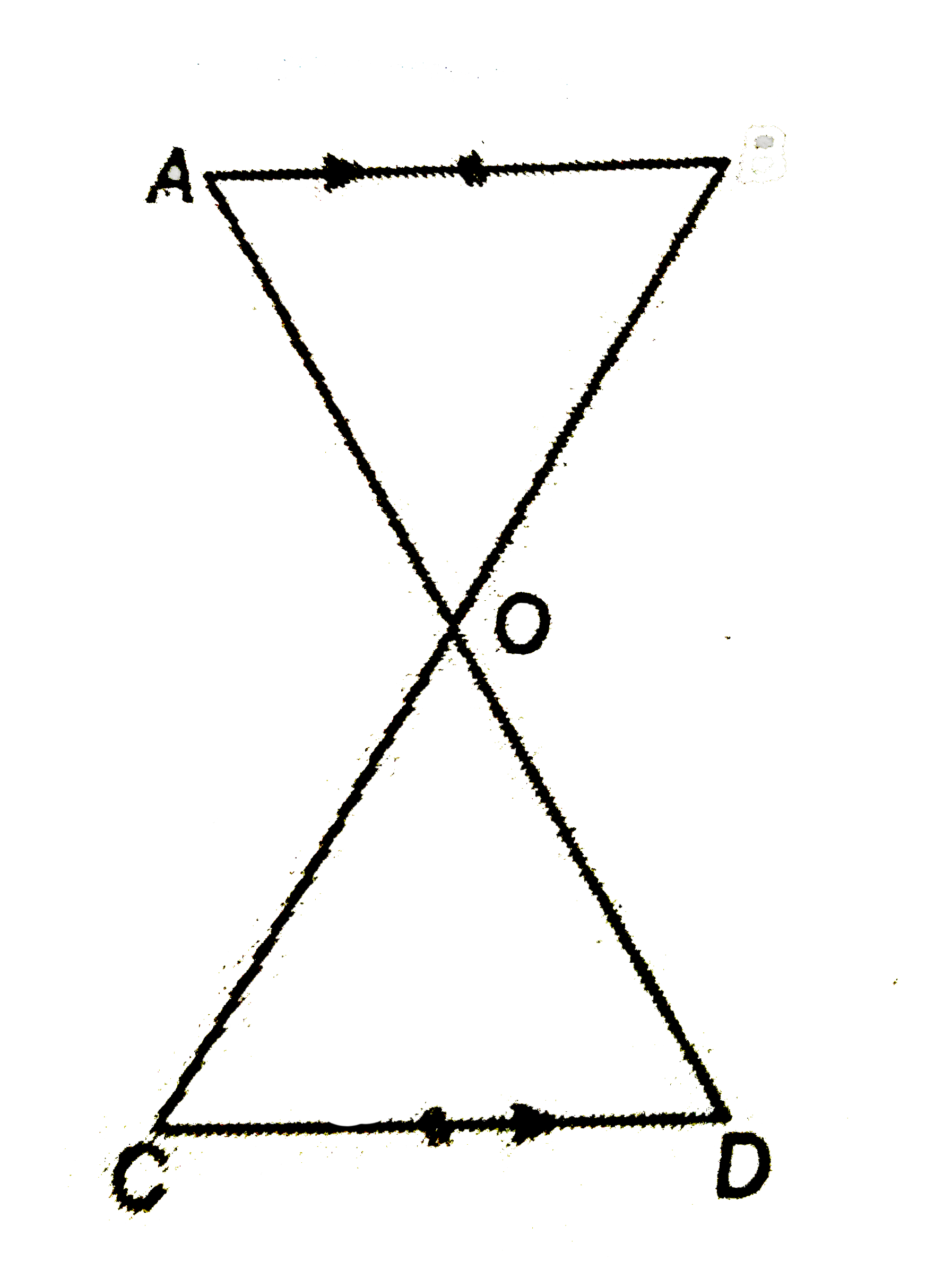
View SolutionIn the adjoining figure AB=CD and AB||CD prove that :
(i) ΔAOB≅ΔDOG
(ii) AD and BC bisect each other at point O.
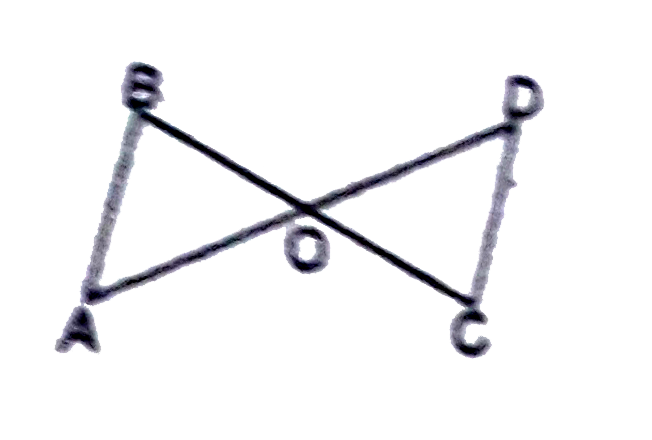
View SolutionIn the given figure △AOB~△DOC, prove that AB||CD.
View SolutionIn Figure, if AB=AC and BE=CD, prove that AD=AE.
View Solution