In the figure, ABC is an isosceles triangle in which AB = AC and LM is parallel to BC. If ∠A=50∘ find ∠LMC.
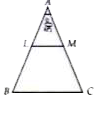
The correct Answer is:115∘
Step by step video, text & image solution for In the figure, ABC is an isosceles triangle in which AB = AC and LM is parallel to BC. If angleA= 50^(@) find angleLMC. by Maths experts to help you in doubts & scoring excellent marks in Class 9 exams.
Updated on:21/07/2023Topper's Solved these Questions
- TRIANGLESBook:MTG IIT JEE FOUNDATIONChapter:TRIANGLESExercise:EXERCISE (Multiple Choice Questions) (LEVEL-2 )
Explore 10 Videos
- TRIANGLESBook:MTG IIT JEE FOUNDATIONChapter:TRIANGLESExercise:EXERCISE (Multiple Choice Questions) (MATCH THE FOLLOWING)
Explore 2 Videos
- TRIANGLESBook:MTG IIT JEE FOUNDATIONChapter:TRIANGLESExercise:NCERT SECTION (Exercise 7.5)
Explore 4 Videos
- STATISTICSBook:MTG IIT JEE FOUNDATIONChapter:STATISTICSExercise:Olympiad/HOTS Corner
Explore 20 Videos
Similar Questions
In the given figure (not to scale), ABC is an isosceles triangle in which AB=AC. AEDC is a parallelogram. If ∠CDF=70∘ and ∠BFE=180∘, then find ∠FBA.
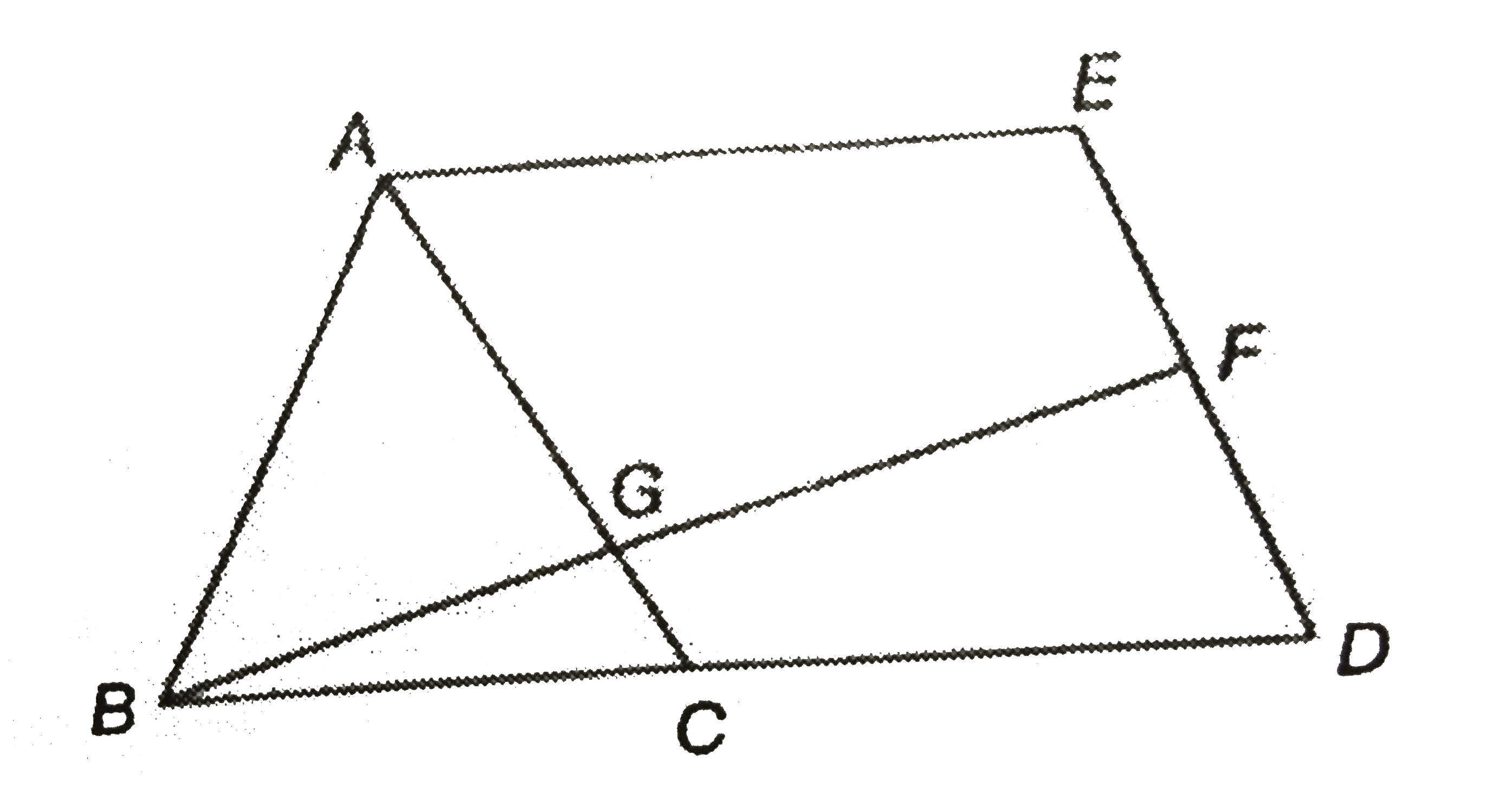
View SolutionIn the adjoining figure, ΔABC is an isosceles triangle in which AB = AC. Side CP is parallel to AB and AP is the bisector of exterior angle CAD of Δ ABC. Prove that ∠PAC=∠BCAand□ABCP is a parallelogram.
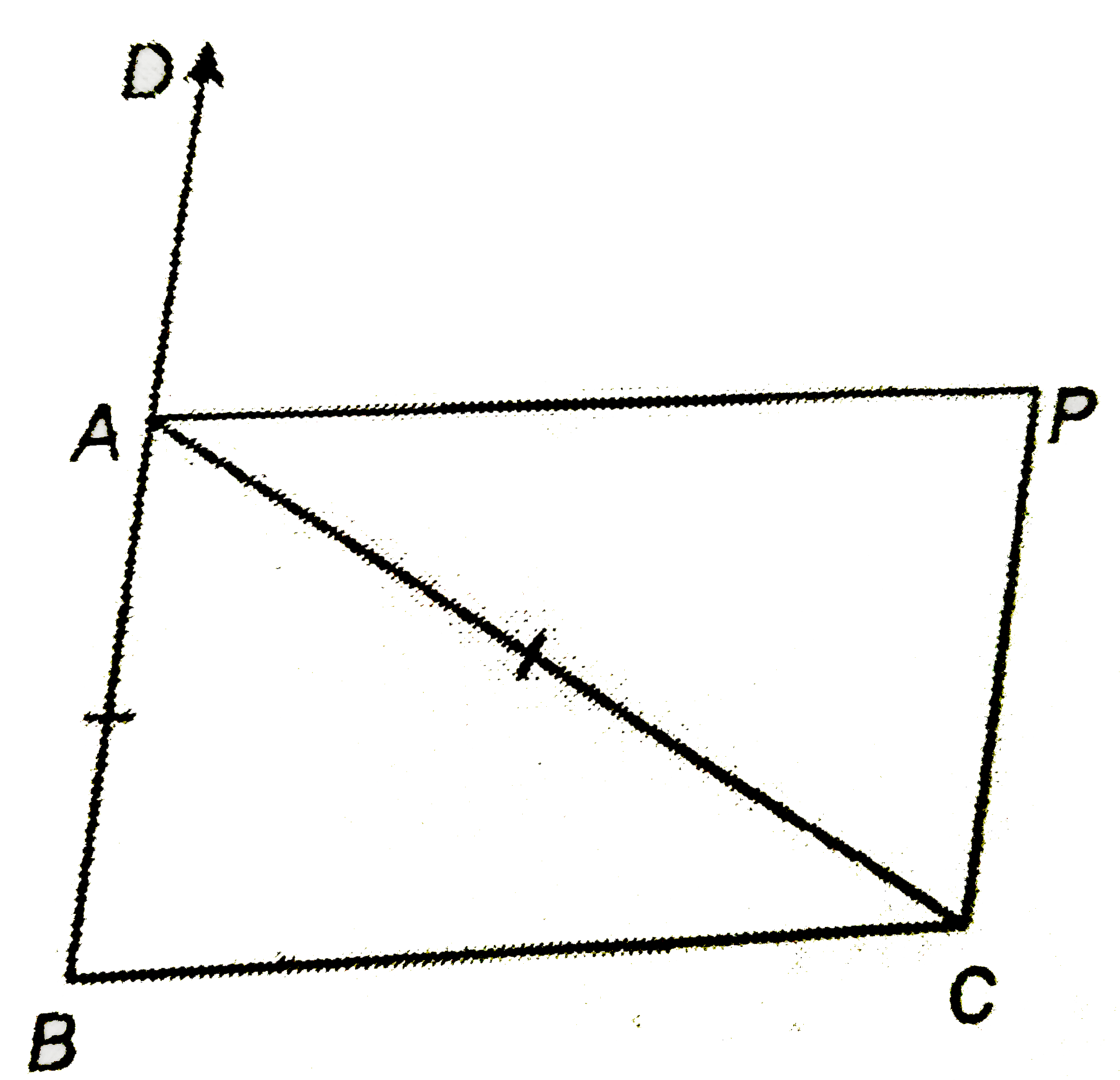
View Solution
Knowledge Check
In the given figure, ABC is an isosceles triangle in which AB=ACand∠ABC=50∘,∠BDC :
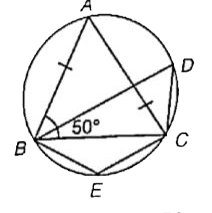 A80∘B60∘C65∘D100∘
A80∘B60∘C65∘D100∘In the given figure, △ABC is an isosceles triangle in which AB=AC and ∠ABC=50∘ , the ∠BDC is equal to
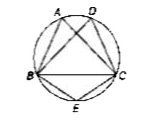 A60∘B10∘C80∘D90∘
A60∘B10∘C80∘D90∘
In the adjoining figure, ΔABC is an isosceles triangle in which AB = AC and AD is the bisector of ∠A. Prove that:
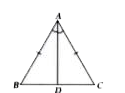
∠B=∠C
View SolutionIn the adjoining figure, ΔABC is an isosceles triangle in which AB = AC and AD is the bisector of ∠A. Prove that:
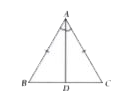
ΔADB≅ΔADC
View SolutionIn the adjoining figure, ΔABC is an isosceles triangle in which AB = AC and AD is the bisector of ∠A. Prove that:

BD = CD
View SolutionIn the adjoining figure, ΔABC is an isosceles triangle in which AB = AC and AD is the bisector of ∠A. Prove that:
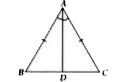
AD⊥BC
View SolutionIn the given figure, ΔABC is an isosceles triangle in which AB=AC and AE bisects ∠CAD. Prove that AE∣∣BC.
View SolutionABC is an isosceles triangle with AB = AC. Draw AP⊥BC to show that ∠B=∠C.
View Solution
