Question

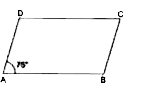
ABCD is a parallelogram in which $\angle A={78}^{\circ }$. Compute $\angle B,\angle C\text{and}\angle D$.

Explanation

To solve the problem, we need to find the measures of angles B, C, and D in the parallelogram ABCD, given that angle A = 78°.

1. Identify the properties of a parallelogram:
In a parallelogram, opposite angles are equal, and adjacent angles are supplementary (i.e., they add up to 180°).

2. Find angle C:
Since angle A is opposite to angle C, we have:
\( \text{Angle C} = \text{Angle A} = 78° \)

3. Find angle B:
Since angles A and B are adjacent angles, we can use the property that they sum to 180°:
\( \text{Angle A} + \text{Angle B} = 180° \)
Substituting the value of angle A:
\( 78° + \text{Angle B} = 180° \)
To find angle B, we subtract 78° from both sides:
\( \text{Angle B} = 180° - 78° = 102° \)

4. Find angle D:
Since angle B is opposite to angle D, we have:
\( \text{Angle D} = \text{Angle B} = 102° \)

Summary of Angles:
- Angle A = 78°
- Angle B = 102°
- Angle C = 78°
- Angle D = 102°

Final Answer:
- Angle B = 102°
- Angle C = 78°
- Angle D = 102°

---