In the figure ¯¯¯¯¯¯AB∣∣¯¯¯¯¯¯XY. BX and AY are the transversals intersecting at O, such that ¯¯¯¯¯¯OA=¯¯¯¯¯¯OY. Show that ΔOAB≅ΔOYX.

Step by step video & image solution for In the figure bar(AB)||bar(XY). BX and AY are the transversals intersecting at O, such that bar(OA)=bar(OY). Show that DeltaOAB~=DeltaOYX. by Maths experts to help you in doubts & scoring excellent marks in Class 7 exams.
Updated on:21/07/2023Topper's Solved these Questions
- CONGRUENCE OF TRIANGLES BOOK - MTG IIT JEE FOUNDATIONCHAPTER - CONGRUENCE OF TRIANGLES EXERCISE - Subjective Problems (Long Answer Type)
10 Videos
- CONGRUENCE OF TRIANGLES BOOK - MTG IIT JEE FOUNDATIONCHAPTER - CONGRUENCE OF TRIANGLES EXERCISE - Olympiad/HOTS Corner
10 Videos
- CONGRUENCE OF TRIANGLES BOOK - MTG IIT JEE FOUNDATIONCHAPTER - CONGRUENCE OF TRIANGLES EXERCISE - Subjective Problems (Very Short Answer Type)
10 Videos
- COMPARING QUANTITIES BOOK - MTG IIT JEE FOUNDATIONCHAPTER - COMPARING QUANTITIES EXERCISE - Olympaid/HOTS Corner
20 Videos
- DATA HANDLINGBOOK - MTG IIT JEE FOUNDATIONCHAPTER - DATA HANDLINGEXERCISE - Olympiad / Hots Corner
20 Videos
Similar Questions
Knowledge Check

In the figure above, ¯¯¯¯¯¯AB||¯¯¯¯¯¯CD. Find the value of x.A50∘B45∘C60∘D40∘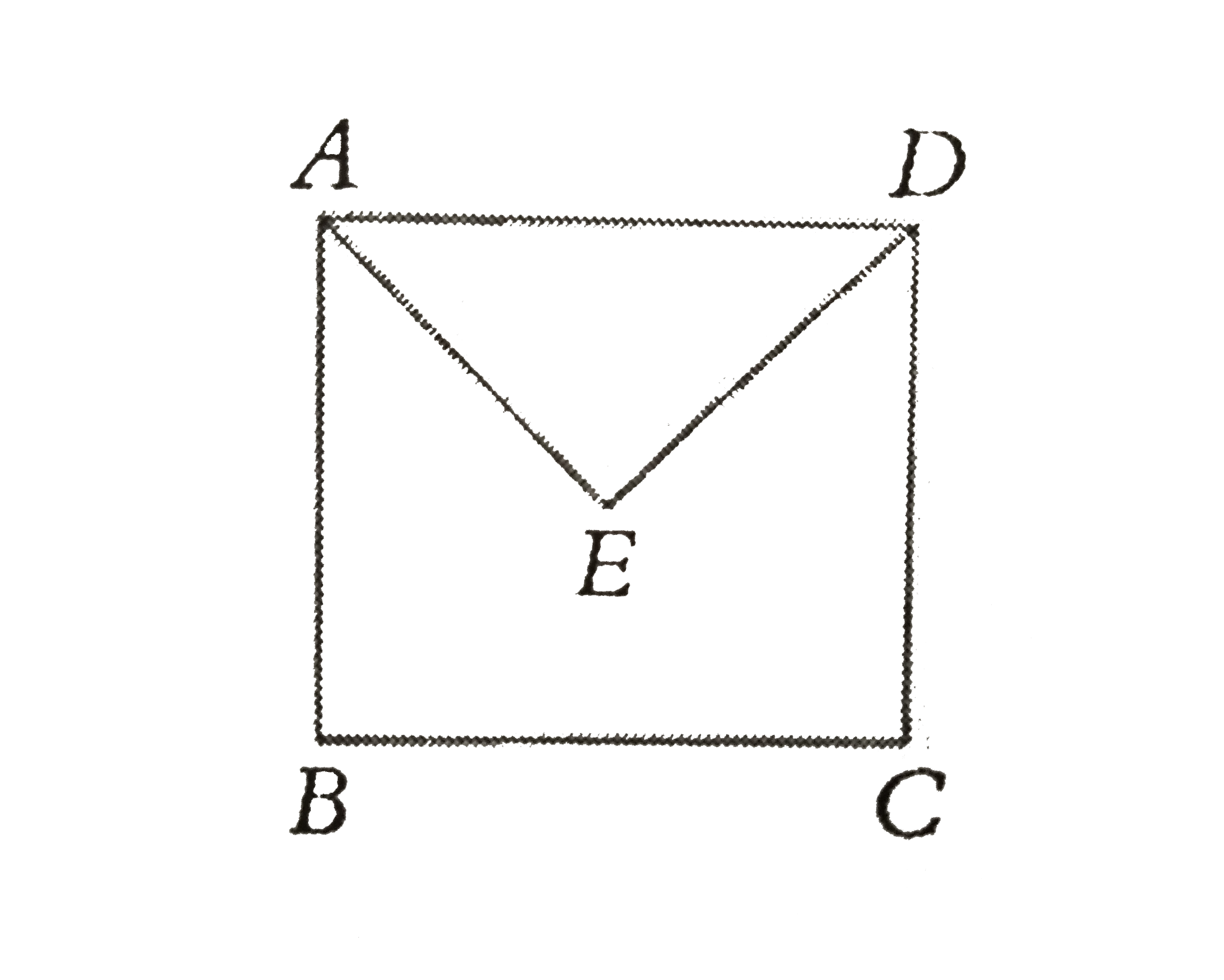
In the figure above, ¯¯¯¯¯¯AB∣∣¯¯¯¯¯¯CD,∠BAE=30∘ and ∠CDE=35∘. If ¯¯¯¯¯¯AB⊥¯¯¯¯¯¯BC, then find ∠AED.A60∘B55∘C65∘D85∘

In the given figure, ¯¯¯¯¯¯¯LM//¯¯¯¯¯¯PN and the line l is a transversal of ¯¯¯¯¯¯¯LMand¯¯¯¯¯¯PN. Find the value of a .
View Solution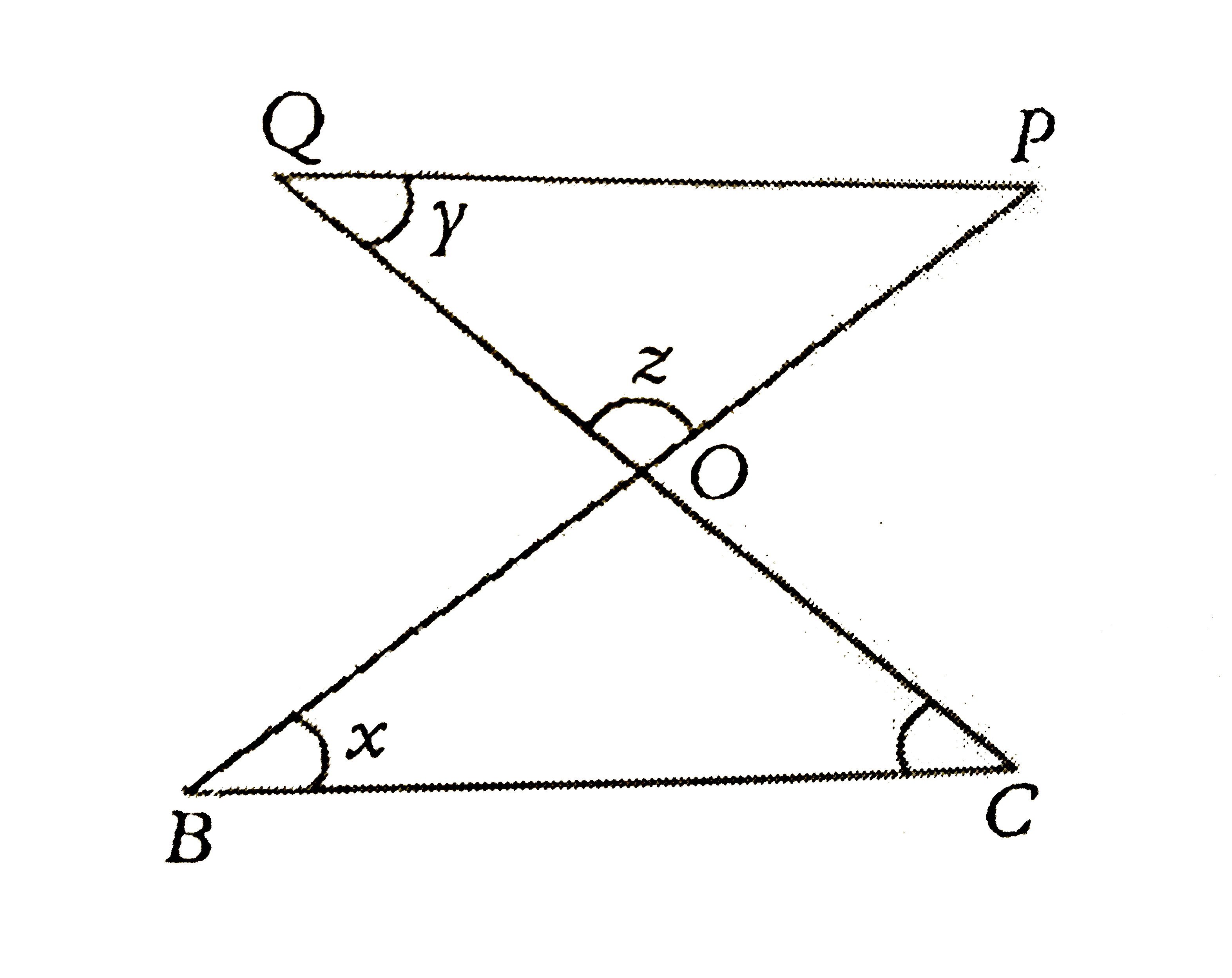
In the figure above, ¯¯¯¯¯¯BC∣∣¯¯¯¯¯¯PQ,¯¯¯¯¯¯BPand¯¯¯¯¯¯CQ intersect at O. If x+y=80∘andz−y=55∘, then find x and y.
View SolutionIn the given figure ¯¯¯¯¯¯AB=¯¯¯¯¯¯BC and ¯¯¯¯¯¯AC=¯¯¯¯¯¯CD if ∠BAD=y and ∠ADB=x then ∠y=
View Solution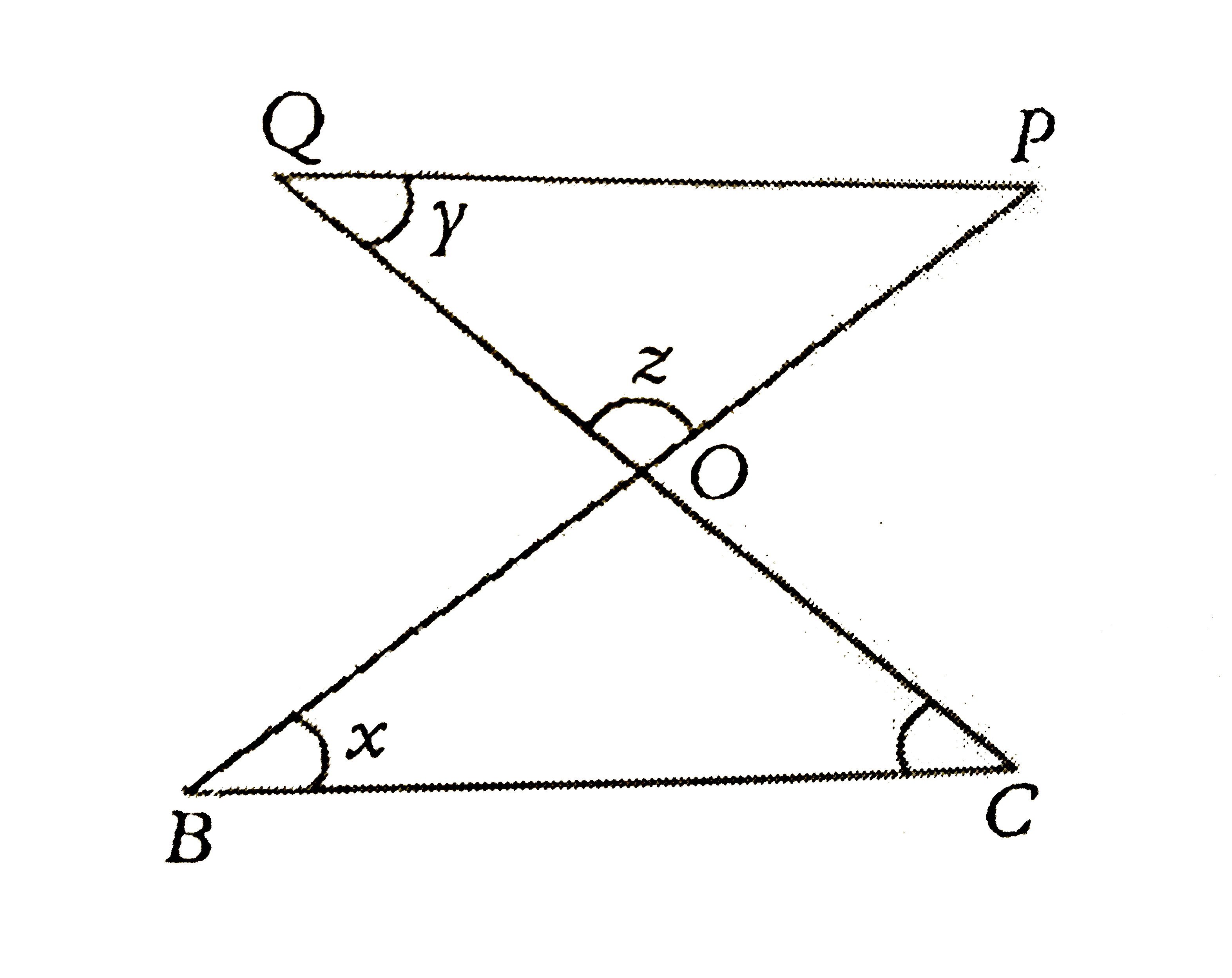
In the figure above, ¯¯¯¯¯¯BC∣∣¯¯¯¯¯¯PQ,¯¯¯¯¯¯BPand¯¯¯¯¯¯CQ intersect at O. If x+y=80∘andz−y=55∘, then find x and y.
View SolutionLet O be an interior point of ΔABC such that ¯¯¯¯¯¯OA+2¯¯¯¯¯¯OB+3¯¯¯¯¯¯OC=0. Then the ratio of a ΔABC to area of ΔAOC is
View SolutionIn the given figure if ¯¯¯AD=¯¯¯BD and ¯¯¯CD=¯¯¯AC ∠B=37∘ then ∠ACB=
View Solution
