ABCD is a rectangle and M is a point on CD . AC and BM meet at X .

It is given that CM =3MD. Find
(i) area of $Δ C X M$ : area of $Δ A X B$
(ii) area of $Δ B X C$ : area of rectangle ABCD

A

(i) $9 : 16$
(ii) $3 : 11$

B

(i) $9 : 16$
(ii) $3 : 14$

C

(i) $16 : 9$
(ii) $14 : 3$

D

(i) $16 : 9$
(ii) $11 : 3$

Text Solution

Verified by Experts

The correct Answer is:B

Updated on:21/07/2023

|

Class 10 MATHS TRIANGLES

Topper's Solved these Questions

TRIANGLES BOOK - MTG IIT JEE FOUNDATIONCHAPTER - TRIANGLES EXERCISE - Exercise (Integer Numerical Value Type)
10 Videos
SURFACE AREAS AND VOLUMESBOOK - MTG IIT JEE FOUNDATIONCHAPTER - SURFACE AREAS AND VOLUMESEXERCISE - OLYMPIAD/HOTS CORNER
20 Videos

Similar Questions

Knowledge Check

ABCD is a rectangle and there are four equilateral triangles . Area of $Δ A S D$ equals to area of $Δ B Q C$ and area of $Δ D R C$ equals to area of $Δ$ APB. The perimeter of the rectangle is 12 cm. Also the sum of the areas of the four triangles is $10 \sqrt{3} c m^{2}$ then the total area of the figures thus formed :

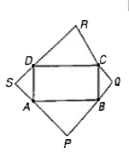A $2 (4 + 5 \sqrt{3}) c m^{2}$
B $5 (4 + 2 \sqrt{3}) c m^{2}$
C $42 \sqrt{3}$
Dnone of these
In the figure, ABCD is a rectangle, and F and E are points on AB and BC respectively. The area of $Δ D F B$ is 9 and the area of $Δ B E D$ is 24. What is the perimeter of the rectangle?

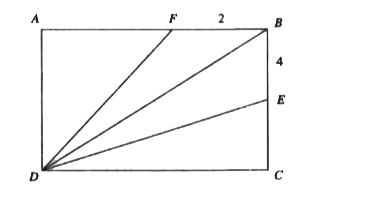A18
B23
C30
D42
In the figure, ABCD is a rectangle, and the area of $Δ A C E$ is 10. What is the area of the rectangle?

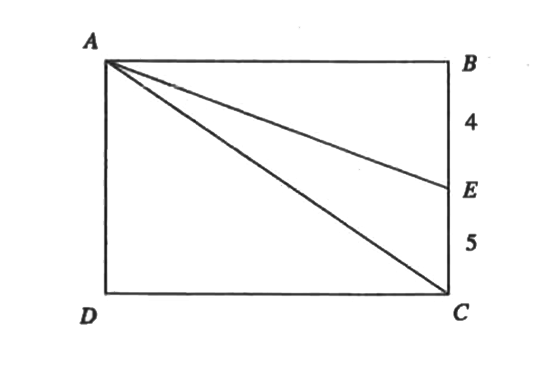A18
B22.5
C36
D44

MTG IIT JEE FOUNDATION-TRIANGLES -Olympiad /HOTS Corner

Home
Ask Doubt
Profile