ABCD is a rectangle. P & Q are two points on AB, such that AP : PQ : QB = 3 : 4 : 5. Find the ratio of area ΔPQC and rectangle ABCD.
The correct Answer is:1:6
To solve the problem, we need to find the ratio of the area of triangle PQC to the area of rectangle ABCD. Let's break it down step by step.
Step 1: Understand the Ratios
We are given that the segments AP, PQ, and QB are in the ratio 3:4:5. Let's denote:
- AP = 3x
- PQ = 4x
- QB = 5x
Step 2: Calculate the Length of AB
The total length of AB can be calculated by adding the lengths of AP, PQ, and QB:
AB=AP+PQ+QB=3x+4x+5x=12x
Step 3: Identify the Height of the Triangle and Rectangle
Let the height of the rectangle (and triangle) from line AB to line CD be denoted as h (which is the same for both the triangle and rectangle).
Step 4: Calculate the Area of Triangle PQC
The base of triangle PQC is PQ, which is 4x. The height is h. Therefore, the area of triangle PQC can be calculated using the formula for the area of a triangle:
Area of △PQC=12×base×height=12×4x×h=2xh
Step 5: Calculate the Area of Rectangle ABCD
The area of rectangle ABCD is given by the formula:
Area of rectangle ABCD=length×height=AB×h=12x×h=12xh
Step 6: Find the Ratio of the Areas
Now we can find the ratio of the area of triangle PQC to the area of rectangle ABCD:
Ratio=Area of △PQCArea of rectangle ABCD=2xh12xh
Here, xh cancels out:
Ratio=212=16
Final Answer
The ratio of the area of triangle PQC to the area of rectangle ABCD is:
Ratio=1:6
We are given that the segments AP, PQ, and QB are in the ratio 3:4:5. Let's denote:
- AP = 3x
- PQ = 4x
- QB = 5x
The total length of AB can be calculated by adding the lengths of AP, PQ, and QB:
AB=AP+PQ+QB=3x+4x+5x=12x
Let the height of the rectangle (and triangle) from line AB to line CD be denoted as h (which is the same for both the triangle and rectangle).
The base of triangle PQC is PQ, which is 4x. The height is h. Therefore, the area of triangle PQC can be calculated using the formula for the area of a triangle:
Area of △PQC=12×base×height=12×4x×h=2xh
The area of rectangle ABCD is given by the formula:
Area of rectangle ABCD=length×height=AB×h=12x×h=12xh
Now we can find the ratio of the area of triangle PQC to the area of rectangle ABCD:
Ratio=Area of △PQCArea of rectangle ABCD=2xh12xh
Here, xh cancels out:
Ratio=212=16
The ratio of the area of triangle PQC to the area of rectangle ABCD is:
Ratio=1:6
Topper's Solved these Questions
- BOOK - ADVANCED MATHS BY ABHINAY MATHS ENGLISHCHAPTER - QUADRILATERALEXERCISE - EXERCISE48videos
QUADRILATERAL
- BOOK - ADVANCED MATHS BY ABHINAY MATHS ENGLISHCHAPTER - PROFIT & LOSSEXERCISE - Questions200videos
PROFIT & LOSS
- BOOK - ADVANCED MATHS BY ABHINAY MATHS ENGLISHCHAPTER - RATIO & PROPORTIONEXERCISE - QUESTIONS113videos
RATIO & PROPORTION
Similar Questions
Knowledge Check
- Question 1 - Select One
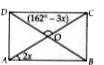
ABCD is a rectangle. Find x.
 A54∘B36∘C24∘D18∘
A54∘B36∘C24∘D18∘ - Question 2 - Select One
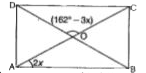
If ABCD is a rectangle, find the values of x and y:
 Ax = 10, y = 2Bx = 12 y = 8Cx= 2, y = 10Dx = 20, y = 0
Ax = 10, y = 2Bx = 12 y = 8Cx= 2, y = 10Dx = 20, y = 0
If ABCD is a rectangle , find the values of x and y
 View Solution
View SolutionABCD is a rectangle . P and Q are two point on AB and AD s.t. area of ΔAPQ,ΔPBCand∠COQ are equal If BP = 2 cm. Find AP
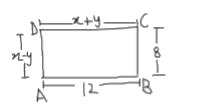
View SolutionWhat is the area of rectangle ABCD?
 View Solution
View SolutionABCD is a rectangle. Let E be a point on
AB and F a point on CD such that DE is
parallel to BF.If AE=3 cm and if the area
of triangle BFC = 6 square cm.
Consider the following statements :
1. Area of rectangle ABCD can be of the
form pq2 square cm where p,q are
distinct primes.
2. Area of the figure EBFD is of the form
r2 square cm where r is rational but not an integer.
Which of the above statements is/are correct ?View SolutionIn the given figure, if ABCD is a rectangle and P, Q are the mid-points of AD, DC respectively. then, the ratio of lengths PQ and AC is equal to
View SolutionIn a figure, ABCD is a rectangle and P & Q are mid-points of CD and BC respectively. Find ratio of area of shadow region and area of un-shad-owed region?
 View Solution
View Solution