the 35 kilogram girl is standing on a 20 kilogram wagon and jumps off. Giving the wagon a kicks that sends it off at 3.8 metres per second. How fast is the girl moving just after the jump off
1.2ms−1
3ms−1
4ms−1
2.2ms−1
The correct Answer is:D
To solve the problem, we will use the principle of conservation of momentum. Here are the steps to find the speed of the girl just after she jumps off the wagon:
1. Identify the Given Data:
- Mass of the girl (Mg) = 35 kg
- Mass of the wagon (Mw) = 20 kg
- Velocity of the wagon after the jump (Vw) = 3.8 m/s
2. Understand the Conservation of Momentum:
- According to the law of conservation of momentum, the total momentum before the jump must equal the total momentum after the jump.
- Initial momentum (before the jump) = 0 (since both the girl and the wagon are at rest).
- Final momentum (after the jump) = Momentum of the girl + Momentum of the wagon.
3. Set Up the Equation:
- Let the velocity of the girl after jumping off be Vg.
- The momentum of the girl after jumping off = Mg * Vg.
- The momentum of the wagon after the jump = Mw * Vw.
- Since the initial momentum is zero, we can write:
Mg⋅Vg+Mw⋅Vw=0
4. Rearranging the Equation:
- This can be rearranged to find Vg:
Mg⋅Vg=−Mw⋅Vw
- Thus, we have:
Vg=−Mw⋅VwMg
5. Substituting the Values:
- Substitute the values of Mw, Vw, and Mg into the equation:
Vg=−20kg⋅3.8m/s35kg
6. Calculating Vg:
- Calculate the value:
Vg=−7635≈−2.17m/s
- Since we are interested in the speed (magnitude), we take the absolute value:
Vg≈2.17m/s
7. Final Answer:
- The speed of the girl just after she jumps off is approximately 2.17 m/s.
- Mass of the girl (Mg) = 35 kg
- Mass of the wagon (Mw) = 20 kg
- Velocity of the wagon after the jump (Vw) = 3.8 m/s
- According to the law of conservation of momentum, the total momentum before the jump must equal the total momentum after the jump.
- Initial momentum (before the jump) = 0 (since both the girl and the wagon are at rest).
- Final momentum (after the jump) = Momentum of the girl + Momentum of the wagon.
- Let the velocity of the girl after jumping off be Vg.
- The momentum of the girl after jumping off = Mg * Vg.
- The momentum of the wagon after the jump = Mw * Vw.
- Since the initial momentum is zero, we can write:
Mg⋅Vg+Mw⋅Vw=0
- This can be rearranged to find Vg:
Mg⋅Vg=−Mw⋅Vw
- Thus, we have:
Vg=−Mw⋅VwMg
- Substitute the values of Mw, Vw, and Mg into the equation:
Vg=−20kg⋅3.8m/s35kg
- Calculate the value:
Vg=−7635≈−2.17m/s
- Since we are interested in the speed (magnitude), we take the absolute value:
Vg≈2.17m/s
- The speed of the girl just after she jumps off is approximately 2.17 m/s.
Topper's Solved these Questions
- BOOK - MTG IIT JEE FOUNDATIONCHAPTER - FORCE AND LAWS OF MOTIONEXERCISE - EXERCISE (MATCH THE FOLLOWING )2videos
FORCE AND LAWS OF MOTION
- BOOK - MTG IIT JEE FOUNDATIONCHAPTER - FORCE AND LAWS OF MOTIONEXERCISE - EXERCISE (ASSERTION AND REASON )10videos
FORCE AND LAWS OF MOTION
- BOOK - MTG IIT JEE FOUNDATIONCHAPTER - FORCE AND LAWS OF MOTIONEXERCISE - EXERCISE MULTIPLE CHOICE QUESTIONS1videos
FORCE AND LAWS OF MOTION
- BOOK - MTG IIT JEE FOUNDATIONCHAPTER - FOOTSTEPS TOWARDS NEETEXERCISE - Multiple Choice Question45videos
FOOTSTEPS TOWARDS NEET
- BOOK - MTG IIT JEE FOUNDATIONCHAPTER - GRAVITATION EXERCISE - Olympiad/HOTS Corner20videos
GRAVITATION
Similar Questions
A 1.2 kilogram basketball travelling at 7.5 metres per second hits the back of a 12 kilogram wagon and bounces off a 3.8 meters per second, sending the wagon off in the original direction of travel of the ball. How fast in the wagon going.
View SolutionWhile moving in a wagon (5kg)along a smoth road at 0.5 m/s, a 15 kg boy throws a 3 kg bag of send in front of him with a speed 4 m/s relative to his original motion. How fast is he moving after he throws the bag of send. What is his final direction of motion ?
View Solution
Knowledge Check
- Question 1 - Select One
Two persons, A of mass 60kg and B of mass 40kg, are standing on a horizontal platform of mass 50kg. The platform is supported on wheels on a horizontal frictionless surface and is initially at rest. Consider the following situations.
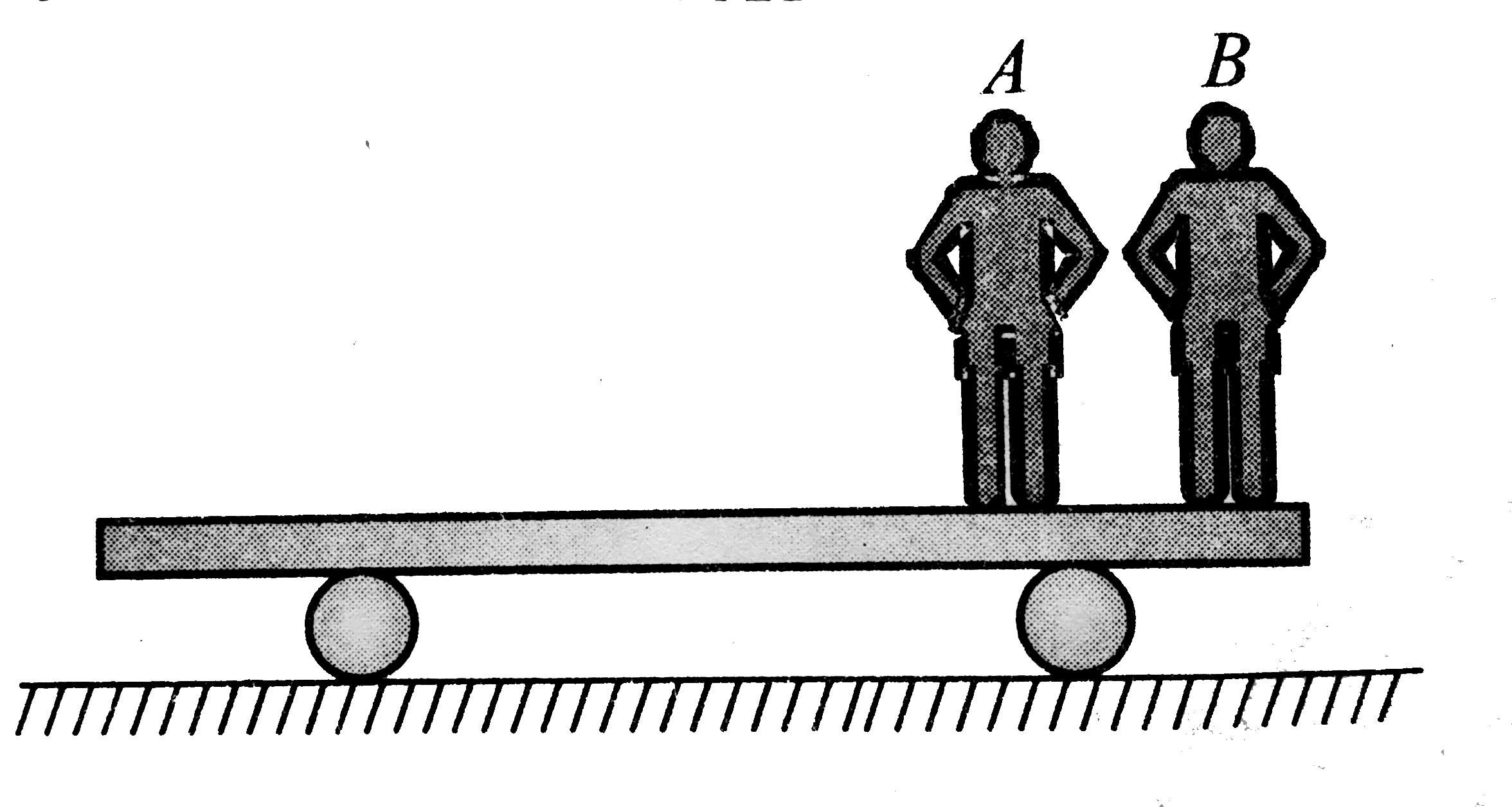
(i) Both A and B jump from the platform simultaneously and in the same horizontal direction.
(ii) A jumps first in a horizontal direction and after a few seconds B also jumps in the same direction. In both the situations above, just after the jump, the person (A or B) moves away from the platform with a speed of 3m/s relative to the platform and along the horizontal.
Final speed of the platform in situation (ii), i.e., just after B has jumped, will be nearlyA7.5m/sB5.5m/sC4.5m/sD2.5m/s - Question 2 - Select One
Two persons, A of mass 60kg and B of mass 40kg, are standing on a horizontal platform of mass 50kg. The platform is supported on wheels on a horizontal frictionless surface and is initially at rest. Consider the following situations.
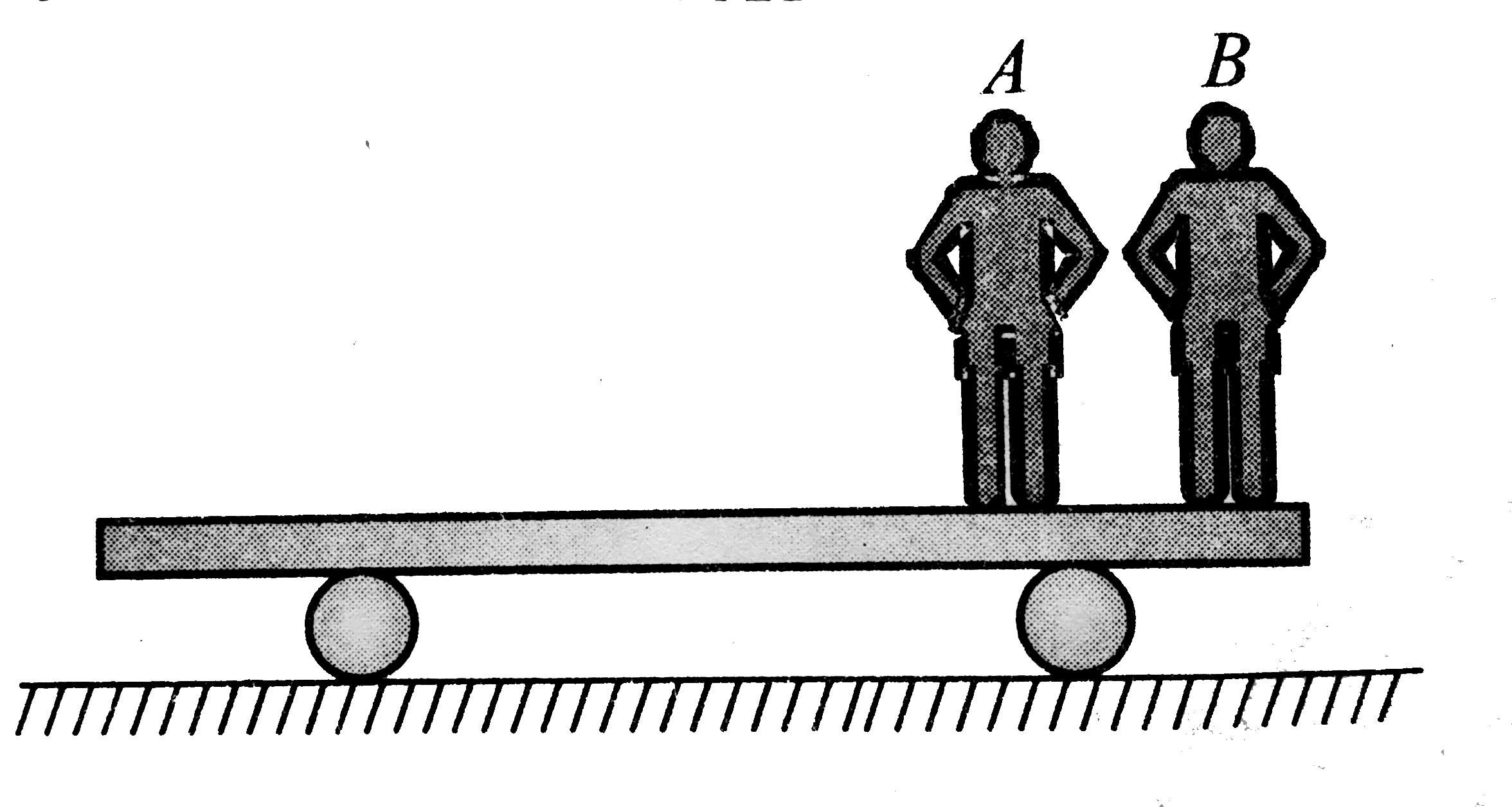
(i) Both A and B jump from the platform simultaneously and in the same horizontal direction.
(ii) A jumps first in a horizontal direction and after a few seconds B also jumps in the same direction. In both the situations above, just after the jump, the person (A or B) moves away from the platform with a speed of 3m/s relative to the platform and along the horizontal.
Final speed of the platform in situation (i), i.e., just after both A and B have jumped will be a.A2m/sB6m/sC5m/sDnone of these - Question 3 - Select One
Two persons, A of mass 60kg and B of mass 40kg, are standing on a horizontal platform of mass 50kg. The platform is supported on wheels on a horizontal frictionless surface and is initially at rest. Consider the following situations.
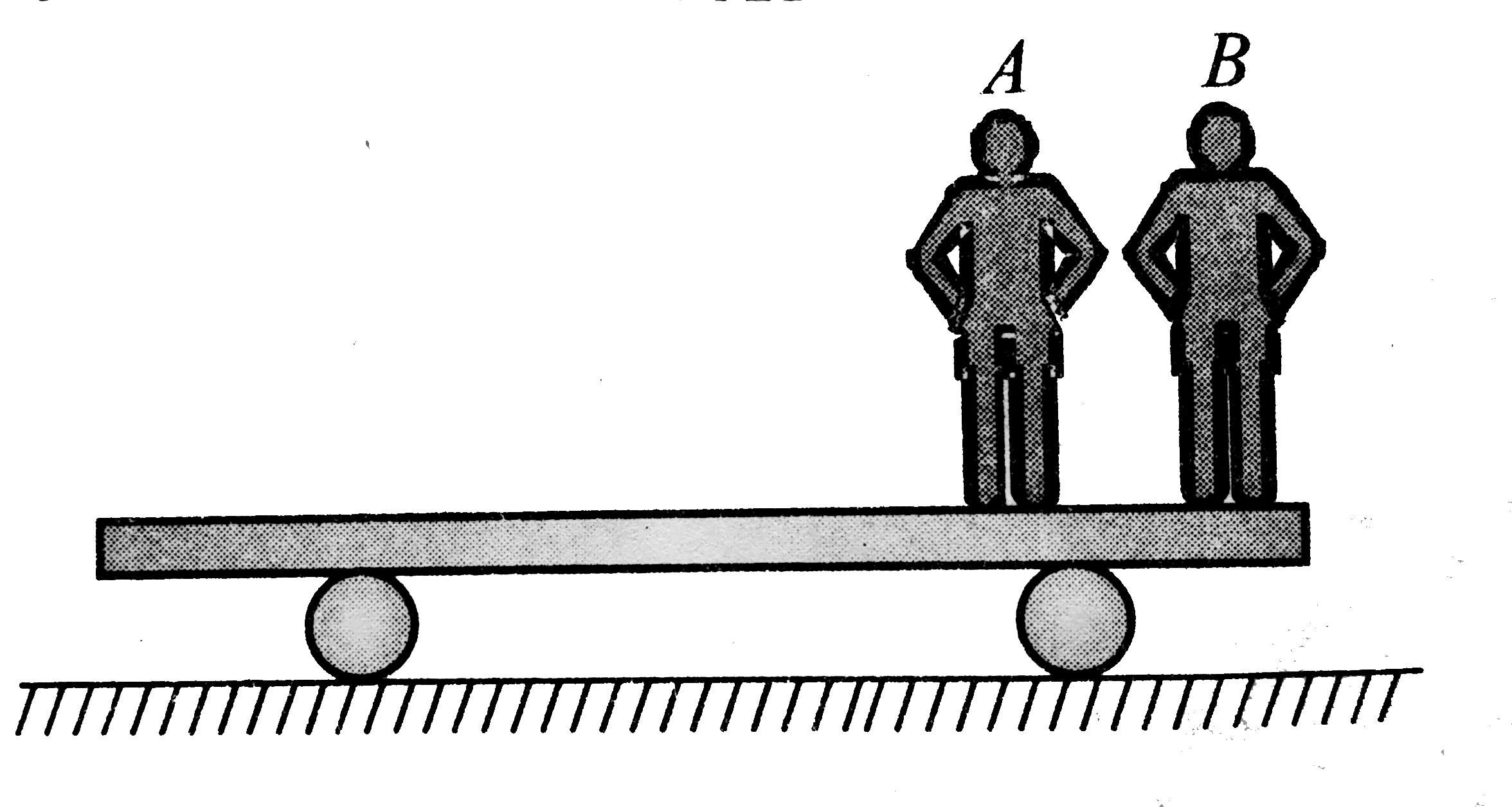
(i) Both A and B jump from the platform simultaneously and in the same horizontal direction.
(ii) A jumps first in a horizontal direction and after a few seconds B also jumps in the same direction. In both the situations above, just after the jump, the person (A or B) moves away from the platform with a speed of 3m/s relative to the platform and along the horizontal.
In situation (i), just after both A and B jump from the platform, velocity of centre of mass of the system (A,B and the platform) isA2m/sB6m/sC5m/sDnone of these
A buggy of mass 100 kg is free to move on a frictionless horizontal track. Two men, each of mass 50 kg, are standing on the buggy, which is initially stationary. The men jump off the buggy with velocity =10m/s relative to the buggy. In one situation, the men jump one after the other. in another situation, the men jump simultaneously. what is the ratio of the velocities of the buggy in two cases?
View Solutiona. A rail road flat car of mass M can roll without friction along a straight horizontal track . Initially, a man of mass m is standing on the car which is moving to the right with speed v0. What is the change in velocity of the car if the man runs to the left so that his speed relative to the car is vrel just before he jumps off at the left end?
b. If there are n men each of mass m on the car, should they all run and jump off together or should they run and jump one by one in order to give a greater velocity to the car?
 View Solution
View SolutionA submarine that is not moving sends out a sonar sound wave travelling at 2000 ms is, which reflects off a boat back to the submarine. The sonar crew detects the reflected wave 8 s after it was sent out. How far away is the boat from the submarine ?
View SolutionTwo electric trains run at the same speed of v=90kmhr along a straight track one after the other with an interval of l=2.0km between them. At the instant when they are located symmetrically relative to point A at a distance of b=1.0km from the track (figure) both trains give a brief sound signal of the same frequency of v=50Hz. What will the number of beats beard per second at point (A) when the vibrations produced by the signals arrive at it? The speed of sound is v=350 m/ s. Round off to nearest integer.
'(##CEN_KSR_PHY_JEE_C17_E01_014_Q03##)'View SolutionAn inquistive student, determined to test the law of gravity for himself, walks to the top of a building og 145 floors, with every floor of height 4 m, having a stopwatch in his hand (the first floor is at a height of 4 m from the ground level). From there he jumps off with negligible speed and hence starts rolling freely. A rocketeer arrives at the scene 5 s later and dives off from the top of the building to save the student. The rocketeer leaves the roof with an initial downward speed v0. In order to catch the student at a sufficiently great height above ground so that the rocketeer and the student slow down and arrive at the ground with zero velocity. The upward acceleration that accomplishes this is provided by rocketeer's jet pack, which he turns on just as he catches the student, before the rocketeer is in free fall. To prevent any discomfort to the student, the magnitude of the acceleration of the rocketeer and the student as they move downward together should not exceed 5 g.
Just as the student starts his free fall, he presses the button of the stopwatch. When he reaches at the top of 100th floor, he has observed the reading of stopwatch as 00:00:06:00 (hh:mm:ss:100 th part of the second). Find the value of g. (correct upt ot two decimal places).View Solution

