A cubical block of mass 2 kg with each side of 2 cm is lying on the ground. What will be the pressure exerted by the block on the ground?
(Take g=9.8m/s2)
To find the pressure exerted by the cubical block on the ground, we can follow these steps:
Step 1: Calculate the Area of the Base of the Cubical Block
The area A of the base of the cubical block can be calculated using the formula for the area of a square:
A=side×side
Given that each side of the cube is 2 cm:
A=2cm×2cm=4cm2
Step 2: Convert the Area from cm² to m²
To use SI units, we need to convert the area from square centimeters to square meters. We know that:
1m=100cm
Thus,
1m2=(100cm)2=10000cm2
To convert 4cm2 to m2:
A=4cm210000=4×10−4m2
Step 3: Calculate the Weight of the Block (Force)
The weight F of the block can be calculated using the formula:
F=m×g
Where:
- m=2kg (mass of the block)
- g=9.8m/s2 (acceleration due to gravity)
Calculating the force:
F=2kg×9.8m/s2=19.6N
Step 4: Calculate the Pressure Exerted by the Block
Pressure P can be calculated using the formula:
P=FA
Substituting the values we found:
P=19.6N4×10−4m2
Calculating the pressure:
P=49000Pa(or Pascals)
Final Answer
The pressure exerted by the block on the ground is:
P=49000Pa
The area A of the base of the cubical block can be calculated using the formula for the area of a square:
A=side×side
Given that each side of the cube is 2 cm:
A=2cm×2cm=4cm2
To use SI units, we need to convert the area from square centimeters to square meters. We know that:
1m=100cm
Thus,
1m2=(100cm)2=10000cm2
To convert 4cm2 to m2:
A=4cm210000=4×10−4m2
The weight F of the block can be calculated using the formula:
F=m×g
Where:
- m=2kg (mass of the block)
- g=9.8m/s2 (acceleration due to gravity)
F=2kg×9.8m/s2=19.6N
Pressure P can be calculated using the formula:
P=FA
Substituting the values we found:
P=19.6N4×10−4m2
Calculating the pressure:
P=49000Pa(or Pascals)
The pressure exerted by the block on the ground is:
P=49000Pa
Similar Questions
Knowledge Check
The sides of rectangular plate of mass 20 kg is 5 m x 4 m placed on a horizontal table. The pressure exerted by the block on the table is (Take g=10ms2)
A10 PaB100 PaC20 PaD30 PaA block of mass 4 kg is kept on ground. The co-efficient of friction between the block and the ground is 0.80. an external force of magnitude 30 N is applied parallel to the ground. The resultant force exerted by the ground on the block is (g=10m/x2)
A40NB30NC0ND50N
A person weighs 60 kg. The area under the foot of the person is 180cm2. Find the pressure exerted on the ground by the person. (Take g=9.8m/s2)
View SolutionA block of mass 2kg is released from rest on a rough inclined ground as shown in figure. Find the work done on the block by.
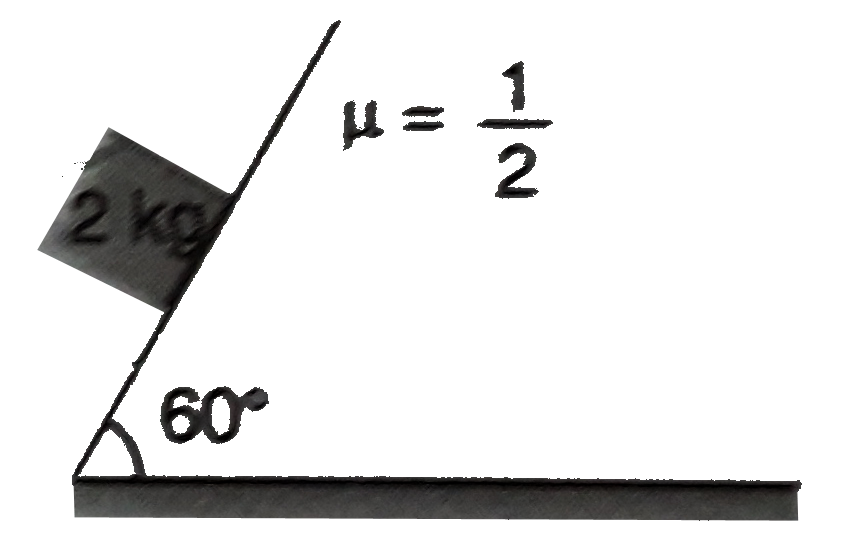 .
.
(Take g10m/s2).
View SolutionThe foot of an elephant has an area of 275cm2. If the mass of the elephant is 2200 kg, find the pressure exerted by the on the ground. (g=10ms−2)
View SolutionA uniform rod of length 1m having mass 1kg rests against a smooth wall at an angle of 30∘ with the ground. Calculate the force exerted by the ground on the rod. Take g=10ms−2.
View SolutionIf a block of 5 kg is lying on the ground, then the thrust on the surface of the ground due to the weight of the block.
View SolutionAn object of mass 8 kg is placed at a height of 5 m above the ground.
Find the amount of energy possessed by the object. (Take g=9.8 m/s2)
View Solution
