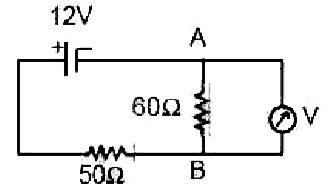Two resistances 300Ω and 400Ω are connected to a 60 V power supply as shown in Fig, A voltmeter connected across the 400Ω resistor reads 30 V.
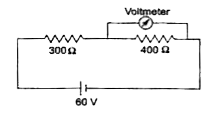
The resistance of the voltmeter is:
6000Ω
800Ω
1000Ω
1200Ω
The correct Answer is:D
Potential difference across the 400Ω resistance=30 V. Therefore, potential difference across the 300Ω resistance = 60-30V=30 V. Let R be the resistance of the voltmeter. As the voltmeter is in parallel with the 400Ω resistance, their combined resistance is.
R.=400R400+R
As the potential differenceof 60 V is equally, shared between the 300Ω and 400Ω resistances. R. should be equal to 300Ω. Thus
300400R400+R
which gives R=1200Ω
Similar Questions
Knowledge Check
- Question 1 - Select One
The voltmeter reads 40V across 60Ω resistance. The resistance of voltmeter is.
 .A10ΩB20ΩC30ΩD40Ω
.A10ΩB20ΩC30ΩD40Ω - Question 2 - Select One
Two resistances 300 ohm and 400 ohm are connected in series with a 60 volt supply. voltmeter connected across 400 ohm reads 30V. The same voltmeter when connected across the other resistance will read.
A22.5 VB50 VC30 VD10 V - Question 3 - Select One
Two resistors 4Ω and 800Ω are connected in series with a 6V battery. The potential difference measured by voltmeter of 10kΩ across 400Ω resistor is
A2VB1.95VC3.8VD4V
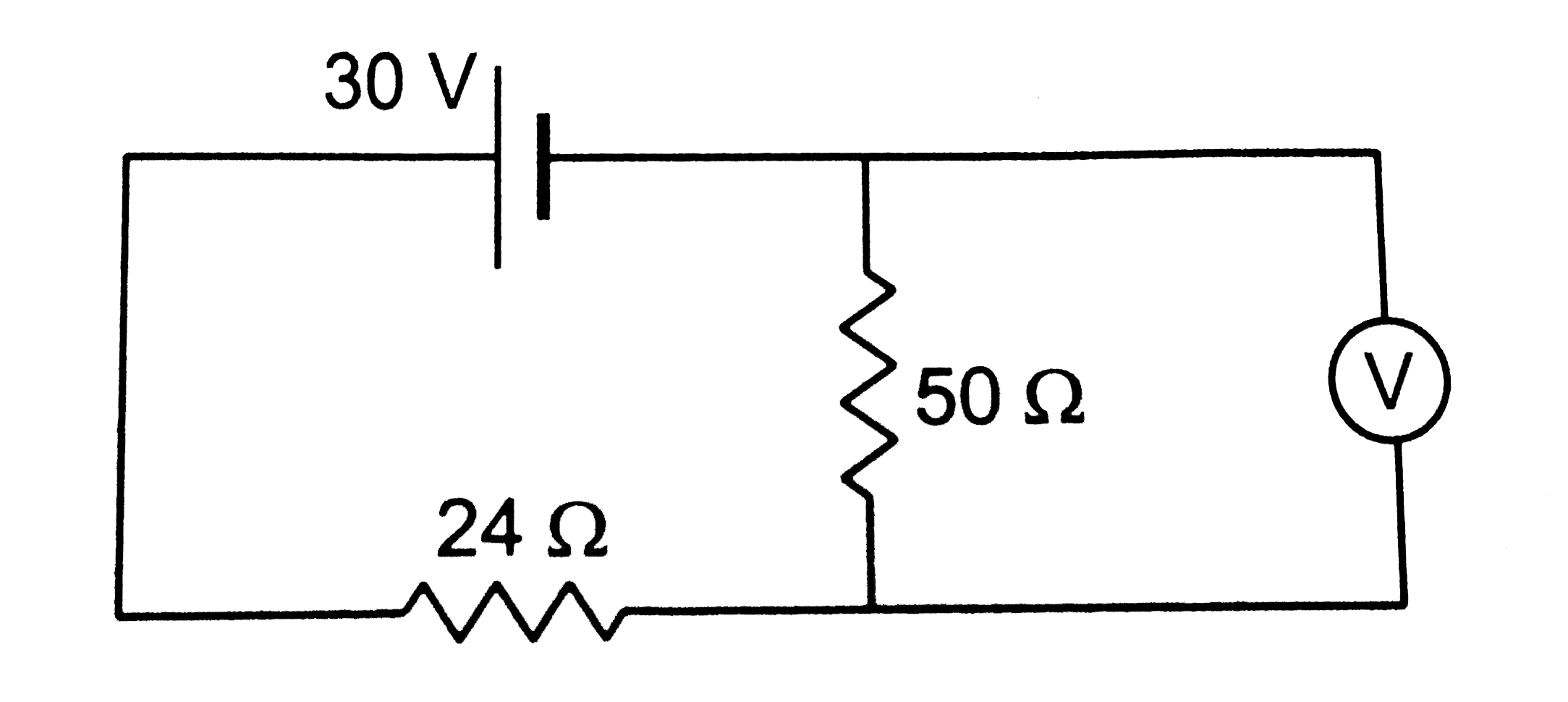
The voltmeter shown in figure reads 18V across the 50(Ω)resistor. Find the resistance of the voltmeter.
 View Solution
View SolutionA 600Ω resistor and a 400Ω resistor are connected in series across a 90-volt line. A voltmeter acros the 600Ω resistor reads 45V. What is the value of the voltmeter resistance? If the same voltmeter is connected across the 400Ω resistor, what will be its reading?
View SolutionTwo resistor 400Ω and 800Ω connected in series across a 6 V battery . Reading of Voltmeter of 10kΩ across 400Ω resistor is close to :
View SolutionTwo resistor 400Ω and 800Ω connected in series across a 6 V battery . Reading of Voltmeter of 10kΩ across 400Ω resistor is close to :
View SolutionThe voltmeter shown in the figure reads 10V across 40 ω . The resistance of voltmeter is
View Solution