On a triangle ABC, on the side AB, 5 points are marked, 6 points are marked on the side BC and 3 points are marked on the side AC (none of the points being the vertex of the triangle). How many triangles can be made by using these points?
90
333
328
None of these
The correct Answer is:B
To solve the problem of how many triangles can be formed using the marked points on the sides of triangle ABC, we can follow these steps:
1. Identify the Points:
- On side AB, there are 5 points.
- On side BC, there are 6 points.
- On side AC, there are 3 points.
2. Calculate Total Points:
- Total points = Points on AB + Points on BC + Points on AC
- Total points = 5 + 6 + 3 = 14 points.
3. Choose 3 Points to Form a Triangle:
- To form a triangle, we need to select 3 points from the total of 14 points.
- The number of ways to choose 3 points from 14 is given by the combination formula (nr), where n is the total number of points and r is the number of points to choose.
- Thus, we need to calculate (143).
4. Calculate (143):
- Using the formula (nr)=n!r!(n−r)!:
(143)=14!3!(14−3)!=14!3!⋅11!
- This simplifies to:
(143)=14×13×123×2×1=21846=364
5. Subtract Collinear Points:
- We need to subtract the cases where the selected points are collinear (which do not form a triangle).
- Collinear points can be chosen from each side:
- For side AB (5 points): (53)=10
- For side BC (6 points): (63)=20
- For side AC (3 points): (33)=1
- Total collinear combinations = 10+20+1=31.
6. Calculate Valid Triangles:
- The total number of valid triangles is:
Total triangles=Total combinations−Collinear combinations=364−31=333.
Final Answer:
The total number of triangles that can be formed is 333.
- On side AB, there are 5 points.
- On side BC, there are 6 points.
- On side AC, there are 3 points.
- Total points = Points on AB + Points on BC + Points on AC
- Total points = 5 + 6 + 3 = 14 points.
- To form a triangle, we need to select 3 points from the total of 14 points.
- The number of ways to choose 3 points from 14 is given by the combination formula (nr), where n is the total number of points and r is the number of points to choose.
- Thus, we need to calculate (143).
- Using the formula (nr)=n!r!(n−r)!:
(143)=14!3!(14−3)!=14!3!⋅11!
- This simplifies to:
(143)=14×13×123×2×1=21846=364
- We need to subtract the cases where the selected points are collinear (which do not form a triangle).
- Collinear points can be chosen from each side:
- For side AB (5 points): (53)=10
- For side BC (6 points): (63)=20
- For side AC (3 points): (33)=1
- Total collinear combinations = 10+20+1=31.
- The total number of valid triangles is:
Total triangles=Total combinations−Collinear combinations=364−31=333.
The total number of triangles that can be formed is 333.
Topper's Solved these Questions
- PERMUTATIONS AND COMBINATIONSBOOK - DISHA PUBLICATIONCHAPTER - PERMUTATIONS AND COMBINATIONSEXERCISE - PRACTICE EXERCISES ( EXPERT LEVEL )
48 Videos
- PERCENTAGESBOOK - DISHA PUBLICATIONCHAPTER - PERCENTAGESEXERCISE - PRACTICE EXERCISE (TEST YOURSELF)
15 Videos
- PROBABILITYBOOK - DISHA PUBLICATIONCHAPTER - PROBABILITYEXERCISE - TEST YOURSELF
15 Videos
Similar Questions
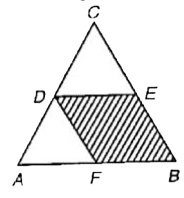
ABC is a triangle in which D, E and F are the mid-points of the sides AC, BC and AB respectively. What is the ratio of the area of the shaded to the unshaded region in the triangle?
View SolutionIn a triangle ABC, points P, Q and R are the mid-points of the sides AB, BC and CA respectively. If the area of the triangle ABC is 20 sq. units, find the area of the triangle PQR
View Solution
Knowledge Check
Twenty points are marked on a plane so that non three points are collinear except 7 points. How many triangle can be formed by joining the points?
A995B1105C1200D1250Twelve points are marked on a plane so that no three points are collinear. How many different triangles can be formed joining the points.
A180B190C220D230There are 10 points on a straight line AB and 8 on another straight line AC, none of them being A. How many triangles can be formed with these points as vertices?
A720B640C816DNone of these
The sides of a triangle have 4, 5 and 6 interior points marked on them respectively. The total number of triangles that can be formed using any of these points
View SolutionIn a triangle ABC with side AB = AC and ∠BAC=20∘, D is a point on side AC and BC = AD. Find ∠DBC
View SolutionIn right angled ΔABC,∠B=90∘, if P and Q are points on the sides AB and BC respectively, then :

View SolutionA triangle ABC in which side AB,BC,CA consist 5,3,6 points respectively, then the number of triangle that can be formed by these points are
View SolutionIn a triangle ABC with side AB = AC and ∠BAC=20∘, D is a point on side AC and BC = AD. Find ∠DBC
View Solution
