In a chess competition involving some boys and girls of a school, every student had to play exactly one game with every other student. It was found that in 45 games both the players were girls and in 190 games both were boys. The number of games in which one player was a boy and the other was a girl is
200
216
235
256
The correct Answer is:A
To solve the problem, we need to determine the number of games played where one player is a boy and the other is a girl. We are given the number of games played by girls and boys separately.
1. Define Variables:
Let n be the number of boys and m be the number of girls.
2. Games Played by Girls:
The number of games played between girls is given by the combination formula (m2):
(m2)=45
This can be expressed as:
m(m−1)2=45
Multiplying both sides by 2:
m(m−1)=90
3. Solve for m:
We need to find two consecutive integers whose product is 90. The pairs are:
- 9×10=90
Thus, m=10 (the number of girls).
4. Games Played by Boys:
The number of games played between boys is given by:
(n2)=190
This can be expressed as:
n(n−1)2=190
Multiplying both sides by 2:
n(n−1)=380
5. Solve for n:
We need to find two consecutive integers whose product is 380. The pairs are:
- 19×20=380
Thus, n=20 (the number of boys).
6. Calculate Games with One Boy and One Girl:
The number of games where one player is a boy and the other is a girl can be calculated as:
n×m=20×10=200
Final Answer:
The number of games in which one player was a boy and the other was a girl is 200.
---
Let n be the number of boys and m be the number of girls.
The number of games played between girls is given by the combination formula (m2):
(m2)=45
This can be expressed as:
m(m−1)2=45
Multiplying both sides by 2:
m(m−1)=90
We need to find two consecutive integers whose product is 90. The pairs are:
- 9×10=90
Thus, m=10 (the number of girls).
The number of games played between boys is given by:
(n2)=190
This can be expressed as:
n(n−1)2=190
Multiplying both sides by 2:
n(n−1)=380
We need to find two consecutive integers whose product is 380. The pairs are:
- 19×20=380
Thus, n=20 (the number of boys).
The number of games where one player is a boy and the other is a girl can be calculated as:
n×m=20×10=200
The number of games in which one player was a boy and the other was a girl is 200.
Topper's Solved these Questions
- PERMUTATIONS AND COMBINATIONSBOOK - DISHA PUBLICATIONCHAPTER - PERMUTATIONS AND COMBINATIONSEXERCISE - PRACTICE EXERCISES ( EXPERT LEVEL )
48 Videos
- PERCENTAGESBOOK - DISHA PUBLICATIONCHAPTER - PERCENTAGESEXERCISE - PRACTICE EXERCISE (TEST YOURSELF)
15 Videos
- PROBABILITYBOOK - DISHA PUBLICATIONCHAPTER - PROBABILITYEXERCISE - TEST YOURSELF
15 Videos
Similar Questions
Knowledge Check
In a carrom board game competition, m boys and n girls (m>n>1) of a school participate in which every student has to play exactly one game with every other student. Out of the total games played, it was found that in 221 games one player was a boy and the other player was a girl. Consider the following statements :
1. The total number of student that participated in the competition is 30.
2. The number of games in which both players were 4 girls is 78.
Which of the statements given above is/are correct?A1 onlyB2 onlyCBoth 1 and 2DNeither 1 nor 2In a badminton each player played one game with ll the other players. Number of players participated in the tournament if they played 105 games is
A35B15C12D10In a school, there are 360 students out of which two-thirds are girls and the rest are boys. Three-fourths of the number of boys are players. The number of boys who are not players are
A60B75C25D30
In a class tournament where the participants were to play one game with one another, two of the class players fell ill, having played 3 games each. If the total number of games played is 24, then the number of participants at the beginning was
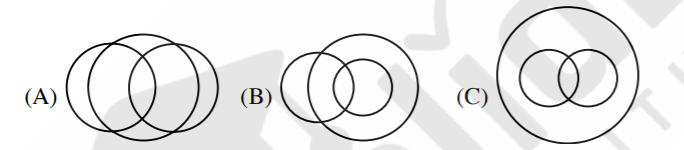
View Solution3 games are played in a school . If some students played exactly 2 games , and no student play all the 3 games , then which venn diagram can represents the above situtation
View SolutionIn a class of 60 students, the number of girls is one third the number of boys. Find the number of girls and boys in the class.
View Solution45% of the students in a school are boys. If the total number of students in the school is 880, find the number of girls in the school.
View SolutionIn a school out of 1000 students 548 play football, 367 play hockey and 198 play both games. Then, the number of students who play neither of the two games is
View Solution
