In a triangle ABC, if taken in order, consider the following statements
1. −−→AB+−−→BC+−−→CA=→0
2 −−→AB+−−→BC−−−→CA=→0
3. −−→AB−−−→BC+−−→CA=→0
4. −−→BA−−−→BC+−−→CA=→0
How many of the above statements are correct?
one
two
Three
Four
The correct Answer is:A
To solve the problem, we will analyze each of the given statements regarding the vectors in triangle ABC.
Step 1: Understand the Vectors in Triangle ABC
In triangle ABC, we can define the vectors as follows:
- →AB=→B−→A
- →BC=→C−→B
- →CA=→A−→C
Step 2: Analyze Each Statement
Statement 1: →AB+→BC+→CA=→0
Substituting the vectors:
→AB+→BC+→CA=(→B−→A)+(→C−→B)+(→A−→C)
Simplifying:
=→B−→A+→C−→B+→A−→C=→0
This statement is correct.
Statement 2: →AB+→BC−→CA=→0
Substituting the vectors:
→AB+→BC−→CA=(→B−→A)+(→C−→B)−(→A−→C)
Simplifying:
=→B−→A+→C−→B−→A+→C=2→C−2→A≠→0
This statement is incorrect.
Statement 3: →AB−→BC+→CA=→0
Substituting the vectors:
→AB−→BC+→CA=(→B−→A)−(→C−→B)+(→A−→C)
Simplifying:
=→B−→A−→C+→B+→A−→C=2→B−2→C≠→0
This statement is incorrect.
Statement 4: →BA−→BC+→CA=→0
Substituting the vectors:
→BA−→BC+→CA=(→A−→B)−(→C−→B)+(→A−→C)
Simplifying:
=→A−→B−→C+→B+→A−→C=2→A−2→C≠→0
This statement is incorrect.
Conclusion
Only Statement 1 is correct. Therefore, the number of correct statements is 1.
In triangle ABC, we can define the vectors as follows:
- →AB=→B−→A
- →BC=→C−→B
- →CA=→A−→C
→AB+→BC+→CA=(→B−→A)+(→C−→B)+(→A−→C)
Simplifying:
=→B−→A+→C−→B+→A−→C=→0
This statement is correct.
→AB+→BC−→CA=(→B−→A)+(→C−→B)−(→A−→C)
Simplifying:
=→B−→A+→C−→B−→A+→C=2→C−2→A≠→0
This statement is incorrect.
→AB−→BC+→CA=(→B−→A)−(→C−→B)+(→A−→C)
Simplifying:
=→B−→A−→C+→B+→A−→C=2→B−2→C≠→0
This statement is incorrect.
→BA−→BC+→CA=(→A−→B)−(→C−→B)+(→A−→C)
Simplifying:
=→A−→B−→C+→B+→A−→C=2→A−2→C≠→0
This statement is incorrect.
Only Statement 1 is correct. Therefore, the number of correct statements is 1.
Topper's Solved these Questions
Similar Questions
Knowledge Check
If →A+→B=→C, and ∣∣→A∣∣=2∣∣→B∣∣ and →B.→C=0, then
A∣∣→A+→C∣∣=∣∣→A+→B∣∣B∣∣→A+→B∣∣=BC→A.→B<0D→A.→C may be zeroConsider the following statements:
1. The magnitude of →a×→b is same as the area of a triangle with sides →aand→b.
2. If →a×→b=→0 where →a≠0.→b≠0 then →a=λ→b which of the following statements is/are correct?AA) 1 onlyBB) 2 onlyCC) Both 1 and 2DD) Neither 1 nor 2→a+→b+→c=→0 such that |→a|=3,∣∣→b∣∣=5and|→c|=7.
What is →a.→b+→b.→c+→c.→a equal to ?A-83B−83/2C75D−75/2
State whether the following relations are true or false
(1)−−→AB=−−→BA
(2)−−→AB=−−−→BA
(3)∣∣∣−−→AB∣∣∣=∣∣∣−−→BA∣∣∣
(4)∣∣∣−−→AB∣∣∣=∣∣∣−−−→AB∣∣∣
(5)ˆj=ˆk
(6)∣∣ˆj∣∣=∣∣ˆk∣∣
View SolutionABC is an equilateral triangle. Length of each side is 'a' and centroid is point O.Find
(i) −−→AB+−−→BC+−−→CA=?
(ii) −−→OA+−−→OB+−−→OC=?
(iii) If ∣∣∣−−→AB+−−→BC+−−→AC∣∣∣= na then n = ?
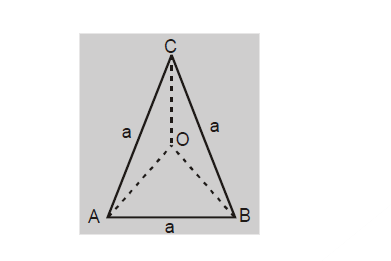
(iv) If −−→AB+−−→AC=n−−→AO then n = ?
View SolutionConsider the following inequalities in respect of vector →aand→b
1. ∣∣→a+→b∣∣≤|→a|+∣∣→b∣∣
2. ∣∣→a−→b∣∣≥|→a|−∣∣→b∣∣
Which of the above is/are correct?
View SolutionIf ABCDE is a pentagon, then
−−→AB+−−→AE+−−→BC+−−→DC+−−→ED+−−→AC is equal to
View SolutionAssertion A : If A, B, C, D are four points on a semi-circular arc with centre at 'O' such that
∣∣∣−−→AB∣∣∣=∣∣∣−−→BC∣∣∣=∣∣∣−−→CD∣∣∣, then
−−→AB+−−→AC+−−→AD=4−−→AO+−−→OB+−−→OC
Reason R : Polygon law of vector addition yields
−−→AB+−−→BC+−−→CD+−−→AD=2−−→AO
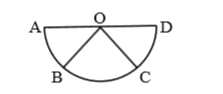
In the light of the above statements, choose the most appropriate answer from the options given below :
View Solution
