Using Gauss's theorem derive an expression for electric field intensity at a point due to -uniformly charged spherical shell.
Consider a thin spherical shell of radius R and centre O. Let a charge + q be distributed uniformly over the surface of the shell. Let us calculate electric field intensity at point P, where OP=r. Imagine a sphere S, of radius r with centre O. The surface of this sphere is Gaussian surface. According to Gauss.s theorem, ∮→E.−→dS ⇒ ∮EdScosθ=qɛ0
or E∮dS=qɛ0 [∴→E||−→dS]
∴ E4πr2=qɛ0
∴ E4πr2=qɛ0
or E=q4πɛ0r2 ........(i)

Electric field intensity at any point outside the spherical shell is such as, if the entire charge were concentrated at the centre of the shell.
At a point on the surface of the shell , r=R∴E=q4πɛ0R2
If σ is surface density of charge on the shell, then q=4πR2σ
∴ E=4πR2σ4πɛ0R2=σɛ0 If the point P lies inside the spherical shell then, Gaussian surface is the surface of a sphere S2, of radius r(<R). As the charge inside the spherical strell is zero, therefore the Gaussian surface in this case encloses no charge,
i.e., q=0∴E=0 i.e., electric field inside a spherical shell is always zero. Figure shows the variation of electric field intensity E with distance from the centre of a uniformly charged spherical shell. 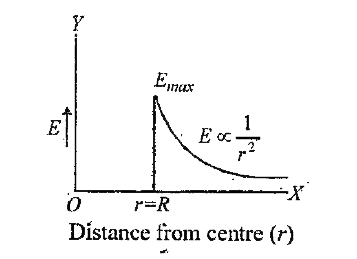
Similar Questions
Derive an expression for electric field intensity at a point due to point charge.
View SolutionDerive an expression for electric field intensity at a point due to point charge.
View SolutionState Gauss's theorem in electrostatics. Apply this theorem to derive an expression for electric field intensity at a point outside a uniformly charged thin spherical shell.
View SolutionUsing Gauss's law, derive an expression for the electric field intensity at any point near a uniformly charged thin wire of charge/length=λC/m.
View SolutionApply Gauss's theorem to find an expression for the electric field intensity at a point due to a point charge.
View SolutionUsing Gauss's theorem derive an expression for the electric field at any point outside a charged spherical shell of radius R and of charge density σC/m2
View SolutionUsing Gauss’s theorem in electrostatics, deduce an expression for electric field intensity due to a charged spherical shell at a point (i) inside (ii) on its surface (iii) outside it. Graphically show the variation of electric field intensity with distance from the centre of shell.
View SolutionDerive an expression for electric intensity E due to a uniformly charged thin spherical shell at a point outside the shell.
View SolutionFind expression for electric field intensity due to a uniformly charged thin spherical shell at a point outside the shell.
View SolutionUsing Gauss’ law, derive an expression for the electric field at a point near an infinitely long straight uniformly charged wire.
View Solution
