From the peak of a hill which is 300 m high ,the angle of depression of two sides of a bridge lying on a ground are 45∘and30∘(both ends of the bridge are on the same side of the hill) .Then the length of the bridge is
300(√3−1)m
300(√3+1)m
300√3m
300√3m
The correct Answer is:A
To solve the problem, we will use trigonometric principles involving angles of depression and the properties of right triangles.
1. Understand the Problem:
We have a hill that is 300 m high. From the top of the hill, we observe two points on a bridge below, with angles of depression of 45° and 30°.
2. Draw a Diagram:
Visualize the situation. Let:
- Point A be the top of the hill (300 m high).
- Point B be the point on the ground directly below A.
- Point C be one end of the bridge where the angle of depression is 45°.
- Point D be the other end of the bridge where the angle of depression is 30°.
3. Identify the Right Triangles:
- Triangle ABC (for angle of depression 45°)
- Triangle ABD (for angle of depression 30°)
4. Using the Angle of Depression:
- The angle of depression from A to C is 45°. Therefore, the angle of elevation from C to A is also 45°.
- The angle of depression from A to D is 30°. Therefore, the angle of elevation from D to A is also 30°.
5. Calculate Distances:
- For triangle ABC (with angle 45°):
tan(45°)=ABBC
Since tan(45°)=1:
1=300BC⟹BC=300 m
- For triangle ABD (with angle 30°):
tan(30°)=ABBD
Since tan(30°)=1√3:
1√3=300BD⟹BD=300√3 m
6. Find the Length of the Bridge:
The length of the bridge CD is the difference between BD and BC:
CD=BD−BC=300√3−300
Factoring out 300:
CD=300(√3−1) m
Final Answer:
The length of the bridge is 300(√3−1) meters.
---
We have a hill that is 300 m high. From the top of the hill, we observe two points on a bridge below, with angles of depression of 45° and 30°.
Visualize the situation. Let:
- Point A be the top of the hill (300 m high).
- Point B be the point on the ground directly below A.
- Point C be one end of the bridge where the angle of depression is 45°.
- Point D be the other end of the bridge where the angle of depression is 30°.
- Triangle ABC (for angle of depression 45°)
- Triangle ABD (for angle of depression 30°)
- The angle of depression from A to C is 45°. Therefore, the angle of elevation from C to A is also 45°.
- The angle of depression from A to D is 30°. Therefore, the angle of elevation from D to A is also 30°.
- For triangle ABC (with angle 45°):
tan(45°)=ABBC
Since tan(45°)=1:
1=300BC⟹BC=300 m
tan(30°)=ABBD
Since tan(30°)=1√3:
1√3=300BD⟹BD=300√3 m
The length of the bridge CD is the difference between BD and BC:
CD=BD−BC=300√3−300
Factoring out 300:
CD=300(√3−1) m
The length of the bridge is 300(√3−1) meters.
Topper's Solved these Questions
- BOOK - KIRAN PUBLICATIONCHAPTER - TRIGONOMETRY EXERCISE - TYPE -V45videos
TRIGONOMETRY
- BOOK - KIRAN PUBLICATIONCHAPTER - TRIGONOMETRY EXERCISE - TEST YOURSELF25videos
TRIGONOMETRY
- BOOK - KIRAN PUBLICATIONCHAPTER - TRIGONOMETRY EXERCISE - TYPE -III90videos
TRIGONOMETRY
- BOOK - KIRAN PUBLICATIONCHAPTER - TIME AND WORKEXERCISE - TEST YOURSELF25videos
TIME AND WORK
Similar Questions
A vertiacal pole is 60 m high, The angle of depression of two points P and Q on the ground are 30∘and45∘ respectively. If the points P and Q lie on either side of the pole, then find the distance PQ.
View SolutionThe angles of depression of two ships from the top of a lighthouse are 45∘and30∘. If the ships are 120 m apart, then find the height of the lighthouse.
View Solution
Knowledge Check
- Question 1 - Select One
From the top of a cliff 90 m high, the angles of depression of the top and bottom of a tower are observed to be 30∘ and 60∘ respectively. The height of the tower is :
A60 mB75 mC30 mD45 m - Question 2 - Select One
From the top of a tower of height 180 m the angles of depression of two objects on either sides of the tower are 30∘and45∘ . Then the distance between the objects are
A180(3+√3)mB180(3−√3)mC180(√3−1)mD180(√3+1)m - Question 3 - Select One
From the top of a cliff 50 m high, the angles of depression of the top and bottom of a tower are observed to be 30∘ and 45∘. The height of tower is
A50mB50√3mC50(√3−1)mD50(1−√33)m
From the top of a hill, the angles of depression of two consecutive kilometre stones due east are found to be 300and450 . Find the height of the hill.
View SolutionFrom the top of the hill; the angle of depressions of two consecutive kilometre stones due east are found to be 30∘ and 45∘ . Find the height of the hill.
View SolutionThe height of a hill is 150 m. From the top of the hill the angles of depression of two objects lying towards east to the hill are 45∘ and 30∘. Find the distance btween the objects.
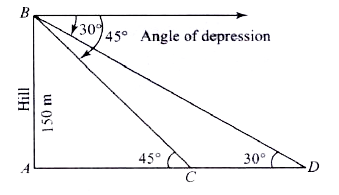 View Solution
View SolutionFrom a tower 125 metres high the angle of depression of two objects ,which are in horizontal line through the base of the tower , are 45∘and30∘ and they are on the same side of the tower The distance (in metres) between the objects is
View SolutionThe cliff a mountain is 180 m high and the angles of depression of two ships on the either side of cliff are 30∘and60∘ .What is the distance between the tow ships ?
View Solution

