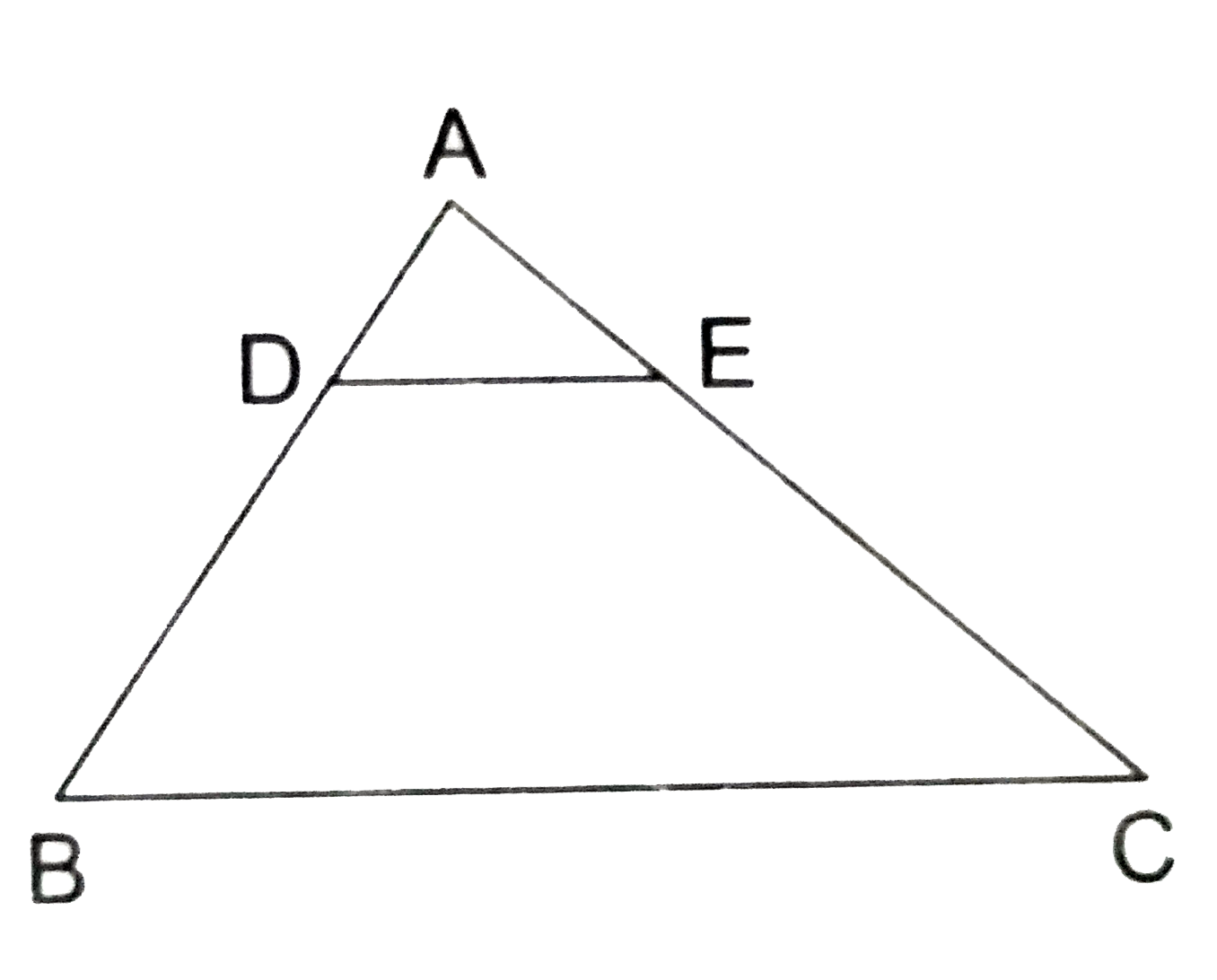
D and E are points on the sides AB and AC respectively of $Δ A B C$ such that DE is parallel to BC and AD:DB = 4:5, CD and BE intersect each other at F. Then the ratio of the areas of $Δ D E F$ and $Δ C B F$ .

$16 : 25$

$16 : 81$

$81 : 16$

$4 : 9$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:B

Updated on:21/07/2023

Answer

Step by step video, text & image solution for D and E are points on the sides AB and AC respectively of DeltaABC such that DE is parallel to BC and AD:DB = 4:5, CD and BE intersect each other at F. Then the ratio of the areas of DeltaDEF and DeltaCBF. by Maths experts to help you in doubts & scoring excellent marks in Class 14 exams.

Updated on:21/07/2023

Class 14 MATHS MENSURATION

Topper's Solved these Questions

Knowledge Check

D and E are two points on the sides AC and BC respectively of $Δ A B C$ such that $D E = 18 c m, C E = 5 c m$ and $∠ D E C = 90^{\circ}$ . If tan $∠ A B C = 3.6$ , then $A C : C D =$
A $B C : 2 C E$
B $2 C E : B C$
C $2 B C : C E$
D $C E : 2 B C$
D and E are points on sides AB and AC of $Δ A B C$ . DE is parallel to BC. If AD : DB = 2 : 3, what is the ratio of area $Δ A D E$ and area of quadrilateral BDEC ?
A $4 : 21$
B $4 : 25$
C $4 : 29$
D $4 : 9$
In $Δ A B C$ , D and E are points on the sides AB and AC respectively, such that DE || BC and $D E : B C = 6 : 7$ . (Area of $Δ A D E$ ) : (Area of trapezium BCED) = ?
A $49 : 13$
B $36 : 13$
C $13 : 49$
D $13 : 36$

KIRAN PUBLICATION-MENSURATION-TYPE -II

Home
Ask Doubt
Profile

D and E are points on the sides AB and AC respectively of ΔABC such that DE is parallel to BC and AD:DB = 4:5, CD and BE intersect each other at F. Then the ratio of the areas of ΔDEF and ΔCBF.

The correct Answer is:B

Topper's Solved these Questions

Similar Questions

Knowledge Check

D and E are two points on the sides AC and BC respectively of ΔABC such that DE=18cm,CE=5cm and ∠DEC=90∘. If tan ∠ABC=3.6, then AC:CD=

D and E are points on sides AB and AC of ΔABC. DE is parallel to BC. If AD : DB = 2 : 3, what is the ratio of area ΔADE and area of quadrilateral BDEC ?

In ΔABC, D and E are points on the sides AB and AC respectively, such that DE || BC and DE:BC=6:7. (Area of ΔADE) : (Area of trapezium BCED) = ?

KIRAN PUBLICATION-MENSURATION-TYPE -II

D and E are points on the sides AB and AC respectively of $Δ A B C$ such that DE is parallel to BC and AD:DB = 4:5, CD and BE intersect each other at F. Then the ratio of the areas of $Δ D E F$ and $Δ C B F$ .

D and E are two points on the sides AC and BC respectively of $Δ A B C$ such that $D E = 18 c m, C E = 5 c m$ and $∠ D E C = 90^{\circ}$ . If tan $∠ A B C = 3.6$ , then $A C : C D =$

D and E are points on sides AB and AC of $Δ A B C$ . DE is parallel to BC. If AD : DB = 2 : 3, what is the ratio of area $Δ A D E$ and area of quadrilateral BDEC ?

In $Δ A B C$ , D and E are points on the sides AB and AC respectively, such that DE || BC and $D E : B C = 6 : 7$ . (Area of $Δ A D E$ ) : (Area of trapezium BCED) = ?