If D and E are the mid points of AB and AC respectively of ΔABC, then the ratio of the areas of ADE and BCED is?
1:2
1:4
2:3
1:3
To solve the problem, we need to find the ratio of the areas of triangles ADE and BCED in triangle ABC, where D and E are the midpoints of sides AB and AC, respectively.
1. Identify the Points:
- Let triangle ABC be given with points A, B, and C.
- D is the midpoint of AB, and E is the midpoint of AC.
2. Draw the Triangle:
- Sketch triangle ABC with points A, B, and C. Mark points D and E on sides AB and AC, respectively.
3. Use the Midpoint Theorem:
- By the midpoint theorem, line segment DE (connecting midpoints D and E) is parallel to side BC and DE = 1/2 BC.
4. Establish Similarity:
- Since DE is parallel to BC, triangles ADE and ABC are similar by AA (Angle-Angle) similarity criterion. Angle A is common, and angles D and B are equal (corresponding angles).
5. Determine the Ratio of Corresponding Sides:
- The ratio of the sides of triangle ADE to triangle ABC is 1:2 (since D and E are midpoints).
6. Calculate the Ratio of Areas:
- The ratio of the areas of similar triangles is equal to the square of the ratio of their corresponding sides.
- Therefore, the area ratio of triangle ADE to triangle ABC is (1/2)² = 1/4.
7. Find the Area of Triangle BCED:
- The area of triangle ABC is the sum of the areas of triangles ADE and BCED.
- If we let the area of triangle ABC be 4 units (since the area of triangle ADE is 1 unit), then the area of triangle BCED is:
Area of BCED=Area of ABC−Area of ADE=4−1=3 units.
8. Calculate the Final Ratio:
- The ratio of the areas of triangles ADE and BCED is:
Area of ADE:Area of BCED=1:3.
Conclusion:
The ratio of the areas of triangles ADE and BCED is 1:3.
- Let triangle ABC be given with points A, B, and C.
- D is the midpoint of AB, and E is the midpoint of AC.
- Sketch triangle ABC with points A, B, and C. Mark points D and E on sides AB and AC, respectively.
- By the midpoint theorem, line segment DE (connecting midpoints D and E) is parallel to side BC and DE = 1/2 BC.
- Since DE is parallel to BC, triangles ADE and ABC are similar by AA (Angle-Angle) similarity criterion. Angle A is common, and angles D and B are equal (corresponding angles).
- The ratio of the sides of triangle ADE to triangle ABC is 1:2 (since D and E are midpoints).
- The ratio of the areas of similar triangles is equal to the square of the ratio of their corresponding sides.
- Therefore, the area ratio of triangle ADE to triangle ABC is (1/2)² = 1/4.
- The area of triangle ABC is the sum of the areas of triangles ADE and BCED.
- If we let the area of triangle ABC be 4 units (since the area of triangle ADE is 1 unit), then the area of triangle BCED is:
Area of BCED=Area of ABC−Area of ADE=4−1=3 units.
- The ratio of the areas of triangles ADE and BCED is:
Area of ADE:Area of BCED=1:3.
The ratio of the areas of triangles ADE and BCED is 1:3.
Topper's Solved these Questions
Similar Questions
Knowledge Check
If D, E and F are the mid-points of sides BC, CA and AB respectively of △ABC, then the ratio of the areas of △DEF to the area of △ABC is :
A1:4B1:2C1:3D2:3If D, E and Fare the mid points of BC, CA and AB respectively of the ABC then the ratio of area of the parallelogram DEFB and area of the trapezlum CAFD is:
A2:3B3:4C1:2D1:3If D, E and F are the mid points of BC, CA and AB respectively of the △ABC then the ratio of aria parallelogram DEFB and area of the trapezium CAFD is ,
A2:3B3:4C1:2D1:3
If D and E are the mid-points of the side AB and AC respectively of the ΔABC in the figure given here, the shaded region of the triangle is what per cent of the whole triangular region?
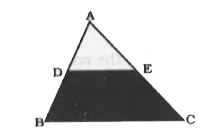
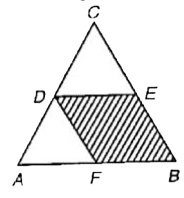
View SolutionABC is a triangle in which D, E and F are the mid-points of the sides AC, BC and AB respectively. What is the ratio of the area of the shaded to the unshaded region in the triangle?
View SolutionSuppose ABC is a triangle with AB of unit length. D and E are the points lying on AB and AC respectively such that BC and DE are parallel. If the area of triangle ABC is twice the area of triangle ADE, then the length of AD is
View SolutionD is any point on side AC of ΔABC. If P,Q, X, Y are the mid points of AB,BC,ADandDCrespectively, then the ratio of PXandQYis
View SolutionABC is a triangle and D,E,F are the mid-points of the sides BC,CA, AB respectively . The ratio of the areas of ΔABCandΔDEF is :
View Solution

