ABC is a right angled triangle. B being the right angle. Mid-points of BC and AC are respectively B' and A'. Area of ΔA'B'C' is
12× area of ΔABC
23× area of ΔABC
14× area of ΔABC
18× area of ΔABC
The correct Answer is:C
Step by step video, text & image solution for ABC is a right angled triangle. B being the right angle. Mid-points of BC and AC are respectively B' and A'. Area of DeltaA'B'C' is by Maths experts to help you in doubts & scoring excellent marks in Class 14 exams.
Updated on:21/07/2023Topper's Solved these Questions
- MENSURATIONBOOK - KIRAN PUBLICATIONCHAPTER - MENSURATIONEXERCISE - TYPE - III
21 Videos
- MENSURATIONBOOK - KIRAN PUBLICATIONCHAPTER - MENSURATIONEXERCISE - TYPE - IV
169 Videos
- MENSURATIONBOOK - KIRAN PUBLICATIONCHAPTER - MENSURATIONEXERCISE - Test Yourself
28 Videos
- LCM AND HCF BOOK - KIRAN PUBLICATIONCHAPTER - LCM AND HCF EXERCISE - Test Yourself
18 Videos
- MISCELLANEOUSBOOK - KIRAN PUBLICATIONCHAPTER - MISCELLANEOUSEXERCISE - TYPE-VI
15 Videos
Similar Questions
Knowledge Check
ABC is a right angled triangle. B being the right angle. Mid-points of BC and AC are respectively B' and A'. Area of ΔA'B'C' is
A12×ΔABC का क्षेत्रफलB23×ΔABC का क्षेत्रफलC14×ΔABC का क्षेत्रफलD18×ΔABC का क्षेत्रफलABC is a right angled triangle, B being the right angle. Mid-points of BC and AC are respectively B' and A'. The ratio of the area of the quadrilateral AA' B'B to the area of the triangles ABC is
A1:2B2:3C3:4DNone of the aboveIn right angled ΔABC,∠B=90∘, if P and Q are points on the sides AB and BC respectively, then :
 AAQ2+CP2=2(AC2+PQ2)BAQ2+CP2=AC2+PQ2C(AQ2+CP2)=12(AC2+PQ2)D(AQ+CP)=12(AC+PQ)
AAQ2+CP2=2(AC2+PQ2)BAQ2+CP2=AC2+PQ2C(AQ2+CP2)=12(AC2+PQ2)D(AQ+CP)=12(AC+PQ)
The distance of incentre of the right-angled triangle ABC (right angled at A) from B and C are √10and√5, respectively. The perimeter of the triangle is _____
View SolutionIn the given figure, ABC is a right angled triangle with B as right angle. Three semicircles are drawn with AB, BC and AC as diameters. What is the area of the shaded portion, if the area of ΔABC is 12 square units ?
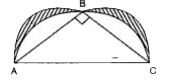
View SolutionIf ABC is a right angled triangle at B and M, N are the mid-points of AB and BC, then 4(AN2+CM2) is equal to :
View SolutionLet triangle ABC is right triangle right angled at C such that A<B and r=8,R=41 .
Q. Area of ΔABC is :
View SolutionLet triangle ABC is right triangle right angled at C such that A<B and r=8,R=41 .
Q. Area of ΔABC is :
View Solution
