The angle of depression of vertices of a regular hexagon lying in a plane from the top of a 75 m high tower standing at the centre of the hexagon is 60∘. What is the length of each side of the hexagon ?
50√3 m
75 m
25√3 m
25 m
The correct Answer is:C
To solve the problem step by step, we will use the information given about the regular hexagon and the height of the tower.
1. Understand the Setup:
- We have a regular hexagon with vertices A, B, C, D, E, and F.
- A tower of height 75 m is located at the center of the hexagon (point O).
- The angle of depression from the top of the tower to each vertex of the hexagon is 60°.
2. Identify the Triangle:
- When looking from the top of the tower (point A) to a vertex of the hexagon (point B), we can form a right triangle OAB.
- In this triangle, AO is the height of the tower (75 m), and OB is the horizontal distance from the center of the hexagon to vertex B.
3. Use the Angle of Depression:
- The angle of depression from A to B is 60°. Therefore, the angle ∠OAB is also 60° (alternate interior angles).
- We can use the tangent function to relate the height and the distance:
tan(60°)=AOOB
- Substituting the known values:
tan(60°)=√3andAO=75 m
√3=75OB
4. Solve for OB:
- Rearranging the equation gives us:
OB=75√3=25√3 m
5. Determine the Length of the Side of the Hexagon:
- In a regular hexagon, all sides are equal, and the distance from the center to any vertex (OB) is equal to the radius of the circumcircle of the hexagon.
- The length of each side (s) of the hexagon can be calculated using the formula:
s=OB⋅√32
- Substituting the value of OB:
s=25√3⋅√32=25⋅32=752=37.5 m
6. Final Answer:
- The length of each side of the hexagon is 25√3 m.
- We have a regular hexagon with vertices A, B, C, D, E, and F.
- A tower of height 75 m is located at the center of the hexagon (point O).
- The angle of depression from the top of the tower to each vertex of the hexagon is 60°.
- When looking from the top of the tower (point A) to a vertex of the hexagon (point B), we can form a right triangle OAB.
- In this triangle, AO is the height of the tower (75 m), and OB is the horizontal distance from the center of the hexagon to vertex B.
- The angle of depression from A to B is 60°. Therefore, the angle ∠OAB is also 60° (alternate interior angles).
- We can use the tangent function to relate the height and the distance:
tan(60°)=AOOB
- Substituting the known values:
tan(60°)=√3andAO=75 m
√3=75OB
- Rearranging the equation gives us:
OB=75√3=25√3 m
- In a regular hexagon, all sides are equal, and the distance from the center to any vertex (OB) is equal to the radius of the circumcircle of the hexagon.
- The length of each side (s) of the hexagon can be calculated using the formula:
s=OB⋅√32
- Substituting the value of OB:
s=25√3⋅√32=25⋅32=752=37.5 m
- The length of each side of the hexagon is 25√3 m.
Topper's Solved these Questions
- BOOK - LUCENT PUBLICATIONCHAPTER - HEIGHT AND DISTANCEEXERCISE - EXERCISE-12B14videos
HEIGHT AND DISTANCE
- BOOK - LUCENT PUBLICATIONCHAPTER - HEIGHT AND DISTANCEEXERCISE - EXERCISE-12B14videos
HEIGHT AND DISTANCE
- BOOK - LUCENT PUBLICATIONCHAPTER - GRAPHICAL SOLUTION OF LINEAR EQUATION EXERCISE - EXERCISE-3B8videos
GRAPHICAL SOLUTION OF LINEAR EQUATION
- BOOK - LUCENT PUBLICATIONCHAPTER - INDICES AND SURDS EXERCISE - Exercise - 2B14videos
INDICES AND SURDS
Similar Questions
Knowledge Check
- Question 1 - Select One
Each angle of a regular hexagon is:
A60∘B120∘C90∘Dnone of these - Question 2 - Select One
Each angle of a regular hexagon is:
A60∘B120∘C90∘Dnone of these - Question 3 - Select One or More
Each side of an equilateral triangle subtends an angle of 60∘ at the top of a tower h m high located at the centre of the triangle. If a is the length of each side of the triangle, then
A3a2=2h2B2a2=2h2Ca2=3h2D3a2=h2
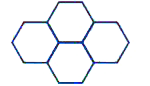
Four regular hexagons are drawn so as to form the design as shown in Fig. 6.11. If the perimeter of the design is 28cm, find the length of each side of the hexagon.
 View Solution
View SolutionEach side of a square ABCD subtends an angle of 60∘ at the top of a tower of height h standing at the centre of a square. If a be the length of the side of the square, then
View SolutionEach side of a square substends an angle of 60∘ at the top of a tower h metres h metres high standing in the centre of the square . If a is the length of each side of the square , then
View SolutionEach side of a square subtends an angle of 60∘ at the tip of a tower of height h metres standing at the centre of the square. If I is the length of each side of the square, then what is h2 equal to ?
View SolutionEach side of an equilateral triangle subtends an angle of 60∘ at the top of a tower h meters high located at the centre of the triangle. If a meters is the length of each side of the triangle, then
View Solution

